TL;DR
I tend to agree with the answer by @GyroGearloose that the difference in the temperatures of ice is the crucial factor here. In the glacier situation the the ice surface and the surface of one's hand are both at the same temperature, so nearly nothing happens during a brief touch. If holding a piece of ice for a while, it begins to melt, as the hand is being constantly warmed up and loses its heat to the air and to the piece of ice that it holds.
The ice coming out from freezer is at the temperature lower than the freezing point (-18C and possibly lower.) Hence the characteristic sticky feeling when holding it - the moisture on the hand freezes when in contact with ice, and the ice begins to melt only after being out of freezer for come time.
A similar effect can be observed, if touching the glacier ice with a warmer part of one's body, e.g., with one's tongue (I suggest doing it as a though experiment, rather than actually trying it - in freezing weather one risks not being able to separate one's tongue afterwards with possible long-lasting physical damage.)
Update
In response to the comments it is worth stressing that the temperature of human body is not homogeneous. It is true that warm-blooded animals, like humans, need to sustain temperature in a certain range, to remain alive. However, the optimal temperatures depend on the tissue, and lower for the parts of the body exposed to exterior. Furthermore, the outer layers of skin are essentially dead cells, which are warmed only via contact with the still alive cells inside, but cooled by their contact with exterior.
To illustrate these points:
Distribution of temperatures in human body image source

Optimal temperature for different parts
The fact that temperature varies around the body is taken into account in medical settings, where different temperature ranges are considered as "normal" for different parts, and certain measures are considered more reliable (i.e., subject to less fluctuations) - like rectal and oral temperature, vs. less reliable armpit:
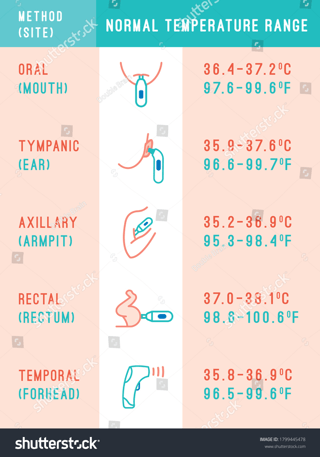
(image source)
Skin in contact with cold air
Outer skin temperature approaches that of the surrounding environment:
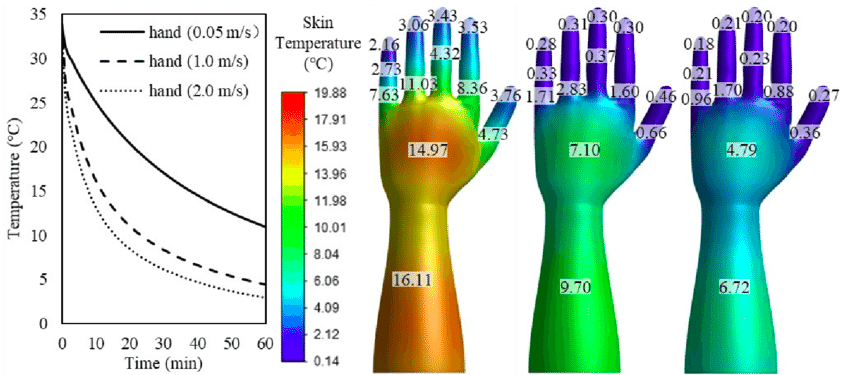
(image source)
Heat capacity and size
Whether touching ice may cause residual water on fingers to freeze or whether the ice melts from the contact with fingers depends on the temperatures (of the ice and the fingers) and the amount of ice. Let $c_{ice}, c_{water}$ be the specific heat capacities of ice and water, $m_{ice}, m_{water}$ are the amounts of ice and water on the fingers, $T_{ice}, T_{water}$ their initial temperatures, and $\lambda$ the latent heat of ice.
We expect that the ice warms up from the contact with fingers, while the water on fingers cools down (in principle, we have to take into account cooling down of the skin, but the outer skin layers have 70% water content and heat capacity close to that of water - e.g., see here). If the ice didn't melt, i.e., the equilibrium temperature is below the freezing point, it is determined by:
$$m_{ice}c_{ice}(T-T_{ice})=m_{water}c_{water}(T_{water}-T) + m_{water}\lambda$$
that is
$$
T = \frac{m_{ice}c_{ice}T_{ice} + m_{water}c_{water}T_{water}+m_{water}\lambda}
{m_{ice}c_{ice} + m_{water}c_{water}}
$$
If the temperatures are measured in respect to the freezing point (it is the default for the Centigrade scale, otherwise simply replace all temperatures by $T-T_{freezing}$), then the first term is negative, and the result is negative, provided that $m_{ice}T_{ice}$ has big enough magnitude - i.e., when we deal with a large quantity of ice or when it is very cold (or both.)
If instead the ice melted, the condition is:
$$m_{ice}c_{ice}(T-T_{ice})+ m_{ice}\lambda=m_{water}c_{water}(T_{water}-T)$$
that is
$$
T = \frac{m_{ice}c_{ice}T_{ice} + m_{water}c_{water}T_{water}-m_{ice}\lambda}
{m_{ice}c_{ice} + m_{water}c_{water}}
$$
Assuring that this temperature is above freezing point requires higher temperature of fingers.
While estimating the exact amount of water on fingers, its temperature and how fast the heat propagates may be tricky, we can base our reasoning on the common experience that:
- ice cube does not melt immediately when taken out of the freezer: the amount of heat required to melt it is substantially larger than the amount of heat in the fingers. It eventually melts because the heat is resupplied by the rest of the body, which tries to prevent temperature loss
- ice cube taken out of a freezer is sticky, while the ice cube that has been exposed to the ambient temperature above the freezing point is not sticky - its surface has temperature that is not very different from that of our fingers, and the contact does not result in immediate freezing or melting of water.
- likewise, at ambient temperatures below the freezing point, no sticking is observed - this time because the outer surface of fingers is cold.
Sensation of cold
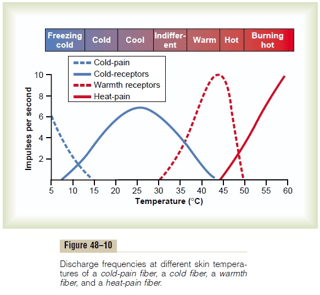
The sensation of cold is not determined by a gradient of temperatures between the temperature inside and outside the, but rather by the firing rate of thermoreceptors, a particular type of sensory neurons. There are different kinds of thermoreceptors, active at different temperatures, and with temperature-dependent sensitivity:

(image source) Holding an ice cube coming out of freezer (-18C or lower) triggers cold-pain receptors, which are normally inactive at comfortable room temperature (about 20C). This also generates higher contrast with change in firing of cold receptors. Finally, this generates contrast with the other receptors in the body, which are far from the area in contact with ice (there are no thermoreceptors in the outer layers of skin, they are buried inside (see, e.g., this article.)
When touching glacier at lower ambient temperatures, the contrast between the firing rates of various receptors and the receptors immediately affected is not that great. Furthermore, if the ambient temperature is above -15C, the cold-pain receptors are not involved at all - whereas at lower temperatures, their signal is no different from the signals sent by the similar receptors in the rest of the body.
Related
Why does ice become sticky the colder it gets?
Why does the tongue stick to a metal pole in the winter?





