Number of integrals of motion

Question

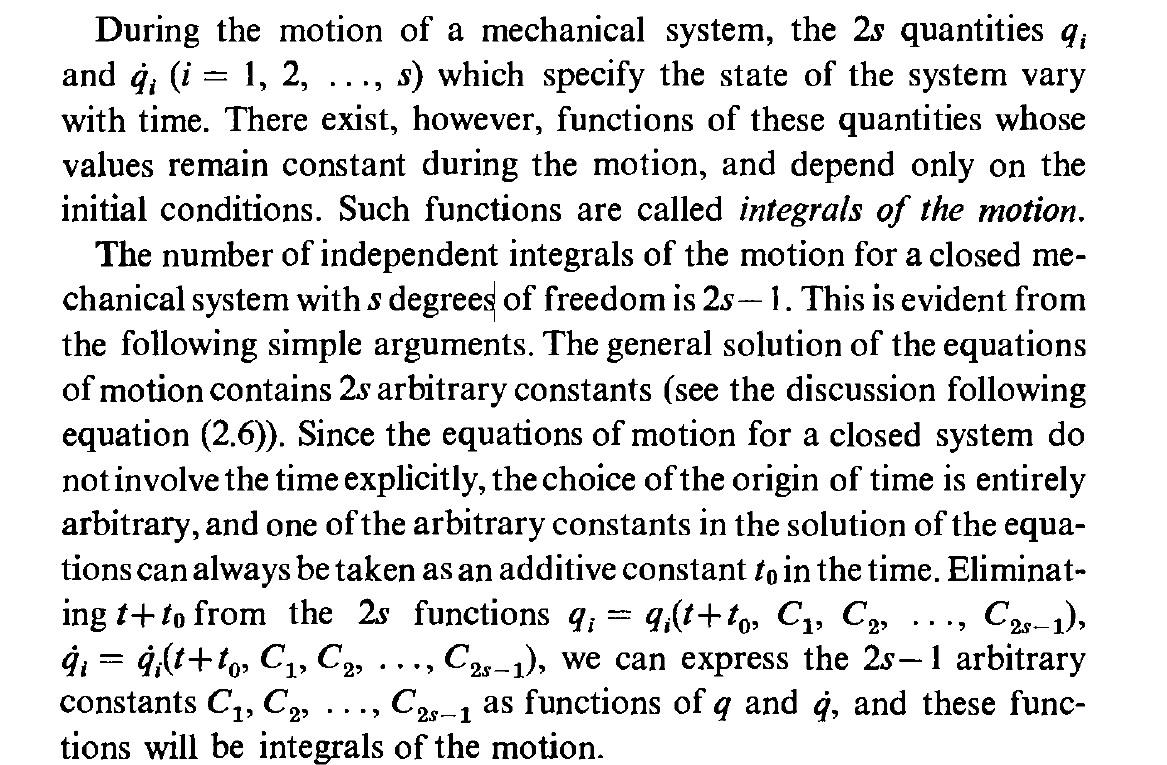
In Landau-Lifshitz Classical mechanics textbook, it is said that there are generally $2s-1$ integrals of motion where $s$ is the number of degrees of freedom. Why is that? I couldn't find anywhere an answer.

Answer 1

Every autonomous dynamical system arising from mechanics can be described as a first-order ODE on a $2n$-dimensional manifold $M$ (the spacetime of kinetic states of Lagrangian mechanics with local coordinates $q,\dot{q}$ or the space of phases of Hamiltonian mechanics with coordinates $q,p$). Every state $s\in M$ determines a unique solution of the ODE describing the motion with that initial condition at $t=0$. As a consequence, the motions are the integral lines of a vector field $Z$ defined on $M$.

In other words, the equations are actually of the form $$\frac{ds}{dt} = Z(s(t))\:.$$ For instance, in Hamiltonian formulation, the right-hand side is the Hamiltonian vector field generated by a given Hamiltonian. $$\frac{dq^k}{dt} = \frac{\partial H}{\partial p_k}\:,\quad \frac{dp_k}{dt} = -\frac{\partial H}{\partial q^k}\:, \quad k=1,\ldots, n$$ so that $$Z = \sum_{k=1}^n\frac{\partial H}{\partial p_k} \frac{\partial}{\partial q^k}- \sum_{k=1}^n\frac{\partial H}{\partial q^k} \frac{\partial}{\partial p_k}\:.$$

In the Lagrangian case, the existence of $Z$ relies on the well-known fact that the equation of Euler-Lagrange can be written in normal form: $$Z = \sum_{k=1}^n A^k \frac{\partial}{\partial \dot{q}^k} + \sum_{k=1}^n\dot{q}^k\frac{\partial}{\partial q^k}$$ in order that $$\frac{dq^k}{dt}= \dot{q}^k\:, \quad \frac{d\dot{q}^k}{dt}= A^k(q,\dot{q})\:,\quad k=1,\ldots, n $$ are the E.L. equations, for some Lagrangian $L=L(q,\dot{q})$, written in normal form.

Unless $Z(s_0)=0$, there is a neighborhood $U$ of every $s_0\in M$ such that $Z(s)\neq 0$ for $s\in U$. As is well-known from differential geometry (I am assuming that $M$ and the vector field $Z$ are at least $C^1$) and as is easy to prove, it is always possible to equip a smaller neighborhood $V\subset U$ of $s_0$ with a system of coordinates $t,x^2,\ldots, x^{2n}$ constructed like this. $t$ is the parameter of the integral curves of $Z$ computed starting from a $2n-1$ submanifold transverse to $Z$ where $t=0$ and the remaining coordinates are coordinates on that submanifold.

So let us denote by $y^1,\ldots, y^n$ the constructed local coordinates around $s_0$.

In these coordinates, the solution of the motion with initial conditions $y^1_0,\cdots, y^{2n}_0$ at time $0$ have a trivial expression: $$y^1(t) = y^1_0+t\:,\quad y^{k}(t)= y_0^k\:, \quad k=2,\ldots, 2n.$$ In other words, the coordinates $y^k$ remain constant along the motion if $k=2,\ldots, 2n$.

These constants of motion are also functionally independent as they are coordinates of a local chart on $M$.

It should be clear that we cannot have more than $2n-1$ functionally independent integrals of motion. If they existed they would define a coordinate system around each state. So, when their values are given, then the state is fixed and no evolution is possible. Around a state where $Z\neq 0$ this is not possible.

ADDENDUM. I prove here the existence of the said coordinate system in the autonomous case.

Let us suppose that $Z(s_0) \neq 0$. Choose a local chart in $M$ around $s_0$ equipped with coordinates $x^1,\ldots, x^{2n}$. Exploiting a suitable bijective linear transformation, we can always change the coordinate system in order that, exactly at $s=s_0$, $$Z(s_0) = c \frac{\partial}{\partial x^1}|_{s_0}\:, \quad c>0\:.$$ By continuity, the component of $Z$ along $x^1$ cannot vanish in a neighborhood of $s_0$. Therefore, the $2n-1$ submanifold $C \subset M$ locally described by $x^1=0$ is transverse to $Z$ around $s_0$. I stress that $C$ is covered by local coordinates $x^2,\ldots, x^{2n}$ as a consequence.

Since $Z$ is $C^1$, it admits a $C^1$ local flow $$\phi^{(Z)}: A \to M$$ where $A \subset \mathbb{R}\times M$ is an open set containing the points $(0,s)$. By definition of flow, the jointly $C^1$ map $$A\ni (t,s) \mapsto \phi_t(s)\in M$$ is the solution of the differential equation $$\frac{dx}{dt}= Z(x(t))$$ with initial condition $s$ and evaluated tat the value $t$ of the parameter: $$\phi_t(s) = x(t; s)\:.$$

Let us consider the $C^1$ map $$I \times C \ni (t,s)\mapsto \phi_t(s) \in M$$ where $I \subset (-\infty,+\infty)$ is a sufficiently small interval containing the origin such that the right-hand side is well defined when $s\in C$. As before $C$ is the $2n-1$ dimensional submanifold $C$ transverse to $Z$. If necessary, we take $C$ sufficiently narrowed around $s_0$. Notice that $I$ exists because $A$ is open.

It is easy to prove that, working in local coordinates $x^1,\ldots, x^{2n}$, the Jacobian matrix of the above map has non zero determinant exactly at $(0,s_0)$.

(That is because the Jacobian matrix evaluated at that point has the first row made of the components of $Z$ in coordinates $x^1,\ldots, x^{2n}$ and the remaining rows made of the rows of the identity matrix $(2n-1)\times (2n-1)$. The determinant is therefore the first component of $Z$ at $s_0$, which does not vanish by hypothesis.)

Applying the theorem of the inverse function, we have that, in a neighborhood of $(0,s_0)$, the local coordinates $(t,x^{2}, \ldots, x^{2n})$ define an admissible $C^1$ local chart on $M$.

The found coordinates around $s_0$ denoted by $y^1,\ldots, y^n$ are such that the solution of the motion with initial conditions $y^1_0,\cdots, y^{2n}_0$ at time $0$ have a trivial expression: $$y^1(t) = y^1_0+t\:,\quad y^{k}(t)= y_0^k\:, \quad k=2,\ldots, 2n.$$ As said, the coordinates $y^k$ remain constant along the motion if $k=2,\ldots, 2n$. As said, we have $2n-1$ functions defined on $M$ around $s_0$ which are constants of motion. These functions are also functionally independent (their Jacobian matrix has maximal rank), just because they are local coordinates.

Answer 2

In Landau's mechanics, one can read in chap 6 about the energy:

What is it you do not understand?