Free Body Diagram for a Body on a Smooth Inclined Plane [duplicate]

Question

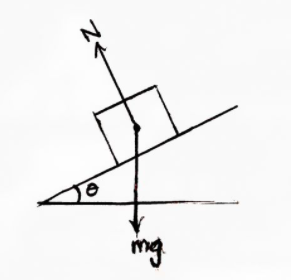
Consider a body of mass $m$ on a smooth inclined plane with the normal force and weight labelled as shown:

Now, usually when the forces are broken into its components, we get an FBD like this:

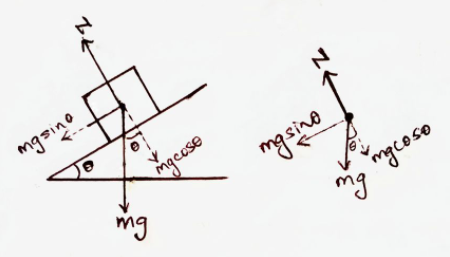
From this we can obtain the equation:

$$ \Sigma F_\perp = 0\quad \implies\quad N = mg\cos(\theta) \tag1$$

However, I tried resolving the forces along the x and y axes and obtained a diagram like this:

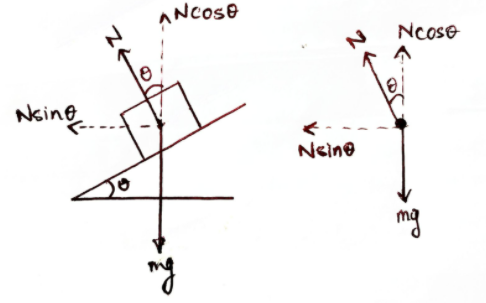
But this time I got a different equation: $$ N\cos(\theta) = mg \tag2$$

Clearly, equation (1) and (2) aren't the same. So what am I missing here?

Answer 1

If instead of decomposing into perpendicular and parallel force components, you use the 'regular' $x$ and $y$ axis as you did to get Eq. (2), then you will have a non-zero acceleration in both $x$ and $y$ directions. So, what you really should end up with for Eq. (2) would be $$ma_y=mg-N\cos\theta\tag2$$ because the forces are not balanced. Therein lies the reason why we decompose into parallel and perpendicular components -- because the block will only slide parallel to the incline, so we can nicely conclude that $\Sigma F_\perp=0$. However, in the usual $xy$ coordinate system, there will be an acceleration in both $x$ and $y$ directions. So you can't just assume one of them is zero.

Answer 2

Nothing wrong with you analysis so far but you now need to consider what you are going to do with the horizontal $N\sin \theta$ force noting that the acceleration is going to be parallel to the slope.