I am confused about the current and voltage. My intuitive example would be that of a pipe of say water. The diameter of the pipe determines the amount of water flowing per second but the pressure is comparable to voltage. Am I right?
And what is the difference between voltage source and current source? In what class our electric sockets on the wall do fall?
A voltage source is assumed to deliver energy with a specific terminal voltage which does not depend upon the current from the source. A current source on the other hand is assumed to deliver energy with a specified current through the terminals.
Both current and voltage sources are ideal. In practice, we represent a real voltage source as an ideal voltage source in series with a resistance and a real current source as an ideal current source in parallel with a resistance.
All the best.
Your analogy with water flow through a pipe is correct. In that analogy a voltage source corresponds to a pump that generates a specific pressure, and a current source corresponds to a pump that generates a specific water displacement (volume per second).
Just to put it slightly differently, in high school physics more than fifty years ago we learned that in the water analogy the voltage corresponds to pressure which corresponds to the height of the head in the water system. Of course this corresponds to DC and our wall sockets are AC. But the voltage is fixed, so they are voltage sources, just as our water pipes are fixed pressure sources. We regulate water flow by turning valves (faucets) to change the size of the opening. We regulate current flow, say in an electric stove or a light with a dimmer switch by changing the resistance. In each case the control acts like a choke point in the piping system.
A voltage source provides a constant voltage but variable current and a current source provides constant current and variable current at given load.
If you think of it from practical side, it's
Voltage source = stabilized (fixed) voltage. That is what we usually expect to see as a power source.
Current source = stabilized (fixed) current. This kind of source will increase/decrease its output voltage to match required current. This is used to power non-linear devices like LEDs, lasers, gas discharge tubes.
It is perhaps easier to consider a battery as the voltage source with no internal resistance.
Within the battery a chemical reaction maintains a constant potential difference $V_b$ across the terminals of the voltage source.
Now connect another voltage source $V_s$ and a resistor $R$ across the terminals of the battery with the negative terminals of the battery and the voltage source connected together then a current $I_b$ flows in the circuit.
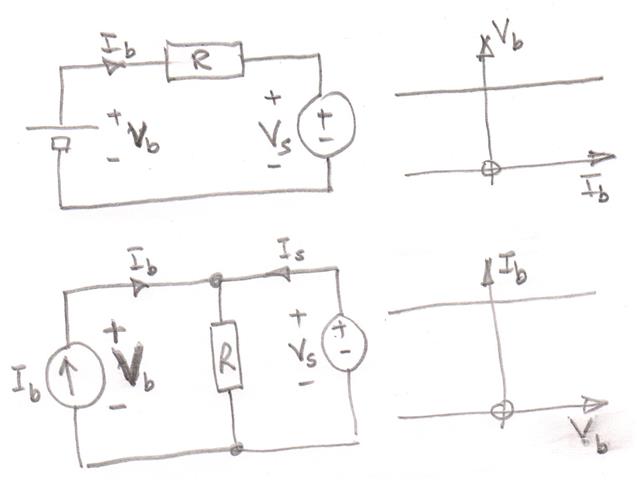
Applying Kirchhoff's voltage law gives
$V_b - V_s = I_b R$
If $V_b > V_s$ then the current $I_b$ flows out of the positive terminal of the battery.
Changing the value of the resistance of the resistor does not change the potential difference across the terminals of the battery.
If $V_b<V_s$ then current flows into the positive terminal of the battery but the voltage across the terminals does not change even when the resistance $R$ and hence the current $I$ changes.
You can interpret this as the battery being recharged with electrical energy being pumped into the battery and reversing the chemical process within the battery.
So electrical energy is flowing into the battery.
Now a similar analysis for a current source yields the fact that the potential difference across a current source has to follow the potential difference imposed on it by an external circuit $V_b = V_s$ whilst still maintaining a constant current.
The interpretation of this effect is a little more difficult to understand but ultimately means that when the terminal of the current source out of which the current flows is at a negative potential relative to the other terminal of the current source (the positive and negative signs in the diagram are reversed) electrical energy is flowing into the current source and perhaps yet again is recharging a battery which is part of the current source circuit?
The voltage source is like a pump which creates specific pressure under which the water in the pipe flow . As,the diameter increase large amount of water can be transmitted with maximum pressure likewise in large diameter wire large amount of current flows with maximum voltage.The electric socket has a plug hole arrangement used to transfer electrical energy from one circuit to other .
