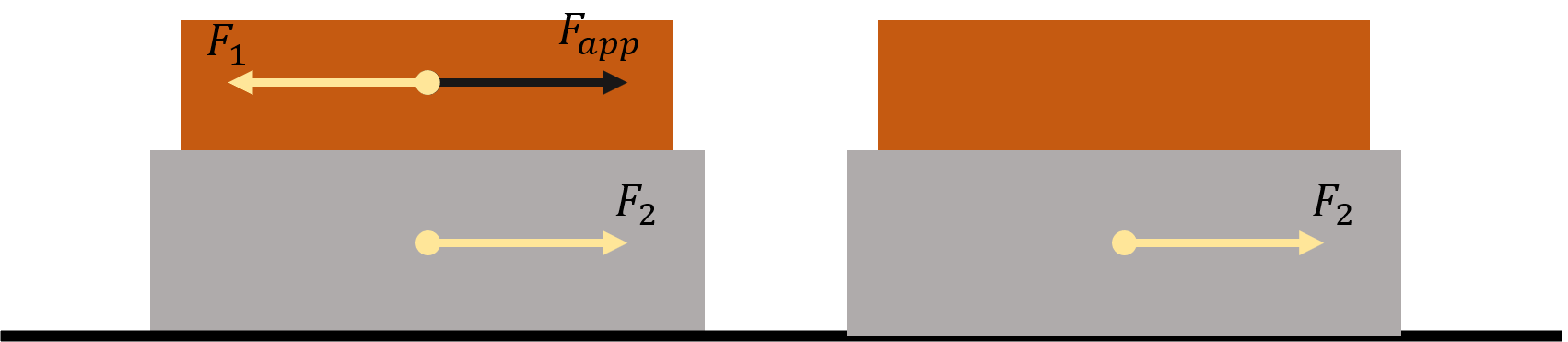Why doesn't the static frictional force between two blocks, placed on top of one another, cancel out the applied force?

Question

After having attended a specific mechanics course I've struggled with a certain problem, which is related to static friction. First of all, if some force is applied to a block (the source of the applied force is not of importance in this scenario), that's placed on top of another block, it's going to accelerate in case there's no static friction to cancel the applied force. However, as the left picture below suggests, there's a static frictional force between the two blocks, and for simplicity no frictional force between the lower block and the ground. Now the static friction will always be equal in magnitude to the applied force but in the opposite direction, at least to a certain level. As can be seen, $F_1$ compensates for $F_{app}$, at the same time, Newton's third law states that each applied force has an equal and opposite force which acts on the other object. Hence, the static frictional force $F_2$ is applied on the lower block, so I would say that the net force on the above block will become 0N and the lower block will experience a net force of $F_2$. As a result the above block will remain stationary and the lower block is going to accelerate, but this final result doesn't seem to make sense to me: the upper block remaining still why the lower block is going to accelerate. I must miss some crucial point about the static frictional force, but what? Thanks.

Answer 1

The key mistake you made is in the following statement:

As can be seen, $F_1$ compensates for $F_{app}$, at the same time, Newton's third law states that each applied force has an equal and opposite force which acts on the other object. Hence, the static frictional force $F_2$ is applied on the lower block, so I would say that the net force on the above block will become 0N and the lower block will experience a net force of $F_2$

The friction force $F_1$ does not cancel out $F_{applied}$ for a net force of zero on the upper block. Assuming the maximum possible static friction force is not exceeded, both blocks will stick together and have the same acceleration with respect to the ground.

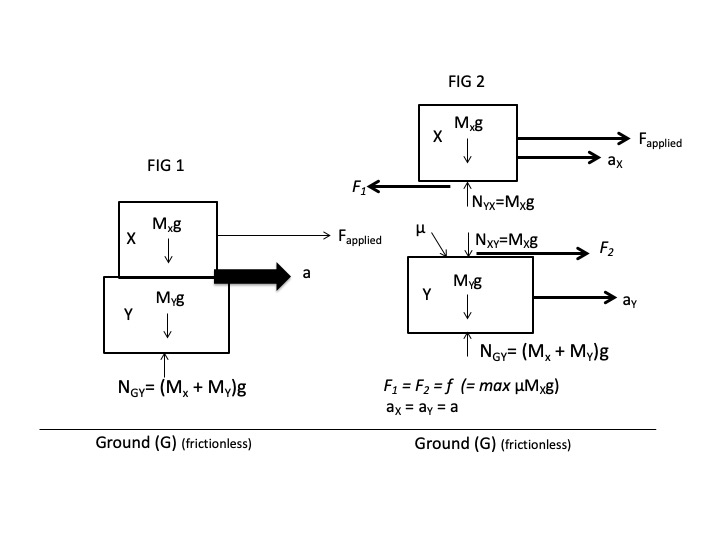
As my undergraduate mechanics professor used to say over and over again, "Draw a free body diagram!!!". A free body diagram (FBD) should show all the external forces acting on the body.

Fig 1 below is a FBD of the combined top and bottom blocks. It assumes (1) no friction between the lower block Y and the ground and (2) that the maximum possible static friction force between the blocks is not exceeded so that the blocks stick together. Based on the FBD the only net external force acting on the combined blocks is $F_{applied}$. Therefore, applying Newton's second the acceleration $a$ of the combined blocks with respect to the ground is

$$a=\frac{F_{applied}}{M_{X}+M_{Y}}$$

Fig 2 are FBDs for the individual blocks. For each block the equal and opposite friction force that each exerts upon the other per Newton's third law acts as an external force. Since the blocks move together, each block has the same acceleration $a$ as the combined blocks of Fig 1.

From the FBD on the upper block applying Newton's second law we have

$$F_{Xnet}=F_{applied}-f=M_{X}a=\frac{M_{X}}{M_{X}+M_{Y}}F_{applied}$$

Solving the equation for the friction force $f$

$$f=\frac{M_Y}{M_{X}+M_Y}F_{applied}$$

From the FBD on the lower block we see that the only external force acting on the block is the friction force of the upper block. Therefore applying Newton's second law to the lower block

$$F_{Ynet}=f=M_{Y}a=\frac{M_{Y}}{M_{X}+M_{Y}}F_{applied}$$

The values of the static friction force is the same, as it should be.

But does that mean my sketched situation is physically impossible? So if 𝐹𝑎𝑝𝑝𝑙𝑖𝑒𝑑=𝑓𝑚𝑎𝑥−𝑠𝑡𝑎𝑡𝑖𝑐 I would say that 𝐹𝑋,𝑛𝑒𝑡=0 and 𝐹𝑌,𝑛𝑒𝑡=𝐹𝑚𝑎𝑥−𝑠𝑡𝑎𝑡𝑖𝑐 because of Newton's 3rd law.

I think I now understand the root cause of your issue. The static friction force is a variable force that matches the applied force up to the maximum static friction force. They do in fact cancel each other but only with respect to the acceleration of the top block relative to the bottom block, but not with respect to the acceleration of the top block relative to the ground.

Up to and until the applied force equals the maximum static friction force when slipping is impending, there is no slippage and the acceleration of the top block is the same as the bottom. But once slipping occurs, the friction force transitions from static to kinetic friction and the upper block moves relative to the lower block. The coefficient of kinetic friction (and thus the kinetic friction force) is generally less than that of the static friction force. At that point the applied force will exceed the kinetic friction force for a net force that causes the upper block to accelerate with respect to the lower block, as well as with respect to the ground.

The acceleration of the lower block with respect to the ground will be dictated by the kinetic friction force, which is generally considered constant regardless of the applied force.

Hope this helps.

Answer 2

In this case the static friction is not equal to the force F. You can conclude this only when the body has zero acceleration. As you have no friction at the bottom, both blocks will start to move with some acceleration. You just have to draw free body diagram for each body and apply Newton's second law for each body to find the static friction and the common acceleration. You can also find at what value of F the two bodies start to move relative to each other and the friction becomes kinetic.

Answer 3

Now the static friction will always be equal in magnitude to the applied force but in the opposite direction, at least to a certain level.

This is where you're running into trouble. There's no particular requirement that the static frictional force be any particular value, so long as it doesn't exceed $\mu_s$ times the normal force. Your assumption is true if the object is at rest; but in this case, the upper block is accelerating, and so the net force on it will not be zero.

A better way to think about static friction problems is to assume that the static friction is less than or equal to its critical value, and then see what constraints this imposes on the situation. If the constraints are violated, then we know that there will be slippage.

In this case, we have from the two free-body diagrams $$ F_\text{app} - F_\text{fr} = m_1 a \qquad \text{ and } \qquad F_\text{fr} = m_2 a, $$ where $m_1$ and $m_2$ are the masses of the top & bottom blocks, respectively. Implicitly, we have assumed that the accelerations of the two blocks are equal. We also know from looking at the vertical forces that the normal force exerted between the two blocks is $m_1 g$, so $F_\text{fr} \leq \mu_s m_1 g$. This inequality lets us (in principle) place bounds on the value of $a$ and/or $F_\text{app}$ if we want the blocks to move without slipping; intuitively, it should make some sense that such bounds exist. But more importantly for your question, you can see that $F_\text{fr} \neq F_\text{app}$ unless $m_1 a = 0$.

Answer 4

You are correct. If your applied force is equal to maximum value of static friction, then lower block will accelerate while the upper block will remain stationary.Thus slipping will occur.

The reason you might feel strange is because in real life the friction between lower block and ground prevent this from happening.