For a pendulum which has a light string to hang a bob, I know that when the bob swing to the leftmost or rightmost end, the velocity of the bob is zero and the acceleration should be maximized. But if you look at the formula of the angular acceleration $a = v^2/r$, but when $v$ is zero, acceleration will be zero too. So why in the text they said that the acceleration at that point is maximum?
$\begingroup$
$\endgroup$
4
asked Mar 28, 2013 at 14:12
-
8$\begingroup$ $a=v^2/r$ is the radial acceleration, not the tangential acceleration. $\endgroup$– Mike DunlaveyCommented Mar 28, 2013 at 14:52
-
$\begingroup$ Angular acceleration is $v^2/r$ only for uniform circular motion when $v$ is constant (and, acceleration exists due to change in direction). $\endgroup$– Earth is a SpoonCommented Mar 28, 2013 at 16:13
-
1$\begingroup$ @SachinShekhar Mike is correct. The acceleration can be resolved into two components in polar coordinates. One is radial and the other is tangential. The radial component has magnitude $v^2/r.$ $\endgroup$– Pricklebush TickletushCommented Mar 28, 2013 at 17:01
-
$\begingroup$ @Alec I am not opposing Mike. I am just explaining to OP what elementary school textbooks mean. My comment and Mike's comment are basically same. $\endgroup$– Earth is a SpoonCommented Mar 29, 2013 at 7:30
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
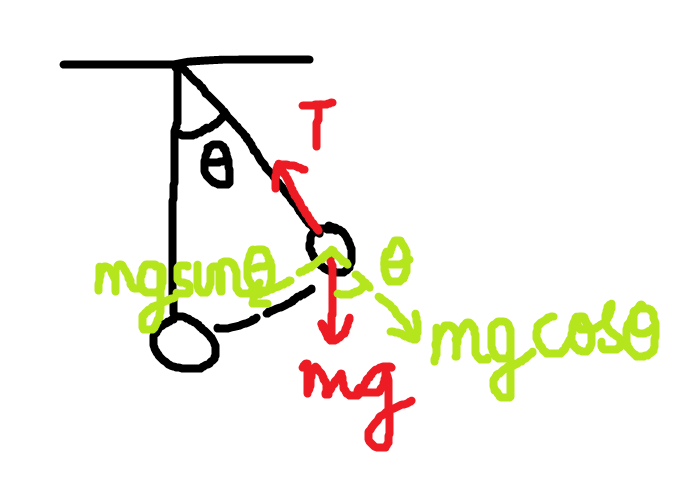
When the pendulum swings, at the time when angle is $\theta$, I have listed the forces. In all there are two forces $T$(tension) and $mg$(weight)[shown in red]
You can resolve $mg$ into components along the motion and perpendicular to the motion[shown in green].
The string is inextensible, so net forces in the direction of string is $0$, so $T=mg\cos\theta$
The unbalanced force is $mg\sin\theta$ which causes the motion of pendulum. At the leftmost or rightmost point, $\theta$ is maximum. Hence $\sin\theta$ is maximum(it doesn't go up the point of suspension), so net acceleration in the direction of motion is $(g\sin\theta)_{max}$. The book probably says this.
dmckee --- ex-moderator kitten
86.7k9 gold badges175 silver badges346 bronze badges
answered Mar 28, 2013 at 15:27
$\begingroup$
$\endgroup$
WE know that the equation of motion of a simple pendulum can be written as y= a sin(wt). y= displacement of the pendulum at time "t" from the mean position a= amplitude of oscillation w= angular frequency of oscillation remember in this case y=0, when time t=0. now (dy/dt)= aw cos(wt)= velocity =v again (dv/dt) = -aw^2 sin(wt)= acceleration=f If T= time period then w= (2 pi/T)
now at time t= T/4, v=0 but at the same instant f= -aw^2= f(max)
$\begingroup$
$\endgroup$
4
I would say that the text is right, as the force on ot always equals mg and T at an angle. So, for the acceleration part, you can be SURE that T will not cancel mg, as there is an angle between them and the magnitude therefore has to be less than mg. Moreover, since force is not zero, and this is the NET force, so there must be a net acceleration.
Furthermore, it is maximum, as T tends towards mg as theta reduces as the bob moves down. So, net force is zero for an instant when the bob is at the mean position. So, we see that theta is directly proportional to acceleration, and this final statement should cover up this horribly long answer! :)
answered Mar 28, 2013 at 14:29
-
-
1$\begingroup$ Watch the simulation there. $\endgroup$ Commented Mar 28, 2013 at 14:35
-
2$\begingroup$ "T tends towards mg as theta reduces as the bob moves down" - $T$ does not become $mg$ at the bottom of the swing unless the speed of the bob is zero there. $\endgroup$ Commented Mar 28, 2013 at 15:19
-
$\begingroup$ Well, I believe, the main answer is not mg becoming or tending to zero, and I also believe that the main concern of the user has been addressed, if there's any inaccuracy wi regards to that, kindly bring it to my attention $\endgroup$ Commented Mar 28, 2013 at 15:54