How does relaxing counterfactual definiteness allows for CHSH inequality violation?

Question

Scenario, I want to play a game with a group of students as a teacher, I ask two teachers to help.

The students are from the central classroom, going to room A and room B where the teachers there asks the students question 1 or 2. So A1, A2 are the possible questions to ask students who are in room A, B1, B2 are the possible questions for students in room B.

The answers the students give, I use lowercase, a1, a2, b1, b2, it can only be either 1 or -1. The goal of the student is to try to violate CHSH inequality, we introduce to the |S|= | average of (a1b1) + average of (a1b2) + average of (a2b1)− average of (a2b2)| and violation means getting |S| to be more than 2.

We impose locality by no communication between room A and room B. We impose counterfactual definiteness by asking the students not to randomly give the answer on the spot, but have it ready for all possible questions they are asked (only 2 possible questions for each student). They are free to decide the correlation (hidden variables), eg. they synchronise their watches, and answer 1 if the minute hand is pointing to even number, and answer -1 if the minute hand is pointing to odd number.
We impose freedom of choice of the experimenter, by allowing the teachers to choose whatever questions they wish to ask and the students do not know which of the 2 questions in each of the 2 rooms will be asked.

I can see easily how relaxing locality (to allow them phones), or freedom (to give them beforehand the questions the teachers in both rooms will ask) allows them to get S to become 4.

But I cannot see how only relaxing counterfactual definiteness (allowing them to guess the answer on the spot), but retaining locality and freedom allows the students to violate CHSH.

So for each pair of students going out, the one going into room A only have to answer 1, whatever the question is. The one going to the room B has to answer 1, except if they got the question B2 and if they know that the question A2 is going to be asked of student in room A The main difficulty is, how would student B know what question student A got? They are too far apart, communication is not allowed. They cannot know the exact order questions they are going to get beforehand.

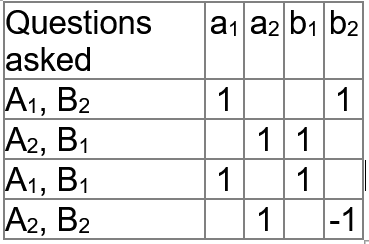
Say if students who go into room B decide to go for random answering if they got the question B2, on the faint hope that enough of the answer -1 will coincide with the question A2. We expect 50% of it will, and 50% of it will not.

So let’s look at the statistics.

Average of (a1b1) = 1

Average of (a2b1) = 1

Average of (a1b2) = 0

Average of (a2b2) = 0

S=2

Average of (a1b2) and Average of (a2b2) are both zero because while a always are 1, b2 take turns to alternate between 1 and -1, so it averages out to zero. Mere allowing for randomisation and denying counterfactual definiteness no longer works

Anything I am missing? Or is it not suitable to cast this as a game to be played by human students in class?

Answer 1

You cannot have counterfactual definiteness when violating the CHSH inequality.

It does not mean that not having counterfactual definiteness guarantees a violation of the inequality. Randomly guessing answers is an obvious example of this.

It applies to the other considerations too. You can let the students violate locality with a phone but they still have to use this in a way that helps them win the game i.e. by discussing the answers to the questions and not something else.

Answer 2

I do not really see how "counterfactual definiteness" corresponds to the students giving random answers on the spot. After all, the violation of the CHSH-inequality corresponds to a correlation that is larger than classicaly allowed, in particular larger than random answers could achieve.

But I have to admit that I may not have understood counterfactual definiteness and also did not hear about it in any prior discussion of Bell inequalities. Wikipedia states, Counterfactual definiteness is

the ability to speak "meaningfully" of the definiteness of the results of measurements that have not been performed.

That sounds like the students will somehow pick the right answers that do not fit into a hidden-variable model.

In my understanding of the implications of the CHSH-inequality, the assumptions are

1. Locality (as you stated).
2. Freedom of Choice / Future-Input-Independence / Statistical Independence (also as you stated).
3. Reality (instead of counterfactual definiteness).

In quantum mechanics, locality can only be achieved by giving up reality if one, in addition, looks at the Heisenberg picture, since in the Schroedinger picture (if we look at the time-evolution of states) any entangled state will be non-local in space: $$ |\psi_1(0,0)\rangle \otimes |\psi_2(0,0)\rangle \to |\psi_1(t,x)\rangle \otimes |\psi_2(t,y)\rangle \neq 0 $$ even if $x$ and $y$ are spacelike separated.

In the Heisenberg picture, we remain with $|\psi_1(0,0)\rangle \otimes |\psi_2(0,0)\rangle$ at time 0 and the observables $T_1 \& T_2$ are time-evolved to $T_1(t) \& T_2(t)$. This gives up reality (in the sense of your Scenario) that we only send the students into a room to chat, then only look at the teachers which will later receive answers to their questions. The students are just "part of the world" which acts on our observables in some way.

I hope that gives you at least one way of understanding the three assumptions used to derive the CHSH inequality. I am not convinced by the notion of "counterfactual definiteness". But if someone can explain it to me I am happy to hear.