Can One-Way Speed of Light be Instantaneous?

Question

I recently watched this video by Veritasium where he talks about the One Way Speed of Light and talks about the limiting case where in one direction the speed of light is $c/2$ while it's instantaneous in the other. He also says this is perfectly fine according to our Physics theories. He also points at Einstein's assumption in his famous 1905 paper where he assumes that the speed of light is same in all directions.

This made me ask this question is taking the speed of light same in all directions an axiom of some sort?

As I've often read no information can be sent at more than the speed of light but here one-way taking the speed to infinite makes no difference.

So are all of our physics theories based on the assumption and what would happen if light turns out to be moving at different speeds in different direction? Will that enable transfer of information faster than the speed of light and is there any way for us knowing that the transfer happens faster than the speed of light?

The video takes a Earth Mars case where he says it isn't possible for us to every realize this discrepancy but is there a more general proof which says it isn't possible

Answer 1

This made me ask this question is taking the speed of light same in all directions an axiom of some sort?

Yes, although it is called a postulate rather than an axiom. This is Einstein's famous second postulate:

Any ray of light moves in the “stationary” system of co-ordinates with the determined velocity c, whether the ray be emitted by a stationary or by a moving body. Hence $${\rm velocity}=\frac{{\rm light\ path}}{{\rm time\ interval}} $$ where time interval is to be taken in the sense of the definition in § 1.

A. Einstein, 1905, "On the Electrodynamics of Moving Bodies" https://www.fourmilab.ch/etexts/einstein/specrel/www/

This postulate is simply assumed to be true and the consequences are explored in his paper. The subsequent verification of many of the rather strange consequences is then taken to be strong empirical support justifying the postulate. This is the heart of the scientific method.

So are all of our physics theories based on the assumption and what would happen if light turns out to be moving at different speeds in different direction? Will that enable transfer of information faster than the speed of light and is there any way for us knowing that the transfer happens faster than the speed of light?

Yes, all of our physics theories are based on this assumption, but the assumption itself is simply a convention. The nice thing about conventions is that there is no "wrong" or "right" convention. This specific convention is known as the Einstein synchronization convention, and it is what the second postulate above referred to by "time interval is to be taken in the sense of the definition in § 1". From the same paper in section 1:

Let a ray of light start at the “A time” $t_{\rm A}$from A towards B, let it at the “B time” $t_{\rm B}$ be reflected at B in the direction of A, and arrive again at A at the “A time” $t'_{\rm A}$.

In accordance with definition the two clocks synchronize if $$t_{\rm B}-t_{\rm A}=t'_{\rm A}-t_{\rm B}$$ A. Einstein, 1905, "On the Electrodynamics of Moving Bodies" https://www.fourmilab.ch/etexts/einstein/specrel/www/

If we define $\Delta t_A= t'_A-t_A$ then with a little rearranging this becomes $t_B=\frac{1}{2}(t_A+t'_A)=t_A+\frac{1}{2}\Delta t_A$. This is a convention about what it means to synchronize two clocks. But it is not the only possible convention. In fact, Reichenbach extensively studied an alternative convention where $t_B=t_A+ \epsilon \Delta t_A$ where $0 \le \epsilon \le 1$. Einstein's convention is recovered for $\epsilon = \frac{1}{2}$ and the Veritasium video seemed oddly excited about $\epsilon = 1$.

Note that the choice of Reichenbach's $\epsilon$ directly determines the one way speed of light, without changing the two way speed of light. For Einstein's convention the one way speed of light is isotropic and equal to the two way speed of light, and for any other value the one way speed of light is anisotropic but in a very specific way that is sometimes called "conspiratorial anisotropy". It is anisotropic, but in a way that does not affect any physical measurement. Instead this synchronization convention causes other things like anisotropic time dilation and even anisotropic stress-free torsion which conspire to hide the anisotropic one way speed of light from having any experimental effects.

This is important because it implies two things. First, there is no way to determine by experiment the true value, there simply is no true value, this is not a fact of nature but a description of our coordinate system's synchronization convention, nature doesn't care about it. Second, you are free to select any value of $\epsilon$ and no experiment will contradict you.

This means that $\epsilon=\frac{1}{2}$ is a convention, just like the charge on an electron being negative is a convention and just like the right-hand rule is a convention. No physical prediction would change if we changed any of those conventions. However, in the case of $\epsilon=\frac{1}{2}$ a lot of calculations and formulas become very messy if you use a different convention. Since there is no point in making things unnecessarily messy, it is a pretty strong convention.

Finally, regarding FTL information transfer. If we use $\epsilon \ne \frac{1}{2}$ then there is some direction where information can travel faster than $c$. However, since in that direction light also travels faster than $c$ the information still does not travel faster than light. It is important to remember that under the $\epsilon \ne \frac{1}{2}$ convention the quantity $c$ is no longer the one way speed of light, so faster than light and faster than $c$ are no longer equivalent.

Answer 2

The question is,

1. "can the one way speed of light be instantaneous?"

2. "is taking the speed of light same in all directions an axiom of some sort?"

3. "what would happen if light turns out to be moving at different speeds in different direction?" Will that enable transfer of information faster than the speed of light and is there any way for us knowing that the transfer happens faster than the speed of light?"

My answer will be different from some others posted here, but this is not owing to a disagreement about the mathematics, it is a disagreement about terminology and what constitutes clear communication.

On Earth we have different time zones. For example, France is one hour ahead of England. This means one could set off on a journey from France to England, departing at noon (12:00) (French time) and, after an hour of travel, arrive in England at noon (English time). Does this mean you have travelled at infinite speed? Of course not. Is it a wonderful and amazing insight into the physics of relativity that challenges our ordinary perceptions about time? I don't think so.

The effect discussed in the video mentioned in the question is precisely this effect.

I'll unpack it algebraically in the following, which I hope will make it clear that this is all there is to it. The physics is intermediate between special and general relativity (GR). It can all be treated using special relativity, but since coordinate transformations are involved (not just Lorentz transformations) it helps if one brings in a little GR as well.

First let's present the standard approach. This first part will be a little technical for some readers, but you will be able to get the main point about how the speed of light is defined.

In GR we assert that spacetime is a 4-dimensional space of a certain kind, called "pseudo-Riemannian manifold, with signature $(-1,1,1,1)$ or (equivalently) $(1,-1,-1,-1)$". This means that near any event there exists a coordinate system in which to calculate the interval $ds$ between neighbouring events one can use the following equation: $$ ds^2 = - A^2 dt^2 + dx^2 + dy^2 + dz^2 $$ where $A$ is a constant, and furthermore it is a universal constant because if the metric having this form appeared to have a different value of $A$ from one event to another, than one can rescale the coordinates to make it come out the same everywhere. Hence the constant $A$ earns a name, because it is a universal constant. It is called the speed of light. It gets this name because it is also found that light waves in empty space move in such a way that $ds = 0$ between events on the worldline, so their speed is given by $$ dx^2 + dy^2 + dz^2 = A^2 dt^2 $$ hence $$ v = \left( (\frac{dx}{dt})^2 + (\frac{dy}{dt})^2 + (\frac{dz}{dt})^2 \right)^{1/2} = A $$

As is widely known, the standard letter used for this constant is $c$.

So much for the speed of light according to the standard definition of terms in physics. It is the same everywhere and it does not depend on any direction of travel.

Now if one chooses to adopt other systems of coordinates, then one can find coordinates say $T,X,Y,Z$ in which the worldline of a light ray can have $dX/dT = c/2$ when travelling in one direction, and $dX/dT = \infty$ while travelling in another direction. Quantities of this kind are called "the coordinate speed of light". They vary from one choice of coordinates to another, and do not tell us much of any relevance to physics.

Here is an example.

Let $x,t$ be ordinary coordinates which can be used, for example, to describe the motion of things moving along a line between Earth and Mars, where we align the $x$ axis with this line (the line will stay still to good approximation during the few tens of minutes required for the motions we will discuss). Now define two other variables as follows: $$ X \equiv x, \;\;\;\;\;\; T \equiv t + x/c $$ These are definitions. The variables $X,T$ are a pair of quantities which I simply decided to define this way.

Now let's consider something moving along the $x$ axis. If its speed is $v$ then $dx/dt = v$ for motion in one direction, and $dx/dt = -v$ for motion in the other direction. We can track the motion also using the $X,T$ coordinates. We have $$ \frac{dX}{dt} = \frac{dx}{dt} = \pm v $$ and $$ \frac{dT}{dt} = 1 + \frac{1}{c} \frac{dx}{dt} = 1 \pm \frac{v}{c} $$ therefore $$ \frac{dX}{dT} = \frac{dX/dt}{dT/dt} = \frac{ \pm v }{1 \pm v/c}. $$ For example, in the case of a light pulse, where $v=c$, we shall find $$ \frac{dX}{dT} = \frac{c}{2} $$ in one direction and $$ \frac{dX}{dT} = \infty $$ in the other direction.

So is the light moving instantaneously from Mars to Earth? No: it is just like the different clock settings in France and England that I started with. The "clocks" indicated by $T$ have been arranged such that a clock on Mars is ahead of one on Earth. Amazing as it may seem to anyone who watched the Veritasium video, there really is no more to it than that. It is all based on a human decision to refer to the parameter $T$ as "time".

If we choose to use the unadorned phrase "speed of light" to mean "coordinate speed of light", without making it crystal clear that that is what we are doing, then we shall merely mislead people, as the video mentioned in the question clearly has mislead the questioner. The phrase "one way speed of light" will alert experts to the fact that something more technical and non-standard is being referred to, but that nuance will not be picked up in the context of popular presentations. It then appears that we are saying that light could really travel from Mars to Earth in the blink of an eye, crossing a spacelike interval. But light cannot cross a spacelike interval. So if one appears to be saying that light signals can cross a spacelike interval, without adding unambiguously that in fact this is not possible, then I think one is being misleading.

The answer to the three questions listed at the start is, then: "if someone asserts that light can move between different locations instantaneously then beware: they may be adopting some non-standard way of dissecting spacetime using coordinate systems, and they may be using the terminology "speed of light" in a misleading way".

Answer 3

Yes, it can. And it can get even worse. The real gist of what's going on here is that, in relativity theory, how 'speed' is defined is arbitrary, which is a consequence more generally of that the selection of a present "now" is arbitrary.

Remember, speed requires us to talk about how much distance in space something covers over time - thus, it requires a separation of spacetime into distinct "space" and "time" components, and most importantly, that apply at distant places from us, so we can sample the motion at two points, check their spatial coordinates, measure the distance in space, measure the time required to traverse, and finally take the quotient to get the speed.

Now, you may be thinking of those cute diagrams they draw that show a separate space and time axis, and how that you can change those by a Lorentz transformation, and so forth. But this is the thing: those diagrams are arbitrary. There is nothing special about that axis "$x$" there, that makes it a necessary, logical consequence of the structure of spacetime. It's a pure artifice, and that also means that everything talked about in terms of it is, to the extent it relies upon it, is also pure artifice as well. This means the idea of measuring the "length" of an extended object is arbitrary (so should you be surprised it has contraction with movement now?), as is measuring the time "you see" between two distant events.

And the "speed of light" is measured with regard to that axis. But there's nothing stopping us from using a different axis, and if we do so, we will get a different scenario for this speed.

Now the reason there's the "$\frac{c}{2}$ vs. $\infty$" limit is because while yes, these things are arbitrary, not all of spacetime's features are arbitrary or the theory would be completely devoid of content. Instead, the following things are not arbitrary, i.e. they are structural features of the spacetime:

1. At any particular event (point in space-time), the tripartite division of the surrounding events into timelike, lightlike, and spacelike domains,
2. The spacetime interval, or action bonus of communication, from one point to another point.

And these do impose some constraints on how you can draw your $x$-axis, but they permit still a lot of freedom: namely, each point on your $x$-axis, or your spatial plane, has to be spacelike from each other point. To measure a speed of light of $\infty$, you need a segment of your $x$ axis coinciding with the path of a light signal. This is a lightlike path, and that is a limiting case of spacelike (and timelike) paths, so it is also a limiting case of "possible $x$-axes".

Answer 4

The first estimates for the speed of light used the delay in the time of occultation of the satellites of Jupiter. When the Earth was on the opposite side of the sun the time was delayed and as the earth moved to the same side it went back to an earlier time. This would be a one way experiment. This is similar to the previous answer using clock on Mars compared to a clock on Earth.

Answer 5

There is a theory, in which light propagates isotopically only in one frame, so – called “preferred frame”.

According to Special Relativity, one – way speed of light is isotropic in all inertial frame of reference. According to Lorentz Ether theory, speed of light is isotropic only in “preferred” frame, or Ether.

The introduction of length contraction and time dilation for all phenomena in a "preferred" frame of reference, which plays the role of Lorentz's immobile aether, leads to the complete Lorentz transformation. Because the same mathematical formalism occurs in both, it is not possible to distinguish between LET and SR by experiment

Moreover, one - way speed of light is definitely anisotropic relatively to the Earth surface.

Imagine giant ring of arbitrarily large diameter. Assume, that the ring is rotating clockwise in certain inertial laboratory, say S.

Assume that there is a laboratory S’ on the rim of this ring. This laboratory is moving with linear velocity v. Velocity v is very close to c. The ring is so huge, that this laboratory S’ can be considered as quasi-inertial. Let there are two clocks on the rim of the ring – A and B.

Imagine, that an observer, who is in the center of this rotating ring flashes light with the aim to synchronize these clocks. This observer employs Einstein synchrony convention, which implies isotropy of the one – way speed of light. As soon beams of light reach these clocks, since these clocks are equidistant from the center, these clocks can be considered as synchronous.

Now, assume that an observer in the moving (rotating) laboratory S’ wants to measure one – way speed of light on the segments A-B, B-A, B-A-B, A-B-A by means of these clocks. An observer in the center of the ring may “see”, that beam of light is moving very, very slowly from A to B but very, very fast from B to A.

However, measured by the observer S’ speed of light in direction from A to B or from B to A will not be c-v or c+v, because we must remember that distance A-B Lorentz – contracts and clocks A and B slow down.

Due to these effects the laboratory would be "distorted" and measured by these clocks one - way speed of light from A to B will be very close to c/2. On the way back, from B to A the beam of light will cover this distance almost instantly.

Sure, measured two – way speed of light, say A-B-A or B-A-B will be isotropic and equal precisely to constant c, see Michelson Morley experiment.

Hence, from the point of view of moving observer S’ the clock would appear as Reichenbach – synchronized.

Sure, an observer S’ on the rim of rotating ring may synchronize clocks A and B Einstein – way, and measured by these clocks one way speed of light will be isotropic. A little problem emerges, however - If clock A and clock B are not synchronized directly, but by using a chain of intermediate clocks, the synchronization depends on the path chosen. Synchronization around the whole circumference of a rotating ring gives a non vanishing time difference that depends on the direction used.

Answer 6

This interesting question since the asymmetry in speed could occur in either path of light travel, direct path or reflected return path of light can be also broken down and translated to the following series of two questions:

1. Is "empty space" uniform?

2. Is reflected or scattered light by an object back to empty space slower?

Answers:

1. This question involves the direct path of light traveling in empty space from its generation source to a target. Astronomical measurement data and experiments so far supports that in general direct light coming from different star (light generation source) positions and distances in the sky travels with the same speed c. This pretty much proves that space is uniform in general and the direct path of light from a light generation source is not affected by space and independent of its relative orientation in space to a target.

2. It is proven by experiments also, that reflected or scattered light by an object back to us (return path of light) that allows us to see the object in the first place, is not slowed down by the process,

Does light accelerate or slow down during reflection?

Combining the two answers above IMO it is safe to say that Einstein's postulate is correct and light travels in both paths, direct and return path with the same speed c in empty space.

Answer 7

You are overthinking it. Fancy equations that I don't understand are not the solution. Using an example based on Veritasium's video, if it was 12:00 on Earth and the message took 20 minutes to arrive, on Mars they would think it was sent 10 minutes ago, so they would set their clocks to 12:10. They would then send a message that it was 12:10, and the people on Earth would think it was sent at 12:10, 10 minutes ago. They would then go back to Earth and measure the time delay. If it was off by 10 minutes, the speed of light would be infinite. If it was off by a different number, they would calculate the speed based on the fact that if it was off by 0 minutes it would be 10 minutes to earth, if it was 12:00 on Earth and the message took 19 minutes to arrive, on Mars they would think it was sent 10 minutes ago, so they would set their clocks to 12:10. It would actually be 12:19. They would then, in 1 minute, send a message that it was 12:10, and the people on Earth would think it was sent at 12:10, 10 minutes ago.Then, it would be 12:11 on Mars, and 12:20 on Earth. They would measure it as a 9 minute difference, meaning Mar's message took 1 minute to reach them. And so on and so on. They would calculate the distance between Earth and Mars when this happened, then divide it by the the time taken for the signal to travel, ultimately getting the speed of light in that direction. They would do the same for Earth to Mars. They would divide the distance from Earth to Mars when that happened by the 19 minutes to travel from Earth to Mars. And, Voila! One-way light speed requires space travel!

Answer 8

No it cannot be instantaneous. Like some other physics videos by Veritassium, that one is mostly nonsense.

If you assume that you are in what Einstein called “the stationary frame”, then by definition the one-way speed of light is the same in all directions. In a frame moving at C/2 relative to you, in the frame's direction of travel, the speed of light in one direction will be C/2 and in the other direction 3C/2. The limiting case is where the frame is moving at nearly C relative to you, in which case the speed of light is nearly 2C in one direction, and nearly 0 in the other. Somebody in the moving frame measures the one-way speed of light to be the same due to length contraction, time dilation, and the one thing Veritassium got right, clock synchronisation.

Answer 9

Yes. The one way speed of light can approach instantaneous (but will never reach it) BUT only if you ignore or abandon something called Relativity of Simultaneity.

One way speed of light (OWSOL) is the antithesis of Relativity of Simultaneity. The two can’t coexist. There does however seem to still be some interest in promoting One way speed of light, and breaking some laws of physics that stand in its way. The Veritasium video has over 17 million views, and over 100,000 comments (July/2022). Interestingly, the YouTube video asks the question ‘is it possible?’ without ever offering any clues indicating how you would actually answer the question.

So, just for fun, and hypothetically speaking, here is one possible way of calculating the one-way speed of light, ignoring Relativity of Simultaneity in the process.

. . .

Below is a thought experiment where I have drawn out the position of one way light though time frames [T0 – T7] in two different but similar situations: Light behavior in open space, and light behavior within an enclosed object moving through space.

The 2nd diagram indicates that the one-way speed of light (OWSOL) as shown in the moving frame of reference is not constant.

As shown within the moving object in Diagram 2, the photon moving in the forward direction of travel will hit the front wall later in time than the photon moving in the reverse direction of travel hits the back wall.

As the moving object’s speed approaches the speed of light, the photon’s speed in the forward direction (measured against the moving object) approaches zero, while the photon’s speed in the reverse direction approaches instantaneous.

Diagram 1: The light bulb is traveling through space moving at 50% the speed of light to the right. The second green dot light bulb is stationary. Photons are emitted from the moving and stationary light bulbs in the forward and reverse direction at exactly the same time as they pass each other in space.

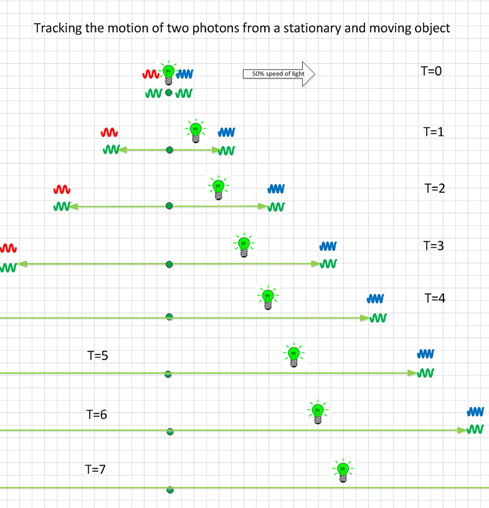
Photons emitted from the moving light bulb are blue shifted in the forward direction, and red shifted in the reverse direction.

Photons emitted from the stationary light bulb are not frequency shifted, but travel through space at exactly the same speed as the frequency shifted photons.

Diagram 2: Same diagram as above, but now with a wire frame box centered around the moving light bulb.

The speed of light plotted inside the moving box is the same as the speed of light plotted outside of the moving box, as the absolute speed of light is constant in all frames of reference.

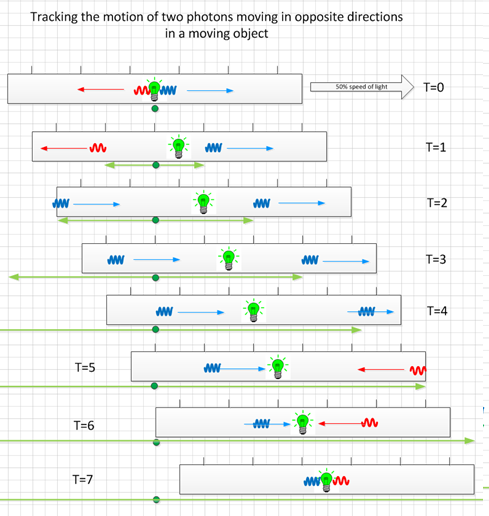
Observation: From the perspective of a person standing inside the box, the forward one-way speed of light in the moving frame of reference (blue photon) is considerably slower than the reversed one-way speed of light (red photon). The two-way speed of light remains constant in all directions.

Advanced Notes: Assume the diagrams are from a computer simulation, and that the simulation can be stopped at any point in time to view the exact location of the photon (Ignores the issues of simultaneity, but still allows us to discuss what’s happening outside of the box, and what is happening inside the box). Also assume that the correct Lorentz adjustment have been made to the moving object, where to the outside observer in the stationary frame, as the speed of the object passing through space approaches c, within the moving object time comes to a stop, and the horizontal contracted length of the object approaches zero.

Supporting Proof:

Below are two diagrams showing the direction light travels when emitted at 90 degrees to the direction of travel.

Diagram 3:

Diagram 3 shows a flashlight traveling at different horizontal velocities through space emitting light in in a vertical direction.

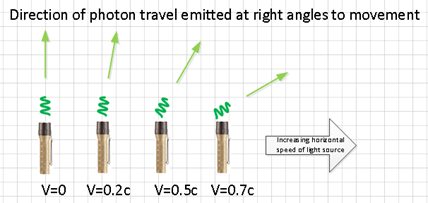
Diagram 4:

Diagram 4 plots an emitted photon as it travels upwards inside an enclosed box moving right at 0.7c

I'm making the point that the the behavior of a photon in a vacuum emitted in the rest frame, and a photon emitted in a moving frame, is the same. The location of the photon plotted against the rest frame is in the same location in space as the photon plotted against the overlaid moving frame of reference. This relationship has a basis in physics, and can be used to derive the Lorentz factor. Link:( https://medium.com/physics-scribbles/deriving-the-lorentz-factor-%CE%B3-of-special-relativity-d5462f3b3b91)

Once launched, the only thing that can change the direction of travel of the photon is to be absorbed, reflected, pass through a transparent medium, or pass through a gravitational field.

So to establish the mathematical framework for the behavior of the photon in the moving object frame, one need only plot the behavior of the photon in the rest frame, and then map that position to the moving object frame, taking into account any objects or walls that the photon hits or bounces off of.

Referring to figure 2, to establish the time and position in the rest frame of when the released photon hits the right side of the box, all we need to do is set up the relationship for distance traveled, taking into account the fact that as a frame of reference approaches the speed of light, in that frame, time slows down, and the object contracts in length in the direction of travel.

If the length of the front half of the box at rest is 1 light-second, and the Lorentz factor at 50% the speed of light is 0.8660, then:

Calculation for when the released photon hits the right side of the box:

  Speed of light * time = (speed of the right side of the box * time) 
         + Lorentz modified length of the box.

  c * t = (0.5c * t) + 0.866025

 0.5t = 0.866025

 t = 1.732051 

The photon hits the right side of the box at time t=1.732051 in the rest frame.

A similar calculation for when the released photon hits the left side of the box:

Reverse Speed of light * time = (speed of the left side of the box * time)
   – Lorentz modified length of the box.

-c * t = (0.5c * t) – 0.866025

-1.5t = - 0.866025

t = 0.57735

The photon hits the left side of the box at time t=0.57735 in the rest frame.

On the return trip, the now forward traveling photon takes 1.732051 seconds to reach the center of the box, and the now reverse traveling photon takes 0.57735 seconds to reach the center of the box, for a total 2-way speed for both photons of 1.732051 + 0.57735 seconds = 2.309401 seconds.

Doing the translation to the moving frame of reference, multiplying by the Lorentz factor 0.866, gives a moving frame of reference time for the speed of two-way light of 2.309401 * 0.866 = 2 seconds in the moving box. The one-way time to reach the right side of the box is 1.732051 * 0.866025 = 1.5 seconds. The one-way time to reach the left side of the box is 0.57735 * 0.866 = 0.5 seconds.

Within the moving frame of reference the speed of light in the forward direction is 1 light second of distance traveled in 1.5 seconds = 0.66667x the speed of light. In the reverse direction, the photon travels 1 light second in 0.5 seconds = 2x the speed of light.

As the moving box approaches the speed of light, the speed of the forward moving photon in the moving box frame of reference approaches an internal time of 2 seconds (50% the speed of light), and a reverse direction speed of close to instantaneous.

Note that if the box travels at 99.99% the speed of light, the measured time to travel 1 light second in the forward direction as measured in the rest frame is 2 / 0.0141 = 142 seconds. At 99.9999% the speed of light, The value is 2 / 0.00141 = 1418 seconds, and goes up exponentially from there.

Supplemental note:

Relativity of Simultaneity postulates that in each reference frame, one-way light travels at the speed of c, and any appearance otherwise is the difference between an actual and a perceived event, in that you can only measure the perceived event. If a calculation leads to two possible outcomes (differences in what two different observers see), this is known as a paradox of simultaneity. That leaves it up to you to decide which calculated answer is correct.

Trying to build a computer simulation of a complex behavior mapping multiple moving frames of reference interacting with a photon, all the while incorporating Relativity of Simultaneity, is a nightmare. I’m not even sure it can be done. My understanding is that the most complex synchronized set of clocks ever built, known as GPS, does not use Relativity of Simultaneity in its calculations.

As a thought experiment, if you were to imagine a world where ‘Relativity of Simultaneity’ did not exist, what terrible things would happen?

• The world you live in would continue to exist as it does today.
• The GPS satellite system would continue to operate unmodified, as it does not use ‘Relativity of Simultaneity’ in any of its calculations involving spacetime.
• The derivation of the Lorentz Factor would remain the same, as the Lorentz factor does not rely on the concept of ‘Relativity of Simultaneity’ in order to correctly calculate time dilation or length contraction differences between moving frames of reference.
• The speed of light c remains a constant in the rest frame.
• The two-way speed of light c continues to be a constant in all frames of reference.
• Physics would have to recognize the one-way speed of light, which is c in the rest frame, but can be less than c, or more than c as measured in a moving frame, dependent on the direction of travel of the photon relative to the local frame, and the direction of travel of the local frame relative to the rest frame.
• All the paradoxes of special relativity would suddenly resolve themselves, and cease to exist.
• Simulations of the universe can go back to predicting the location and time that events occur in ‘all frames of reference’ without having to pretend it’s OK that an event may or may not occur in two different places, or at two different times, depending on who’s perspective is used of the multitude of people who are viewing the event.
• It becomes possible to identify ‘the absolute rest frame’, closer aligning classical physics with quantum physics. That also opens a logical path back to absolute time, and absolute location.

The issue being, no one has figured out a way to measure the one-way speed of light, or how to synchronize clocks, and Relativity of Simultaneity says that doing so is impossible, so don’t even bother trying. However, in an imagined world without Simultaneity, I wonder if that limitation would still be true?