The is a complex question and to cover it we need to look in depth at how tires generate tangential force on the road (as opposed to normal force that is perpendicular to the road).
The answer is of course friction $F=\mu N$ but in this case the answer is complicated by the fact that the rubber surface of the tire deforms, and thus rather than simply taking the normal force multiplying by a friction coefficient and arriving at our peak force, we actually have to integrate the shear stress across the contact patch.
Definitions
The coordinate system is defined relative to the contact patch.
$$\begin{align*}
x &\equiv \text{nominal distance from front of contact patch} \\
y &\equiv \text{distance from left edge of contact patch} \\
s &\equiv \text{length of the contact patch} \\
v_t &\equiv \text{nominal tire surface velocity} \\
v_r &\equiv \text{road velocity} \\
\epsilon &\equiv \text{displacement of tire surface from nominal} \\
\sigma &\equiv \text{surface stress at the tire/road interface} \\
E &\equiv \text{tire stiffness} \\
\mu_s &\equiv \text{static coefficient of friction} \\
\mu_d &\equiv \text{dynamic coefficient of friction} \\
P &\equiv \text{contact pressure} \\
\gamma &\equiv \text{slip rate} \equiv \frac{v_r-v_t}{v_t}
\end{align*}$$
Assumptions
Constant tire stiffness
Second assumption: To calculate what the shear stress is at the tire surface we're going to presume the the shear stress is proportional to the displacement of the tire surface from where it would be if it were not touching the road surface.
$$\sigma = E \epsilon\tag{1}$$
Uniformity across the tire width
The contact pressure is nearly constant across the width (at least if you ignore treads) so if we pretend everything is uniform in that direction then we can take our 2D problem and make it 1D
$$\frac{\partial}{\partial y}=0$$
Steady state
We're going to ignore dynamic effects like relaxation length and assume everything that's happening to the tire right now has been happening for a while.
$$\frac{\partial}{\partial t}=0$$
Slipping or not slipping
We're going to assume for each little segment of the tire either it moves along with the road, not slipping at all, or that it is sliding and thus has a shear stress equal to the normal pressure multiplied by the dynamic coefficient of friction.
For the no slip condition this results in the tire surface moving at $v_r$ instead of $v_t$
so relative to a spot on the tire that moves within this no slip region:
$$\frac{d\epsilon}{dt}=v_r-v_t$$
Note that this implies an ever increasing displacement, and therefore stress. Once this stress exceeds the static friction, this spot will begin slipping and as the non zero velocity difference would ensure that it remained slipping.
$$\frac{dx}{dt}=v_t$$
$$\frac{dt}{dx}\frac{d\epsilon}{dt}=\frac{v_r-v_t}{v_t}=\gamma$$
$$\frac{d\epsilon}{dx}=\gamma\tag{2}$$
$$\sigma \leq \mu_d P\tag{3}$$
For the slipping region:
$$\sigma = \mu_s P\tag{4}$$
Putting it together
Since we know that the contact pressure at the front and rear of the contact patch approach zero, we know that the shear stress must also approach zero.
$$\sigma(x=0)=\sigma(x=s)=0\tag{5}$$
$(1)$ & $(5)$
$$\epsilon(x=0)=\epsilon(x=s)=0\tag{6}$$
If we assume that the tire is in the no slip condition at the beginning then we have $(2)$ & $(6)$:
$$\epsilon(x)=x\,\gamma\tag{7}$$
$(7)$ & $(1)$
$$\sigma(x)=x\, E\,\gamma\tag{8}$$
$(8)$ & $(3)$
$$\mu_s P \geq x\, E\,\gamma$$
$$x \leq \frac{\mu_s P}{E\,\gamma} $$
This implies that there's a maximum x such that before that x the rubber does not stick and after that the tire does slip. It would be useful to define this distance $x_t$
$$x_t \equiv \frac{\mu_s P}{E\,\gamma}\tag{9}$$
$(4)$, $(8)$, & $(9)$
$$\sigma(x)=\left\{
\begin{array}{ll}
x\, E\,\gamma & \quad x \leq x_t \\
\mu_d P(x) & \quad x \gt x_t
\end{array}
\right.$$
Our total friction force is given is the integral of the stress so:
$$F=\int_0^{x_t} x\, E\,\gamma dx + \int_{x_t}^s \mu_d P(x) dx$$
$$F=\frac12 E\,\gamma \, {x_t}^2 + \mu_d\int_{x_t}^s P(x) dx$$
At this point it would be helpful to define a pressure profile for the tire. Unfortunately the shape of this profile depends on a number of factors including tire type, the level of inflation, and current loading.
Parabolic
The simplest non-zero polynomial that has at least two zeros. A parabola is a decent approximation for highly inflated stiff tires.
$$P(x)= \frac{6L}{s^3} (s\,x - x^2)$$
Where L is the total normal load on the tire.
$$F=\frac12 E\,\gamma \, {x_t}^2 + \mu_d \,L \left(1-3\left(\frac{x_t}{s}\right)^2+2\left(\frac{x_t}{s}\right)^3\right)$$
$$x_t = \frac{\mu_s P(x_t)}{E\,\gamma}$$
$$x_t=s-\frac{E\,\gamma\,s^3}{6\,\mu_s\,L}$$
Substituting and a lot of simplification yields:
$$\frac{F}{L\,\mu_d}=\left(3\frac{\mu_s}{\mu_d}-\frac23\right)\left(\frac{E\,s^2\,\gamma}{6\,L\,\mu_s}\right)^3+\left(3-2\frac{\mu_s}{\mu_d}\right)\left(\frac{E\,s^2\,\gamma}{6\,L\,\mu_s}\right)^2+3\frac{\mu_s}{\mu_d}\frac{E\,s^2\,\gamma}{6\,L\,\mu_s}$$
Lets take a look at these variables:
$$\frac{E\,s^2\,\gamma}{6\,L\,\mu_s}$$
Now $\frac{\gamma \, s}2$ is the average displacement that would occur there was no slipping. And $E\,s\,\epsilon_{ave}$ is the force. So $\frac{E\,s^2\,\gamma}2$ is the force that would occur if there was no slipping.
$L\,\mu_s$ is the force that would occur if the entire patch was slipping. Thus we can call this variable a normalized slip rate $\gamma'$
Note the ratio of static to dynamic friction also appears multiple times. We will define this ratio as $\mu'$
$$\frac{F}{L\,\mu_d} = (3\mu'-2){\gamma'}^3+(3-6\mu'){\gamma'}^2+3\,\mu'\,\gamma'$$
This equation can now easily be plotted for a for a given friction ratio
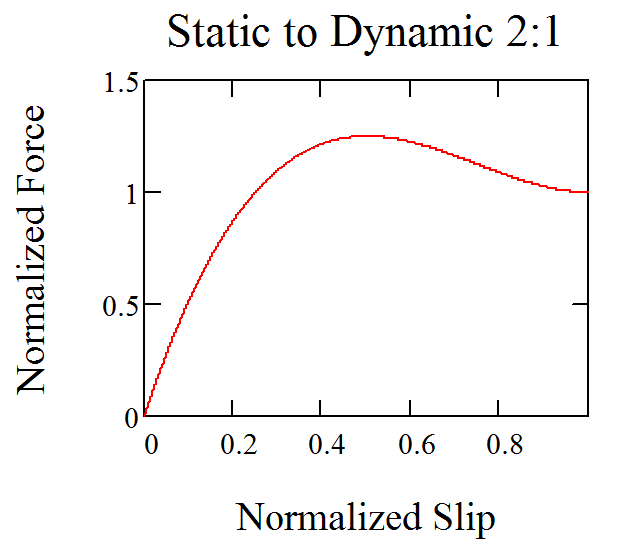
or a more reasonable
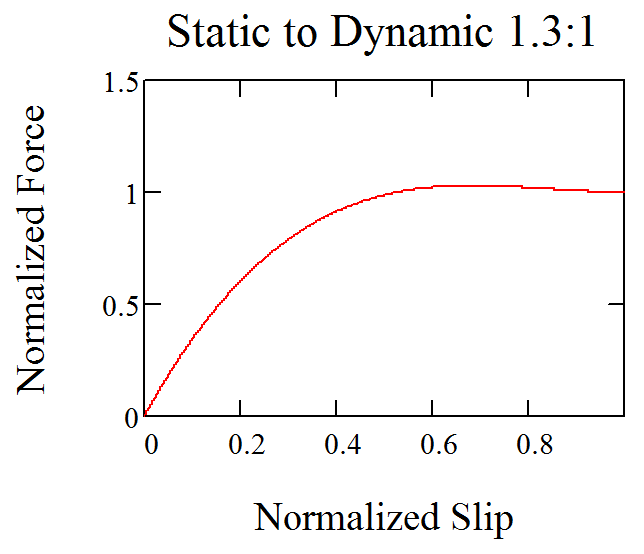
Note that as the slip goes towards full slip the plot levels off at one to indicate that the force levels off at full slipping force once the tire starts fully slipping. Also note that before the tire is fully slipping part of the tire is not slipping and thus providing the higher coefficient of static friction. Thus, the real reason that tires provide more cornering power while partially slipping. This allows a maximal use of static friction.
Also note that in this analysis a direction was never assumed for $v_r-v_t$, $\epsilon$, $\sigma$, and $F$ other than that they all were in the same direction. Thus with the approximations used, accelerating, braking, and cornering forces are all treated identically. If you'd like to use this for pure cornering then $\gamma=tan(\theta)$ where theta is the slip angle.
As for the pressure distribution assumed lets see if there's always a maximum before it starts slipping. There will always be a maximum if the slope is ever negative as it will be decreasing from a maximum.
$$\text{sign}\left(\frac{dF}{d\gamma}\right)=-\text{sign}\left(\frac{dF}{dx_t}\right)$$
$$\frac{dF}{dx_t}=\frac12\mu_s x_t \frac{d P(x_t)}{dx_t}+\left(\frac12\mu_s - \mu_d\right) P(x_t)$$
So this quantity must always be non-positive:
At $x_t=0$
$$\frac{dF}{dx_t}=0$$
Therefore if $\frac{d^2F}{{dx_t}^2}\gt 0$ at $x_t=0$ there will be a maximum.
$$\frac{d^2F}{{dx_t}^2}= (\mu_s-\mu_d) \frac{d P(x_t)}{dx_t} + \frac12 \mu_s x_t \frac{d^2 P(x_t)}{{dx_t}^2}$$
At $x_t=0$
$$\frac{d^2F}{{dx_t}^2}= (\mu_s-\mu_d) \frac{d P(x_t)}{dx_t}$$
Which must be positive for all continuous pressure distributions. So for any realistic pressure distribution there will be a maximum force that occurs before the tire is fully slipping.


