You know the strong force (the one that keeps quarks together). Well it works by exchanging gluons right? So how does that force keep the quarks together? I mean you can imagine that process as three people passing balls between them right? Well as far as I know that throwing of the ball wouldn't force those 3 persons to stay within a range. I had this one idea that when a gluon is emitted it results in a force that pushes the quark in the opposite direction but that would be towards the outside of the quark right? Pls explain this to me. Any help would be helpful and greatly appreciated.
5 Answers
You have stumbled into one of the most interesting questions of QED and QCD, that is, how can we model the attractive and repulsive forces by the exchange of the massless mediators (photon and gluon respectively)? The answer is mathematically very complicated and when we look for an explanation in our everyday classical view, there is a very nice analogy:
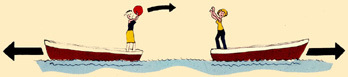
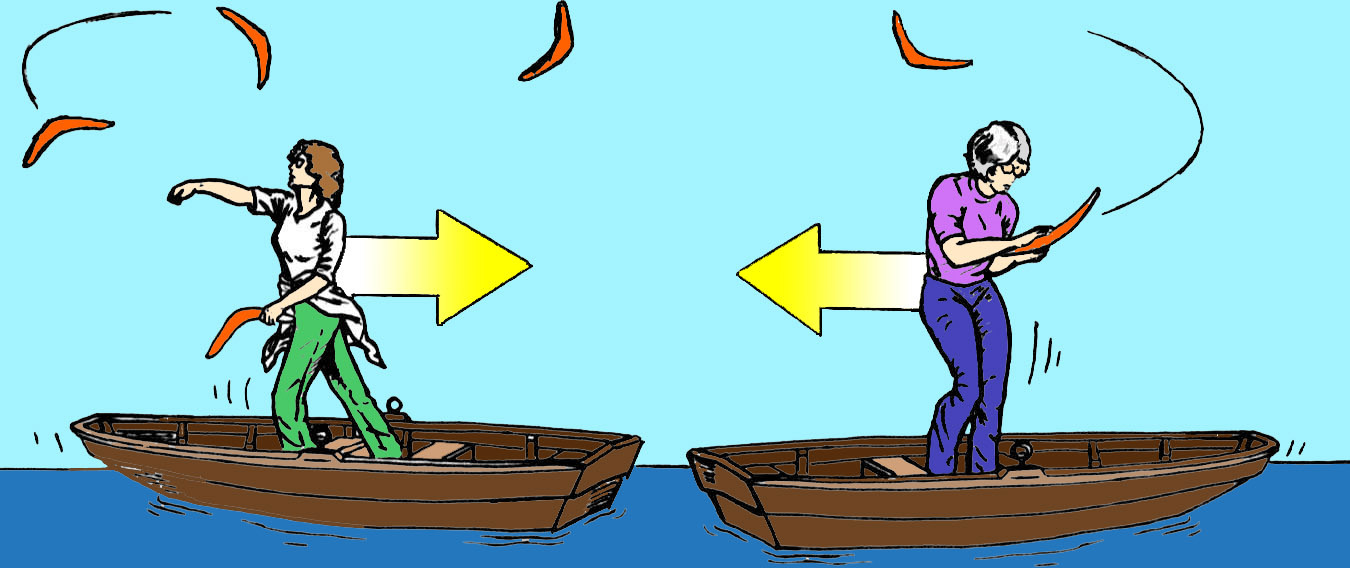
These are very nice classical analogical explanations of how momentum conservation laws can be obeyed by the exchange of the mediator particles (in your case gluons). For repulsive forces, it is easier to understand by throwing balls at each other, but attractive forces are a little bit harder to understand classically, these boomerangs can give a nice analogy.
How can photons cause charges to attract?
All internal lines in a Feynman diagram are force carriers, i.e. transfer dp/dt by construction,not only the gauge bosons. See the diagram for compton scattering for example. Lattice QCD goes for direct solutions on the lattice, and therefore the concept of virtual particles is not necessary. It is a different calculational approach , although the article involves quark propagators in the calculations.
Are force carrying particles always virtual particles?
It is very important to understand that usually these are mediator exchanges are described using a mathematical model that uses virtual particles (like virtual photons), although in the case of lattice QCD virtual particles are not necessary.
-
$\begingroup$ that is a very nice analogy...thanx...but I don't see how a virtual photon(or any other particle)would go around the two particles(at least according to the laws I know)...So can you tell me the mathematical explanation(you know....the one that has no real analogy or intuition)...thanx in advance $\endgroup$ Commented Sep 30, 2020 at 0:07
-
-
Unfortunately, there is no nice answer.
The cop out answer is that the quantum world is weird and your picture of balls doesn’t really work at that level.
A slightly better answer is: the gluons exchanged are virtual, this means they don’t really exist which allows them to behave in ways which are classically forbidden.
-
$\begingroup$ Then why did scientists come up with that answer in the first place...I mean they must've thought that quarks are kept together by the strong force for a reason right? A reason that mathematically and logically works out right?...I completely agree with you on qm being weird though. I know that I can't use the 3 people ball analogy but its the best one I could come up with...A more accurate one would be one where all three are on skateboards but that would just prove the theory wrong cuz if one throws the ball while he's on a skateboard then that would just push him away from the other two $\endgroup$ Commented Sep 29, 2020 at 11:50
-
$\begingroup$ Well, the proper theory is actually quantum field theory and in this, all notions of particles are mere analogies to make the maths feel more intuitive. The origins of both qft and the strong force (quantum chromo-dynamics) are very mathematical $\endgroup$ Commented Sep 29, 2020 at 12:50
The people-throwing-balls-at-each-other analogy doesn't really work. In quantum field theory, all interactions happen through particle exchange, but the situation really isn't at all similar to any analogy that I've ever heard in the classical mechanics. The explanation is in the math.
Particles interact with each other through a field (like the electromagnetic field or the gauge field), and when we apply the laws of quantum mechanics to a field, we find out that the energy of the field can only come in discrete chunks (quanta) which we associate with particles. For example, for the electromagnetic field, the associated particle is the photon, so electromagnetic interactions, mediated by the electromagnetic field, take place through the exchange of photons.
-
$\begingroup$ thanx for the answer but electromagnetic interractions have a logical interaction that results in forces...for example the reason electrons repel is because when it gets close to another electron it emits a photon towards that electron which causes it to move away from the other electron(newtons third law)...But this has no logical explanation $\endgroup$ Commented Sep 29, 2020 at 12:04
-
$\begingroup$ The electromagnetic force can be attractive as well. The force between a proton and an electron is attractive, and is also meditated by the exchange of photons. Newton's third law has nothing to do with it in either case. It's a very limited and misleading analogy. $\endgroup$ Commented Sep 29, 2020 at 12:16
-
$\begingroup$ Well as far as I know that's wrong because protons are not fundermental particles so you'd actually have to apply newtons third law or some other law to the three quarks inside it $\endgroup$ Commented Sep 29, 2020 at 12:52
-
$\begingroup$ We apply the laws of quantum chromodynamics to get the quark structure of the proton, but that doesn't change the fact that it has an attractive interaction with the electron meditated by photons. If you prefer only fundamental particles though, the electron and positron also have an attractive electromagnetic interaction. $\endgroup$ Commented Sep 29, 2020 at 12:56
A simple reason why your ball-throwing-analogy is misleading, is that you cannot throw "virtual" balls, i.e. balls whose energy-momentum-relation is off. Furthermore, the "interaction points", where one particle sends off the exchange particle and the other catches it, are not localized.
When you go too far with the story about "exchange particles" it breaks down. I'd always rather think of the whole story as just a graphical representation off mathematical expressions. There is too much going on in QFT, especially in QCD where you wouldn't even find free elementary particles due to confinement.
The problem is that our classical intuition is simply wrong at that level, so it is futile to try to construct quasi-classical interpretations, imho.
The 'throwing balls at each other' analogy gives a really clear picture for a repulsive forces, but not for attractive forces. I've seen attempts with boomerangs and not-letting-go, but basically it doesn't work. I suspect that, even though it's often seen when popularising QED and other forces, we would be better off not using it. Sorry. But it's more confusing than helpful.
Let me offer - cautiously - an alternative which is not totally satisfactory but probably better than falling back on "It's all in the theoretical quantum stuff".
Between particles there is a field which is some function of the displacement between them. That function can be expanded as a Fourier transform - that's just basic mathematics. That's visualisable as sine/cosine standing waves.
Now a standing wave can be expressed as the sum of two travelling waves. $cos(kx)e^{i\omega t}=(e^{i(kx+\omega t)}+e^{i(-kx+\omega t)})/2$. If one particle absorbs one of the travelling waves, and the other particle absorbs the other, then each particle gets some momentum. These impulses are equal and opposite, and can be attractive or repulsive depending on which particle absorbs which wave.
-
$\begingroup$ thanx for the answer...but whats that field you mentioned?? $\endgroup$ Commented Sep 29, 2020 at 14:53
-
$\begingroup$ For charges and virtual photons, it's the usual electromagnetic field. For QCD it's a force between colours that is sort of similar (but sort of different) as well as being much stronger. $\endgroup$ Commented Sep 29, 2020 at 18:01
-
$\begingroup$ Isn't colour just a property?...Is there a thing as the colour force...you know like anyother force? If so, then could you pls send me an equation describing the colour force...gravity has an equation, electromagnetism has an equation and every other field forces have equations so the colour force must have one too right? thanx in advance $\endgroup$ Commented Sep 29, 2020 at 23:48
-
$\begingroup$ Yes there is a colour force. Colour is not just a label. Red attracts anti-red in the same way that positive (EM) charge attracts negative charge. Complicated because there are 3 colours and EM only has one 'charge', and because it's so much stronger. So QCD has an equation for the Lagrangian (see for example en.wikipedia.org/wiki/Quantum_chromodynamics) but, unlike QED, this cannot be carried over to the classical limit to get Coulomb's Law and such. You get confinement. Which nobody understands properly. $\endgroup$ Commented Sep 30, 2020 at 8:59