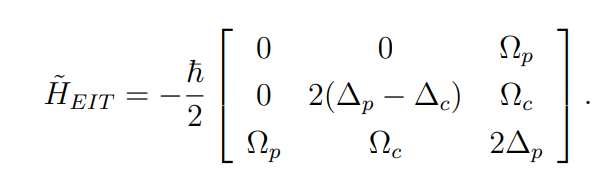Radiative transfer equation for a three-level system

Question

I am trying to derive the radiative transfer equation for a three-level system, which is supposed to be given by:

$\frac{dI(\omega,x)}{dx}+N [\alpha\rho_{11}-\beta( \rho_{22}+\rho_{33})]I(\omega,x)=\beta(\rho_{22}+\rho_{33})$,

where $I(\omega,x)$ is the specific intensity by radiation emitted due to a $|3>$ to $|1>$ transition, $\rho$ is the density matrix of the system, N is the atom density, $\alpha$ is the absorption and $\beta$ the emission cross-section.

From Monaco 1998 (https://doi.org/10.1080/00411459808205646) I found the corresponding equation for a two-level system. Can anyone help generalising this to the three-level case to arrive at the above equation?

Answer 1

The derivation is easy but a bit long, so I'm going to link a clear resource and just say the steps.

Step 1: Write the total Hamiltonian of the system

Step 2: perform rotating wave approximation and co-rotating frame to remove time dependence of problem. Your hamiltonian looks something like this now:

Step 3: Calculate using the "Master Equation": $\dot{P} = [P, H]$

This will give you the diff. eq. you're looking for.