When I was learning about indeterminacy, my teacher wasn't quite clear on why the trajectories of electrons were indeterministic. He made mention of the fact that we cannot know both the position and velocity simultaneously and they are needed in order to calculate trajectories. After that, he just said something briefly about two tennis balls, which when hit with the same force and under the same conditions, were landing on the same spot. Then he said electrons would behave differently (randomly) even when placed under similar conditions. So, Is the trajectory of an electron indeterministic due to our inability to know both the position and velocity of the electron simultaneously or due to the fact that electrons, under similar conditions, do behave differently?
3 Answers
TL;DR it‘s the inability to know the precise location and the precise velocity at the same time that causes the trajectories to be indeterministic (as you called it).
In this answer, I deliberately avoid any mathematics but try to explain the situation in layman terms. I hope it’s not too sloppy.
In QM everything you can possibly know about the electron is „encoded“ in its wave function. Preparing the initial condition of an electron in an experiment means fixing its wave function.
As an example: when electrons are sent through a hole (or a slit) you fix the position of the electrons to the location of the hole. After passing the hole, you can tell the position of the electron with an uncertainty that corresponds to the diameter of the hole. The smaller the hole, the more precisely you know the electron’s position. For the wave functions, this means: if you make the hole smaller you reduce the number of wave functions that are compatible with this initial setup (I know, it‘s an infinite number of wave functions but you get the gist of it).
In another experimental setup, you could prepare electrons to have a very specific velocity (it’s a bit harder to imagine such an apparatus, but it is actually possible to build one). Again, after passing this apparatus, you can tell the velocity of the electron only with some defined uncertainty.
(In your question you used the term velocity. The relevant physical property is momentum. But in this context the difference does not really matter).
Now consider the following experimental setup: first, you fix the velocity of the electron and after that its position. You would hope that all electrons passing through such a double apparatus would follow exactly the same trajectory afterward because the trajectory is completely determined by position and velocity at a specific point in time.
In QM, however, this does not work. The reason is Heisenberg's uncertainty principle which provides a fundamental property of wave functions in general: wave functions that correspond to a more precisely known position are unfortunately the very same that correspond to less well-known velocities and vice versa.
This is what happens in the double apparatus: after the first step, all electrons have wave functions that correspond to well-known velocities. When those electrons enter the hole, the process of fixing the position inevitably modifies the wave functions from “well-known-velocity“-wave functions to „well-known-position“-wave functions. This is called collapse of the wave function. It is called collapse because it reduces the number of wave functions that are compatible with the specific situation.
Remark: this ominous „collapse“ of a wave function is, to this day, ominous. It is highly disputed, essentially not understood and in general, considered to be a quite unsatisfactory concept.
Even for the 2 balls, one would not be able to have "exactly" everything equal in the beginning. So you can determine the trajectory of electrons quite well, even if the initial place and velocity are only known with the difference calculated by h. but you would be glad to determine the path of a ball so it would only differ by h. The path of your ball is also uncertain. With the small electron, this uncertainty is more visible than with the ball, but the general trajectory ist not fully unknown, If you know how an oscilloscope functions, you "see" the path of an e.
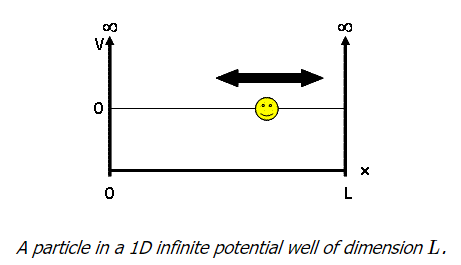
Electrons 'live' in quantum systems. So let's look at a simple (and highly educational) quantum system: an electron in $1D$ box with infinitely high walls:
If the electron was a Classical object, like a tennis ball, it would (in the absence of all friction) simply bounce back and forth between left and right wall for all of eternity. Its position and velocity would always be completely predictable (even at different kinetic energies)
But an electron isn't a tennis ball and doesn't behave like one.
Quantum systems have an associated wave function (usually denoted $\psi$) which contains all the information about the particle and is obtained by solving the Schrödinger equation, here:
$$-\frac{\hbar^2}{2m}\frac{\text{d}^2\psi(x)}{\text{d}x^2}=E\psi(x)$$ For: $$V(x)=+\infty\text{ for }x\leq 0$$ $$V(x)=+\infty\text{ for }x\geq L$$ $$\psi(0)=\psi(L)=0$$
The complete derivation and solution can be found here but need not concern us now.
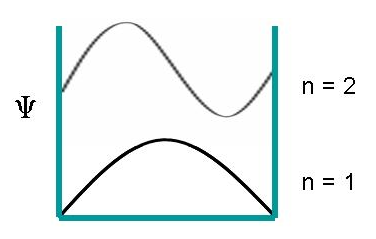
The wave functions for $n=1,2,3,...$ are:
$$\psi_n(x)=\sqrt{\frac{2}{L}}\sin\Big(\frac{n\pi}{L}x\Big)$$
The wave functions for $n=1$ and $2$:
The associated quantised energy levels:
$$E_n = \dfrac{n^{2}h^{2}}{8mL^{2}}$$
Note that a $\text{zero level}$ energy is not allowed (it would also violate the Heisenberg Uncertainty Principle)
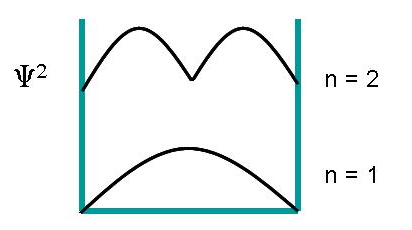
The probability density functions according to Born:
$$P(x)=|\psi(x)|^2=\frac{2}{L}\sin^2\Big(\frac{n\pi}{L}x\Big)$$
The probability density functions for $n=1$ and $2$:
The probability of finding the electron on a interval $(x,x+\Delta x)$ is given by:
$$\boxed{P(x\to \Delta x)=\int_x^{x+\Delta x}\frac{2}{L}\sin^2\Big(\frac{n\pi}{L}x\Big)\text{d}x}\tag{1}$$
For $\Delta x=0$, the probability is $\text{zero}$, which is in accordance with the Heisenberg Uncertainty Principle. The probability of finding the particle in a single point (say $x$) is in fact $\text{zero}$.
So the position of a electron in this quantum system is neither 'random' nor 'undetermined' but is in accordance with $(1)$. This principle applies also for many other quantum systems, atoms and molecules included. The term 'trajectory' is fairly meaningless when talking about quantum systems. Instead we can (very accurately) calculate the probability of finding the particle in a area of space (here $\Delta x$)