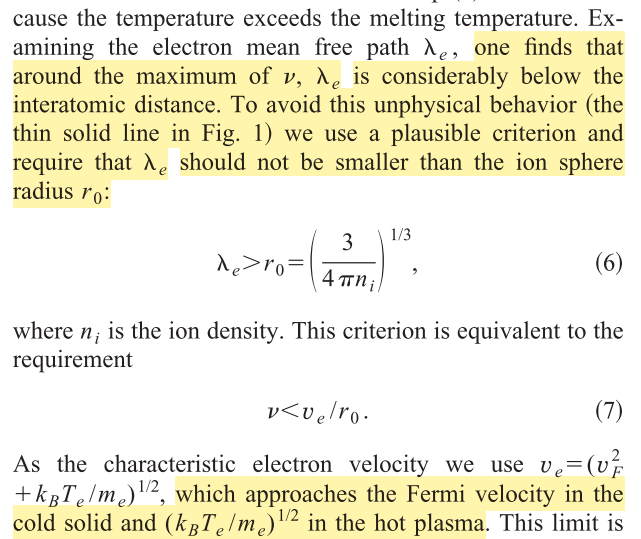
In an article (over 250 citations) on plasma physics I've read a paragraph which I find very confusing. The authors state that there's a minimum mean free path an electron travels before it is scattered which corresponds to the mean distance of ions $r_0$. According to them, a mean free path $\lambda_e<r_0$ is unphysical.
So, why does an electron need to travel at least a distance corresponding to the mean interatomic distance (or equivalently, the ion sphere radius), before it can be scattered? In my opinion, it might be scattered by another electron just as well, which is very likely to be way closer than the nearest ion (In a plasma, i.e. there are usually more electrons than ions).
Any ideas?
EDIT: The only idea that comes to my mind is that electron-electron collisions are negligible compared to electron-ion collisions in a plasma.