Is this a solution of Einstein's equations?

Question

Take infinite space. $\mathbb{R}^3$ Then cut a sphere (a 3-ball) out of it and discard it.

You now have $\mathbb{R}^3\backslash B_3$.

Now take each point on the surface of the hole and identify it with it's antipodal point on $S_2$. So it is like a self-wormhole.

You now have a space with a topological defect in it which seems like it would persist eternally.

I wonder if this topology is consistent with Einstein's equations of General Relativity?

I suppose the question is, can there be such a solution that is Ricci-flat? Or can it exist in a universe with non-zero cosmological constant?

Answer 1

… [I]f this topology is consistent with Einstein's equations of General Relativity? … [C]an there be such a solution that is Ricci-flat?

Short answer: Yes. The resulting Ricci flat solution is known as $\mathbb{RP}^3$ geon, and is a $\mathbb{Z}_2$ quotient of Kruskal–Szekeres extension of Schwarzschild spacetime. Though the nontrivial spatial topology is hidden from outside observer behind black hole horizon, such spacetime and its various generalization serve as important yet simple examples for various branches of general relativity. For instance, correlations in the Hawking-Unruh effect are affected by the topological features of such spacetime.

Long answer: First we note, that by itself topology does not specify a solution of general relativity. We can try to make this manifold into a spacetime by using the euclidean metric on $\mathbb{R}^3$ and then adding time without any position-dependent time dilation (this would make it an ultrastatic spacetime). As a result there would be $\delta$-like singularities of the curvature and Ricci tensors and Ricci scalar on the $\mathbb{RP}^2$ surface, where the cut and identifications were made. Most easily this could be seen by probing such spacetime with geodesics congruences, for example a congruence of ingoing radial geodesics. After passing through the surface, geodesics turn into outgoing and the expansion scalar of this congruence changes sign. By the Raychaudhuri equation this means that certain contraction of Ricci tensor has a $\delta$-like singularity.

In order to make a proper Ricci-flat spacetime $(\mathscr{M},g)$ with a spatial hypersurface $\mathscr{S}$, a 3-maniflold of a given topology, we must supply $\mathscr{S}$ with a Riemannian 3-metric $\gamma$ and a second fundamental form $K$ (extrinsic curvature tensor). The triple $(\mathscr{S},\gamma, K)$ would serve as initial data in the Cauchy problem for Einstein field equations that would determine the Lorentzian metric $g$. The spatial metric $\gamma$ and tensor $K$ must satisfy a set of constraint equations: $$ R^{(3)}=|K|^2−(\mathrm{tr}_\gamma K )^2 + 2\rho , $$ $$ D^i(K_{ij}−\mathrm{tr}_\gamma K \gamma_{ij}) =J_j. $$ where $\rho=\frac{8πG}{c^4}T_{μν}n^μn^ν$ is the matter energy density on $\mathcal{S}$ and $J_j=\frac{8πG}{c^4} T_{μj}n^μ$ the matter momentum vector, with $n^μ$ being the unit normal to $\mathscr{S}$ in $(\mathscr{M},g)$. By looking for Ricci-flat solution we must set $\rho=0$, $J=0$. We further restrict our attention to time-symmetric initial data by requiring that $K_{ij}=0$, meaning that $\mathscr{S}$ would be the slice of zero time $t=0$ of a spacetime $\mathscr{M}$ invariant under replacement $t\to -t$. Vector constraint equations are then satisfied trivially and scalar constraint means that the scalar curvature of 3-metric must be zero. Ignoring for a moment topological “surgery”, if we assume spherical symmetry and asymptotic flatness ($\gamma_{ij}=(1+\frac{M}{2r})^4 \delta_{ij}+O(r^{-2})$), the metric is uniquely specified by its ADM mass $M$ up to the usual diffeomorphism transformations and is simply the slice of Schwarzschild solution at a constant Schwarzschild time, maximally extended into the Einstein–Rosen bridge geometry:

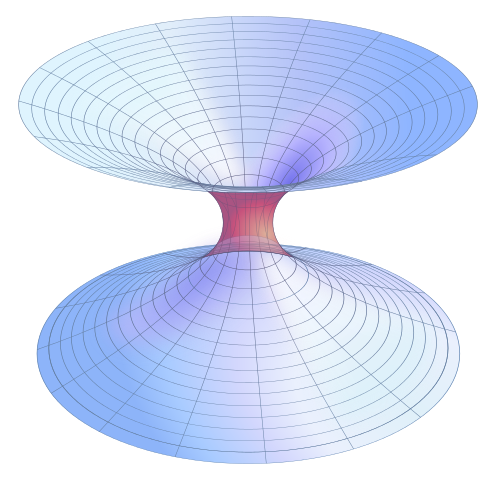
This is an embedding diagram of $\theta=\pi/2$ slice of 3-manifold. For full spatial geometry circles must be spheres $S^2$.

It is on this 3-manifold we now do the surgery, by cutting it along the sphere $r=\mathrm{const}$, removing one of the “halves” and factoring the boundary sphere into $\mathbb{RP}^2$. But this space would still have $\delta$-like singularities at the surface of the cut (geodesic congruence argument from above would still work) unless radial geodesics congruence has zero expansion scalar at the position of the cut. This only happens if we cut along the “throat” of ER bridge:

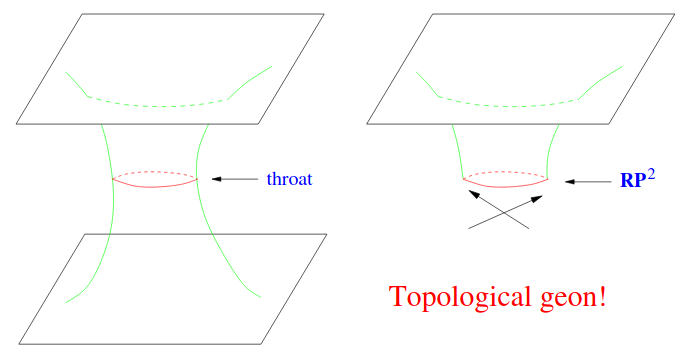
The resulting 3-manifold of course has the same topology as construction in OP (the topology is that of 3 dimensional real projective space $\mathbb{RP}^3$ minus a single point (at spatial infinity)) but no curvature singularities, and could be seen as a quotient of $T=0$ slice of Kruskal–Szekeres maximally extended manifold by a $\mathbb{Z}_2$ factor under involutive isometry of antipodal map: $$ X\to -X,\qquad \theta \to \pi - \theta, \qquad \phi \to \pi +\phi. $$ Since $\mathbb{Z}_2$ is an isometry of initial data, under the evolution by Einstein field equations the same symmetry would persist, and so the whole 4-dimensional spacetime would be the quotient of full Kruskal–Szekeres manifold by that $\mathbb{Z}_2$ isometry. Penrose diagram of the resulting spacetime, called a $\mathbb{RP}^3$ geon, looks like this:

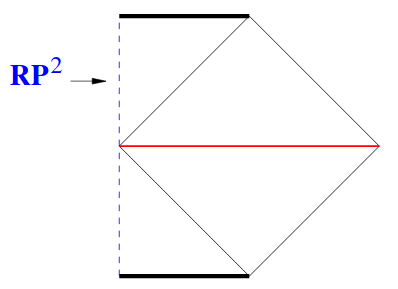
Here, each point of the dashed line corresponds to $\mathbb{RP}^2$ manifold of the cut, while inner points are usual $S^2$. Red line is our $T=0$ initial data slice. We see that $\mathbb{RP}^2$ surface starts at the past singularity, expands but never emerges frim behind the horizon and collapses into the future singularity.

What use does such spacetime have? Since the topology of such geon is present at all times, such solution could not be formed through collapse, but possibly could emerge through quantum pair creation. This spacetime occupies an intermediate position between stationary and dynamical black holes: its time-dependent features are confined behind the horizon. It also serves as a good illustration of topological censorship theorem [$1$]: general relativity does not allow an observer to probe the topology of spacetime (assuming null energy condition): any topological structure collapses too quickly to allow light to traverse it. One could also be interested in quantum mechanical properties of such spacetime: since there is no second exterior region of the Kruskal–Szekeres spacetime, there is no natural way to arrive to thaermality by tracing over the second exterior, and one may wonder what properties the Hawking–Unruh effect in such spacetime would exhibit. It turns out that there is thermal radiation at the usual Hawking temperature but only for a restricted set of observations [$2$].

References

1. Friedman J.L., Schleich K. and Witt D.M. (1993) Topological censorship, Phys. Rev. Lett. 71 1486–9; Erratum 1995 Phys. Rev. Lett. 75 1872, doi:10.1103/PhysRevLett.71.1486, arXiv:gr-qc/9305017.

2. Louko, J. (2010) Geon black holes and quantum field theory, J. Phys. Conf. Ser. Vol. 222. No. 012038, doi:10.1088/1742-6596/222/1/012038, arXiv:1001.0124.

Answer 2

I think this space is a conical defect of order 2 at the center of $\mathbb{R}^3-\{0\}$. This means that any path traversing an angle $2\pi$ at some fixed radius has length $4\pi r$.

This is akin to 2d polar coordinates $ds^2 = 4r^2 d\theta^2 + dr^2$. Indeed the analogous construction is $\mathbb{R}^2$ with the disk $B_2$ removed and the unit circle antipodally identified. The antipodal map is just $\theta \to \theta+\pi$ on the unit circle, and the result is the 2-sheeted radial coordinates whose metric I gave (with $r=1$ the origin of the geometry, and $r<1$ not part of the geometry).

Note that the quotienting procedure does not affect the "bulk" of $\mathbb{R}^3$. Since the Einstein equation is local, a flat metric and vacuum can be chosen there. And at the origin we have $\delta$ function curvature and $\delta$ function matter sourcing this curvature (c.f. conical spacetimes / cosmic strings).

Answer 3

Your space is the tautological real line bundle ${\cal O}$ over ${\mathbb RP}^2$. I'm not sure exactly what structure you're looking for but it seems likely that if you can define it on ${\mathbb RP}^2$ then it will extend in the obvious way to your space. Of course ${\mathbb RP}^2$ comes equipped with a metric as a quotient of $S^2$, so if all you want is a metric, you're done. (To extend to ${\cal O}$ use that a bundle is locally a product and it suffices to define the metric locally.)