1
$\begingroup$

Say I have a simple free source RC as depicted in the image below. The capacitor initially has a charge of Q on its positive plate and at time t=0 the switch is close. I use the mesh current as shown in the diagram and apply KVL to that loop.

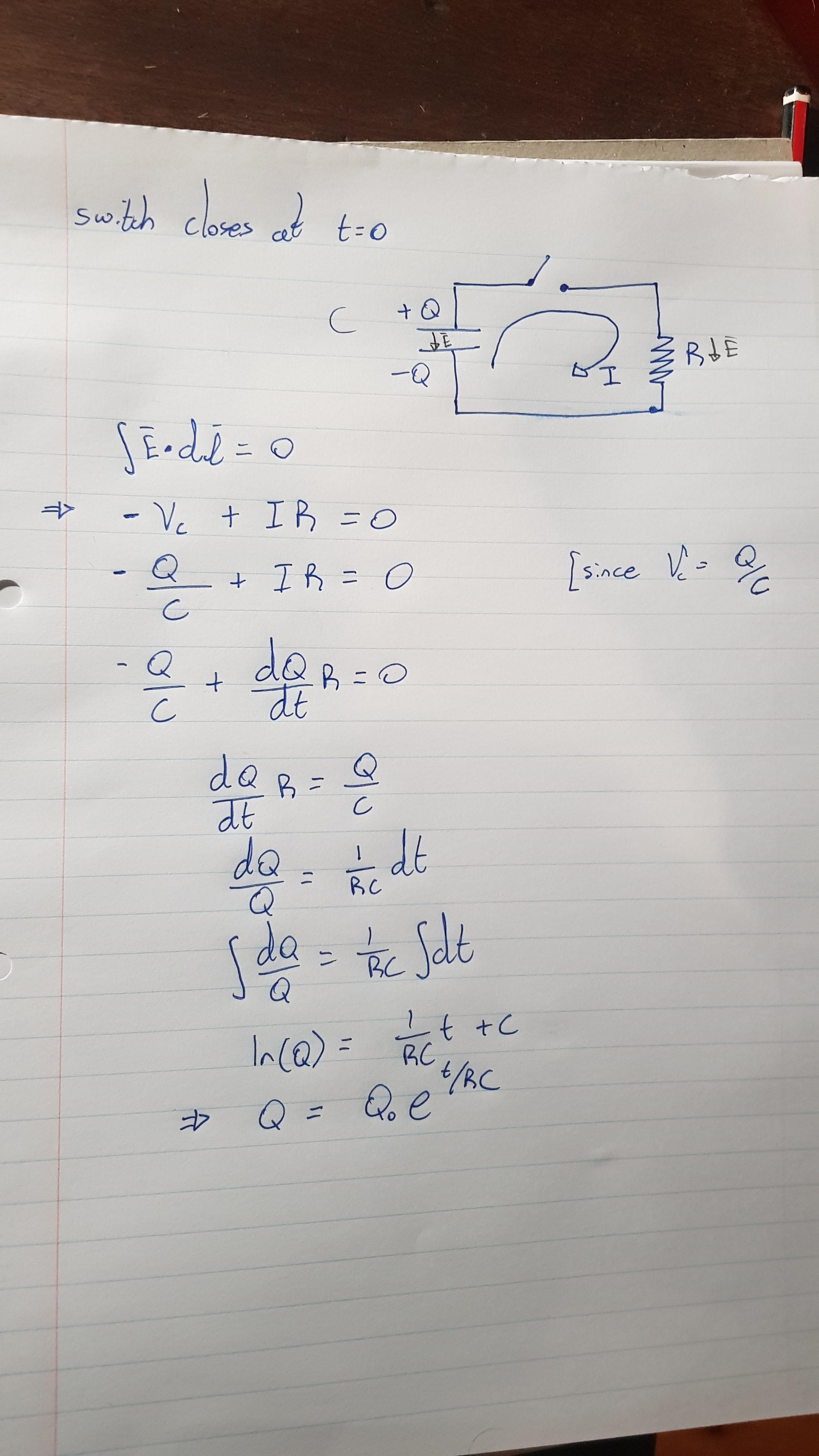Text But then the result I get does not have the minus sign infront of the t in the exponent indicating that the capicator is charging up not discharging. Is this because KVL does not apply here? I have seen some other unconvincing answers to this but none of them apply KVL and I ideally want to understand it in terms of KVL.

Any help on this would be most appreciated.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

K.V.L. does apply here. Your mistake lies in incorrectly substituting the current in the resistor for the time-rate-of-change of charge in the positive-plate of the capacitor. The $I$ in the expression is actually $I(t)$ and it relates to the charge on the positive plate of the capacitor. Notice that as the charge flows around the circuit in the form of current, the charge on the positive plate decreases, so $I(t) = -dQ/dt$. Replace that in your equation and get the right answer.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Ah okay I think I get it. So basically $\frac{dQ}{dt}$ refers to the rate of change of charge on the capacitor. Since the capacitor is discharging it must be a negative quantity. Since I have assumed my current direction to be positive going clockwise, when I perform KVL going in the clockwise direction I have to say that $I(t)=-\frac{dQ}{dt}$ at the resistor and if I dont put that minus in front then I am basically putting my current into the DE as a negative value (since $ \frac{dQ}{dt} <0$) despite the fact that i have assumed clockwise as positive. Right? $\endgroup$
    – SalahTheGoat
    Commented Aug 14, 2020 at 9:18
  • 1
    $\begingroup$ @SalahTheGoat Precisely. "Charge on the capacitor" almost invariably refers to that on the positive plate. $\endgroup$
    – Yejus
    Commented Aug 14, 2020 at 9:27

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.