We define acceleration as $-9.8\frac{m}{s^2}$ for an object that is thrown upwards due to gravity. My question is when the object reaches maximum height at the point where it is about to turn around downwards, will the magnitude of the acceleration still be $9.8\frac{m}{s^2}$ or will it be greater than this as to help it turn around?
$\begingroup$
$\endgroup$
Add a comment
|
7 Answers
$\begingroup$
$\endgroup$
It will be equal to $-9.8\frac{m}{s^2}$. Acceleration due to gravity is constant, as long as it doesn't stray far away from the surface of the Earth.
$\begingroup$
$\endgroup$
2
My question is when the object reaches maximum height at the point where it is about to turn around downwards, will the acceleration still be 9.8 m/s^2 or will it be greater than this as to help it turn around?
It will be smaller, by a small amount. Or a not so small amount if the object goes up several hundred kilometers before falling back to Earth.
Ignoring the Earth's rotation and ignoring the Earth's equatorial bulge, gravitational acceleration at some distance $R$ from the center of the Earth is given by $$g = \frac{GM_\text{Earth}}{R^2}$$ Differentiating with respect to $R$ yields $$\frac{dg}{dr} = -2\frac{GM_\text{Earth}}{R^3} = -\frac{2g}R$$ At 45° latitude, this results in a value of -0.3086 mGal/m, where a mGal is a thousandth of a galileo, a deprecated but widely used unit of acceleration. This is the "free air correction" and is very small over short heights. Using non-deprecated SI units, the free air correction is $-3.086\times10^{-6}\,\text{m/s}^2$ per meter.
answered Aug 6, 2020 at 13:41
-
2$\begingroup$ Downvoter, please explain your downvote. The free air correction of 0.3086 mGal/m is very, very basic concept with regard to understanding gravitational variations near the surface of the Earth. $\endgroup$ Commented Aug 6, 2020 at 14:14
-
$\begingroup$ I don't think the OP is asking about free air correction to the acceleration due to gravity $\endgroup$ Commented Aug 6, 2020 at 15:46
$\begingroup$
$\endgroup$
I'm guessing your confusion is based on a misconception about acceleration and velocity. Acceleration is the rate of change of velocity with time. So as time passes, velocity should change at a certain rate. This rate is the acceleration. So as the ball decelerates, since gravity is acting against it, it's velocity should vary. Hence the velocity of the ball at Max height would be zero, but it's acceleration is still constant. Acceleration can be constant (in the case of gravity) and variable. Variable acceleration simply means that the rate at which velocity changes is not constant i.e it varies. For example a bus driver's acceleration is variable, because he would always have to decelerate at some point.
$\begingroup$
$\endgroup$
0
Acceleration will remain $-9.81 m/s^2$. What changes is the velocity of the ball (think of acceleration as the change in velocity).
Whatever initial velocity you give the ball will keep being subtracted by $9.81 m/s^2$ every second, so this means that the velocity will eventually reach $0$, and then start becoming negative. It is at this point the ball starts to fall back down.
$\begingroup$
$\endgroup$
In my opinion there is a lot of confusion caused by the fact that the formulas derived for the velocity and height by integrating the acceleration $a=-9.81{m\over s^2}$ often omit the integration constants. These result in an initial velocity and an initial height to be taken into account.
Assuming a ball is thrown upwards and leaves the hand at some initial height $h_0$ above the ground, then the ball starts with velocity $v_0$, the velocity decreases more and more (because of the negative acceleration), reaches $0$ at the highest point of the trajectory and then becomes more and more negative:
$$v(t) = v_0 - 9.81{m \over s^2}t$$.
The height is $$ h(t) = h_0 + v_0 t - {1\over 2} 9.81 {m\over s^2} t^2$$
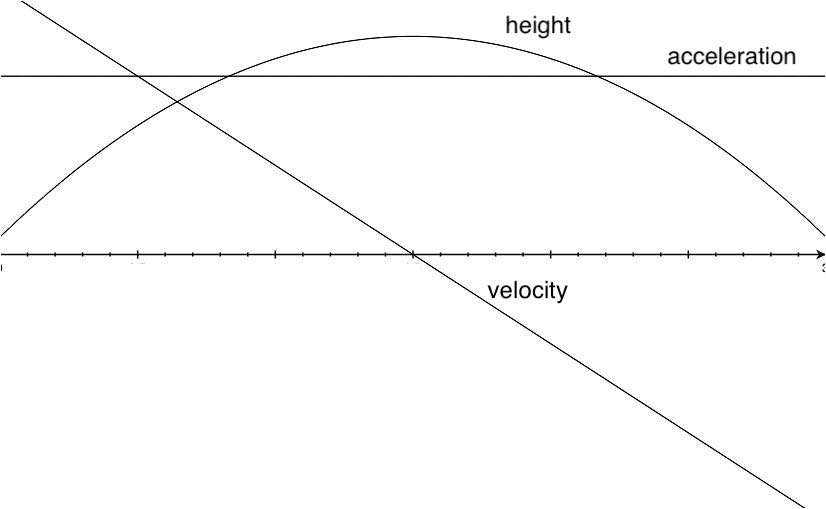
The situation is shown in the following sketch with constant acceleration, linearly decreasing velocity and a parabola for the height:
Of course, the y-axis is not in scale for all three graphs.
$\begingroup$
$\endgroup$
1
The acceleration due to gravity practically does not change near the earth and therefore it is considered constant. If the ball is thrown not high, then it will also accelerate $9.8 \frac{m}{s^2}$ (even astronauts on the ISS are affected by the acceleration of gravity of about $9.2 \frac{m}{s^2}$). How to determine the height to which the ball will rise: equate the kinetic energy at the moment of the throw to potential energy at the moment of the highest point. If you raise the ball vertically upward at speed $v$, then $\dfrac{mv^2}{2} = mgh$, hence $h = \dfrac{v^2}{g}$, for example, if you throw the ball at speed $60 \frac{\text{mile}}{h} = 96.5 \frac{km}{h} = 26.8 \frac{\text{meter}}{s}$ then the ball will take off to a height of $\mathrm{73m = 76.5 \text{yards}}$
-
1$\begingroup$ Please use MathJax for typesetting mathematical expressions. $\endgroup$– user258881Commented Aug 6, 2020 at 17:02
$\begingroup$
$\endgroup$
We know acceleration of freely falling projectile is $\mathrm{g(9.8m/s/s})$.
It seems that you are confused that why an object is still at highest point. We know that acceleration is defined as the change in the speed of an object, not the speed itself.in the process of going from “moving up” to “moving down” though the position of object remains same there is a change in direction of motion of object as its velocity changes from up to down, and that is the effect of the acceleration, which remains consistent throughout its trajectory.
