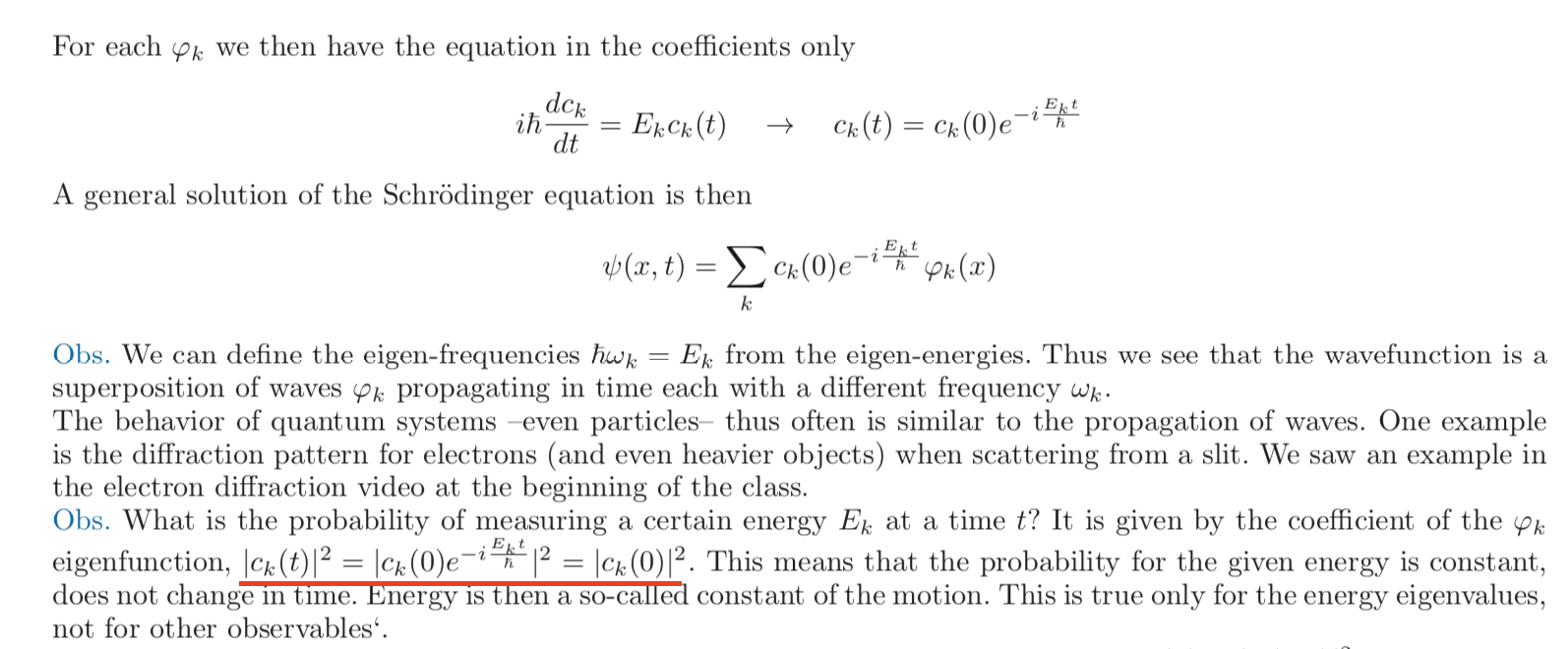
I am trying to make sense of the underlined notes above. I don't understand how did the term $$\Large e^{-2i\frac{E_k t}{\hbar}}$$ got cancelled out? I understand the wave k function times its complex conjugates normalized to $1$, but I am puzzled with the coefficient terms.
Many thanks!
In short, $$ |a\, b|^2 = |a|^2 |b|^2 $$ with $a=c_k(0)$ and $b=e^{-i E_k t/\hbar}$, and $$ |e^{-i E_k t/\hbar}|^2=1. $$ There is no term in $e^{-2i E_k t/\hbar}$: the correct formula for the squared modulus is $|a|^2 = a^*a$, so $$ |e^{-i E_k t/\hbar}|^2 = (e^{-i E_k t/\hbar})^*e^{-i E_k t/\hbar} = e^{+i E_k t/\hbar}e^{-i E_k t/\hbar} = e^{0i} = 1. $$
This is taking the modulus of a complex number, not just "squaring it" in the usual sense:
$$|e^{i\theta}|^2=e^{-i\theta}e^{i\theta}=e^{i\theta-i\theta}=e^0=1.$$
