Can someone provide a physical -- not mathematical -- intuition for the phase in a quantum wavefunction?

Question

I've read every thread on StackExchange (and Quora and reddit...) that I can find about a physical intuition for the phase in the quantum wave function, and I still Just. Don't. Get. It. (Yes, I've seen this thread--didn't help!)

As a jumping off point, I've been watching this terrific visualization of the quantum wave function. According to this video, for a particle in an infinite square well, the "phase" will rotate in the complex plane. Okay...what does that mean physically? Let's focus on just the ground state wave function. If it's not "rotating" in real space (right?), then what exactly is changing to make the phase "rotate"? If I could "see" the wave function with my eyes, what would I see?

I understand the mathematical argument that the phase doesn't matter: the complex exponential cancels out when you calculate the probability distribution, etc.

Maybe my confusion stems from a misunderstanding of what phase even is in quantum mechanics. When I visualize phase, I think of a sine wave and how much it has been shifted to the left or right (relative to some origin). But when I watch that visualization of the ground state wave function, nothing is sliding left or right, the wave isn't going anywhere. So what information does the phase encode here? I'm clearly missing something...

As background, I'm fairly new to quantum physics. I've always wanted to understand it beyond the usual "pop sci" descriptions, so I've been following the MIT OpenCourseware lectures on quantum physics. The physical nature of this phase is just really tripping me up and I've yet to find any explanation anywhere that goes beyond "well it works out in the math." Or is that all it is: just a convenient mathematical bookkeeping trick that physicists keep around because it happens to match observations?

Answer 1

The reason that the overall phase of a wavefunction is hard to gain intuition for is that it's really not real, in the sense that it's just an artifact of a particular choice of quantum formalism and doesn't appear - even purely mathematically - in other formalisms.

In particular, in the density-matrix and projective-space formalisms of quantum mechanics (which are for some purposes more useful than the state-vector formalism), you don't have the freedom to multiply the overall state by an unobservable phase factor. In these formalisms, the states $|\psi\rangle$ and $e^{i \theta} |\psi\rangle$ are represented by literally the exact same mathematical object (a rank-one projection operator or a projective ray, respectively). And energy eigenstates don't pick up an unobservable phase under time evolution, but instead remain completely unchanged.

The reason that you're having a hard time picturing the overall phase of a quantum state is that there is truly nothing to picture.

Answer 2

Visualizing the phase

There are different tricks to visualize the phase. The one you hint at in your answer, amounts to taking the real (or imaginary) part of the wavefunction. For instance, ${\rm Re}[e^{i (k x + \phi_0)}]=\cos(kx + \phi_0)$, which is a sinusoid. The phase determines the location and distance between the crests and troughs. Another trick is to use color -- if you google "complex plane color" you will find many pictures and explanations of this way of looking at this. Yet another visualization is to imagine a little clock face at every point in space, and the position of the minute hand on the clock tracks the phase of the wavefunction. Keep in mind that all of these are simply different representations and are not "what the phase really is"; it's useful to know understand several different ways to visualize the phase, and use the one that is the most convenient or insightful in a particular instance.

Note added, thanks to jgerber's comment: There are some very nice visulations here: vqm.uni-graz.at

Consequence of the phase: interference

The fact that complex probability amplitudes, rather than real-valued probabilities, add in quantum mechanics leads to interference effects. Here we can think about the classic double slit experiment.

Classically, the probability that the particle goes through one slit simply adds to the probability that the particle goes through the other slit. Since probabilities are positive, there are no points with zero probability on the screen which catches the particles.

On the other hand, in quantum mechanics, there are points on the screen where the probability amplitude to go through slit 1 is $a$, and the probability amplitude to go through slit 2 differs by a phase, $e^{i \pi}a=-a$, so the sum of the probability amplitudes is zero and there is zero probability to find the particle at this location. At other locations on the screen, the relative phase will be +1, and the probability amplitudes will add constructively, leading to a "large" probability to find the particle at these locations. In general, the relative phase of the probability amplitude in passing through the two slits is what determines the shape of the interference pattern.

Variations of the phase encode physical information

In general, the phase of the wavefunction $\Psi$ appears in the definition of the "probability current", $\vec{j}\propto i (\Psi^\star \nabla \Psi - \Psi \nabla \Psi^\star)$. A state which is purely real has a vanishing probability current, which is easy to see from the definition. Thus having a non-zero phase allows the state to have a non-zero current, and thus allows the state to change.

We can give this a more direct meaning in some special cases, where the phase of the wavefunction (or more exactly the derivative of the phase with respect to some parameter) encodes an observable quantity. (This is related to the idea of action angle variables in classical mechanics).

This is exactly true when eigenvalue problems for the wave function $\Psi$ of the following form appear \begin{equation} i \frac{\partial \Psi}{\partial z} = \lambda \Psi \end{equation} where $z$ is some parameter and $\lambda$ is an associated eigenvalue. Note that if we write $\Psi=A e^{i \phi}$, where $A$ is a constant, then the above equation can be written \begin{equation} -\frac{\partial \phi}{\partial z} = \lambda \end{equation} If $i \partial/\partial z$ is an operator, and $\lambda$ is a possible measurable outcome of $\lambda$, then the above equation says that the variation of the phase with respect to $z$ gives us the observable value $\lambda$.

There are many examples of type of equation:

• If $z$ is position then $\lambda$ is the momentum; for plane wave states, the derivative of the phase with respect to position is the momentum.
• If $z$ is momentum then $\lambda$ is the position.
• If $z$ is time then $\lambda$ is the energy.
• If $z$ is the azimuthal angle (angle in the $x-y$ plane) then $\lambda$ is the component of the angular momentum parallel to the $z$ axis.

Furthermore, this kind of equation shows up in an approximate form in the WKB approximation. Then, there is a similar type of relation between, say, the derivative of the phase with respect to position, and the momentum, will approximately hold.

Having said that, don't go overboard; the interpretation is a little bit fuzzier for states which are superpositions of eigenstates, and not all observables have the form $i \partial/\partial z$. However, this seed of intuition is useful to keep in mind when considering more complicated observables, which have eigenfunctions more complicated than a plane wave.

TL;DR

• There are many tricks for visualizing complex numbers -- learn several.
• A non-zero phase means the wavefunction is complex, which:
  • allows interference phenomena to occur,
  • means the probability current is non-zero and therefore means the wavefunction can change in time.
• The "more wiggly" the state is with respect to position, the more momentum it has. The "wiggliness" is encoded in the phase. Similar "wiggliness" relationships exist between energy and time, and angle and angular momentum.

Answer 3

While the "devil is in the details", as they say, the concept itself is actually much simpler than you think. There are two related ideas to consider - one is the phase ($\theta$ - just a real number), and the other one is a phase factor ($e^{i\theta}$ - a complex number). Now, I know you asked for "physical — not mathematical — intuition", but what I think you meant is that you're after something that is more tangible than the abstract equations, and not something necessarily physical. So here goes.

The phase $\theta$, in a very general sense, is really just an angle (or, at least that's one way to think about it, and one that happens to work well with the visualization you've posted). This is not quite the same as the overall phase of a wavefunction, but we'll get there.

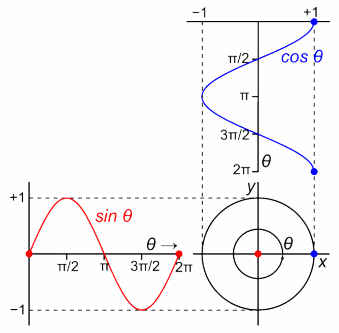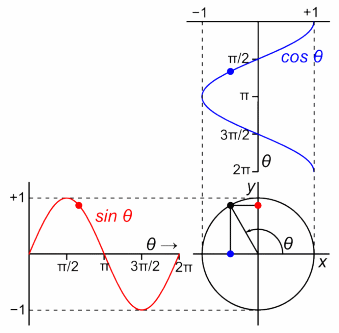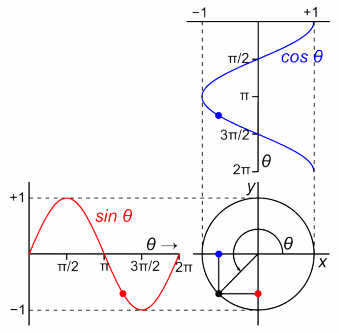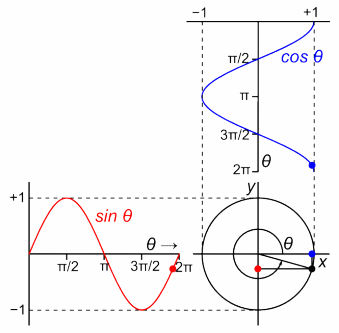
In the image above, you see a point revolving around the unit circle. Here, the angle $\theta$, a.k.a. the phase, increases in what's, by convention, the positive direction. The projection of the point on each axis traces the cosine/sine function. This works the other way around as well, if you combine the cosine and the sine functions to describe the position of a point in 2D space in this particular way, you get a point going around a circle - and that's basically the Euler's formula ($e^{i\theta} = \cos \theta + i \sin \theta$).

Aside:

A complex number is, superficially, not unlike a 2D vector. Roughly speaking, what sets complex numbers apart is their properties (how they "behave", i.e. the kinds of operations possible with them, what they do, etc.) BTW, "real" and "imaginary" are just labels, like x & y, and they don't have any special meaning regarding reality of things.

If you multiply a complex number with a scalar, you can, well, scale it to any size. In other words, by setting the phase (the angle, a.k.a the argument) to some fixed value, and by scaling, you can get any complex number whatsoever (exponential version of the polar form: $z = Ae^{i\theta}$, with $A$ being the magnitude (the scaling factor)).

The more interesting thing is what happens in complex multiplication; more specifically, when you multiply by another complex number that is of unit length (i.e by one that lies on the unit circle), having the angle (argument) $\theta$. Such a multiplication results in a rotation of the first complex number by $\theta$.

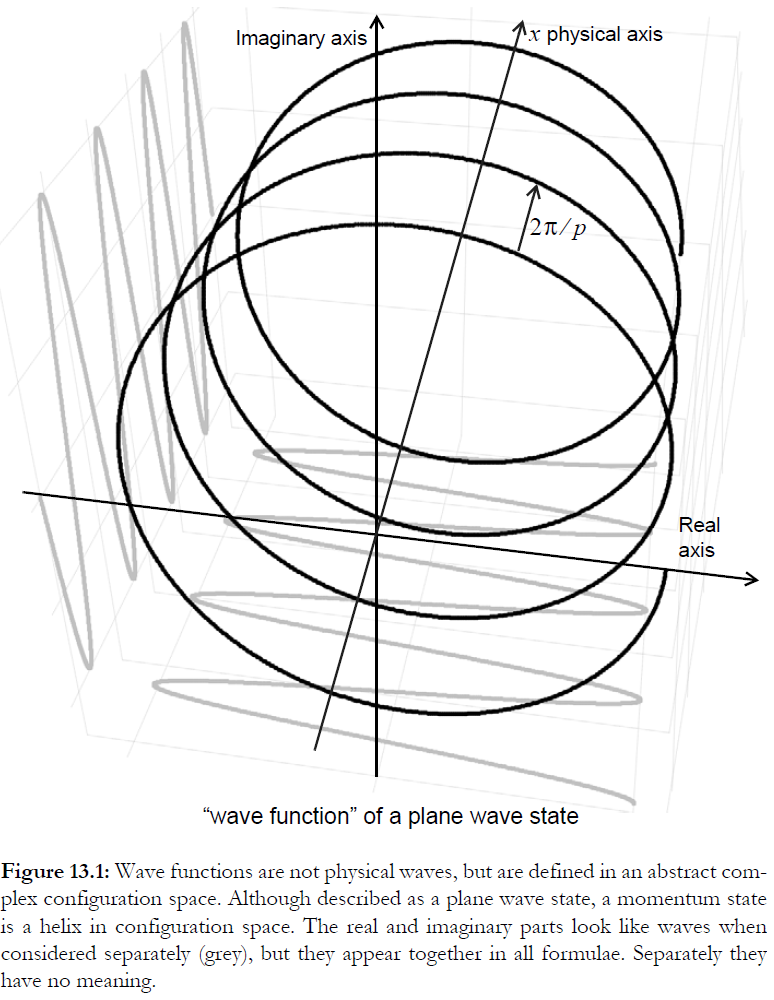
In the visualization you've posted, there's essentially a "string" of complex numbers placed along a line, describing a wavefuntion in 1D space. That is, the "base space" is 1D, but to each point there's a complex number attached.

(Note that there's infinitely many of these arrows, but since that's hard to depict, a selection of representative arrows is shown.)

At this point, there are two kinds of phases we can talk about - the phase of each individual complex number, and the overall phase of the wavefunction. As for the complex numbers themselves, in this case they are all in phase (have the same angle). The phase of the wavefunction is just the overall "rotation" of the whole wavefunction about the central axis; here it is in a different phase:

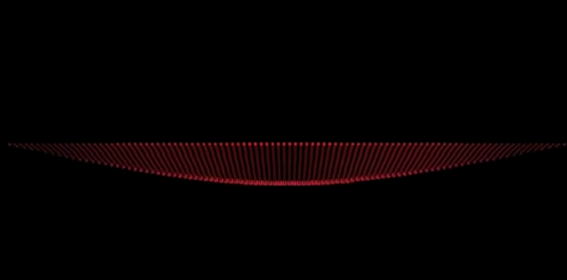
"Let's focus on just the ground state wave function. If it's not "rotating" in real space (right?), then what exactly is changing to make the phase "rotate"? If I could "see" the wave function with my eyes, what would I see?"

It's not rotating in real space. Instead, you can think of it like this: each point in space has a complex value attached to it. You've seen this kind of thing before. E.g., with temperature, each point in space has a single real number attached to it, describing the temperature at that point; and these values change over time. With a gravitational field, each point in space has a vector attached to it. This is the same basic concept, except it's complex numbers, and the way they evolve in time over all that space is "wave-like" in some (formal and informal) sense. To "see" the wavefunction in 3D space "with your own eyes", you would have to have the sensory ability to independently sense/judge/estimate the size of the two components of the complex number at every point throughout 3D space. Imagine that at every point there's a little piece of paper with the complex plane depicted on it, and a little arrow drawn. Or, perhaps, a tiny digital screen displaying a 2D grid with a complex number drawn on it, that can be updated in real-time. The visualization you linked to limits itself to 1D physical space, and essentially uses the other two dimensions to represent the complex plane at each point. It's rotating by having all these arrows (complex numbers) rotate in sync - imagine the little screens updating in sync. For a more complicated situation, there would be some more complicated relationship between the arrows; e.g., the screens could update in some wave-like pattern.

Here's another screenshot from the video you've posted. The blue wavefunction is the superposition of the two others; that just means that the red and green arrows add up (pretty much like vectors) at each point, to form the blue arrows. I'm guessing that you already understand this, but just for clarity, the quantum state is just the blue wavefunctions (there aren't three sets of arrows rotating around, the other two are just shown as the "building blocks" of the blue one).

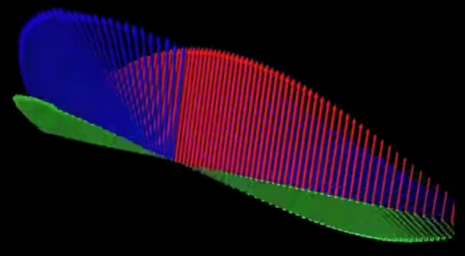
When the narrator says "when the phasors are in phase", he just means that the arrows of the two independent components have, in some region, roughly the same angle and point in the same direction, so they add up to a big arrow pointing in that same direction.

But, the wavefunction itself doesn't give you the probability, its square does. And that's what we're physically interested in. It is represented in the video by the solid green surface (it's the probability associated with the blue (superposed) wavefunction):

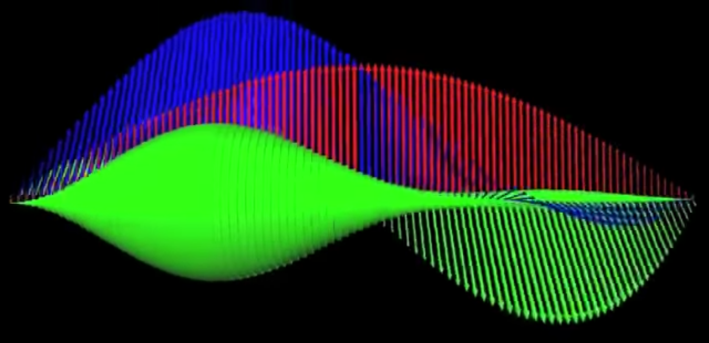
The probability itself at any point is just a real number; this green 3D surface is just a visualization aid. The probability is really the distance of the surface from the central axis (i.e., the radius of the cross section at a given point - that's why it's axially symmetrical).

Now, as you know, the thing about the overall phase (of the wavefunction) is that it doesn't affect the probability. If you freeze time, and rotate the whole thing, the probability distribution (the shape of this solid green surface) doesn't change at all (i.e., the relative relationships stay fixed, you're just spinning the axis, as if everything was glued to a stick that you're spinning between your fingers). That's why absolute phase has no meaning. In a sense, it's just an artifact of the particular mathematical formalism used.

Aside:
Sometimes, a mathematical description of something can be useful and can have desirable properties, but can still give you more than you need. E.g., you can use the mathematical formalism of vectors to describe directions in space - they are arrows, after all. However, many vectors describe the same direction, e.g. $(1, 0, 0)$ and $(5, 0, 0)$, and any $s(1, 0, 0)$, with $s$ being the scaling factor. Then you could say that the $s$ doesn't make a difference for the notion of a direction. But if you need to do things like vector addition (to combine directions for some reason), you have to be careful about $s$ or you may get wrong results - it's what software engineers would call a "leaky abstraction".

The back and forth "sloshing" of the probability distribution happens because the relative relationship of the constituent (red and green) wavefunctions changes in time (because they are rotating at different rates), so the overall shape of the superposed (blue) wavefunction changes, and in turn, so does its square. In other words, it's because the overall phases of the red and green wavefunctions change independently, at different rates, so the shape of the probability distribution dependes on the difference in the overall phases of the two.

"Maybe my confusion stems from a misunderstanding of what phase even is in quantum mechanics. When I visualize phase, I think of a sine wave and how much it has been shifted to the left or right (relative to some origin)."

I'd say that's the core of the issue; the phase is not how much it is shifted left or right, although it can often look like that. The phase is the overall rotation in the sense discussed above. If you disregard time evolution (stop time), you can describe the (overall) phase by picking a value of the wavefunction at some point to serve as a reference. If you then change the phase, you can get the phase difference by comparing the angular displacement of the phasor at the same point. This works well when you have a "wiggly" wavefunction. That way, you can talk about the phase with respect to some reference orientation.

Here's a trickier situation; this is the quantum version of the plane wave, and you can't really tell the difference between an overall phase change (rotation of the overall shape) and wave propagation just by looking at it:

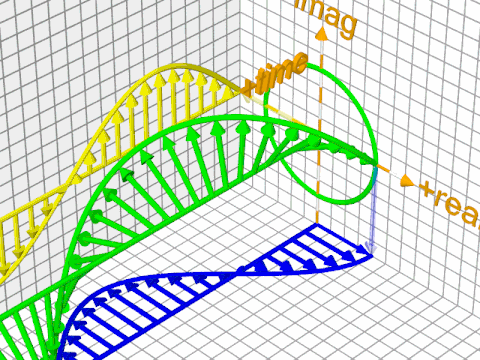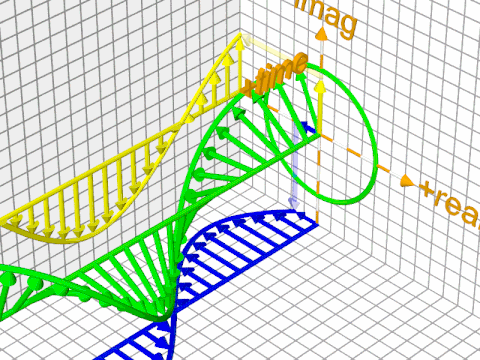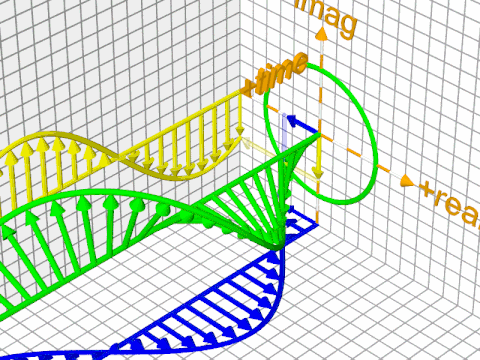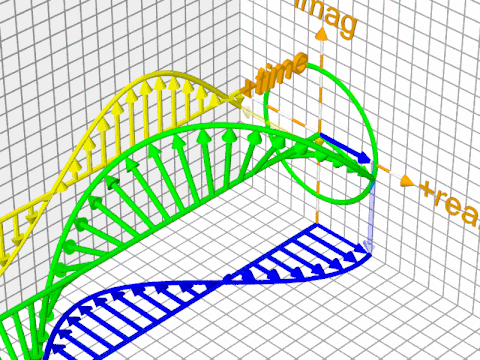
The reason is that its mathematical formula is this (the minus sign is a matter of convention and is not important):

$$\Psi(r, t) = Ae^{i(f(\vec{r}) - g(t))}$$

with, $f(\vec{r})$ giving you the "local" phase of the phasor at point $\vec r$ (its orientation at $t=0$), and $g(t)$ providing a time-based offset from that (both are real-valued functions). The $-g(t)$ essentially works by offsetting the phases of each individual phasor from an "initial" value given by $f(\vec{r})$ for $\vec{r}$ (a particular point in space).

I know this can be confusing, but, again, one way to think about it is that the overall phase change is what happens if you freeze time and rotate the overall wavefunction around its axis, and then "resume playback". It's a mathematical thing - a quirk of the mathematical formalism, rather then something of physical significance. In isolation, a wavefunction with a different phase is technically (mathematically) not the same function, but it is the same physical state that gives you the same probability distribution; there's a redundancy to the mathematical description.

Aside: The more standard version of the above formula is
$$\Psi(r, t) = Ae^{i(\vec{k}\vec{r} - \omega t)}$$

Finally, let me wrap up by coming back to the idea of a phase factor. A change to the overall phase in the above case can be described like this - you just rotate everything by some angle $\alpha$:

$$Ae^{i(f(\vec{r}) - g(t) + \alpha)}$$

Now, due to properties of exponentiation, this is the same as

$$e^{i\alpha}Ae^{i(f(\vec{r}) - g(t))}$$

In other words, rotating everything by $\alpha$ is the same as multiplying by a unit-length complex number $e^{i\alpha}$ (called the phase factor). It's just another way to write things down, one that exploits the properties of complex multiplication.

Answer 4

I love the pictures from the book of Feynman "QED: The Strange Theory of Light and Matter". There, the phase of a wavefunction traveling though the space is depicted as a clock with a moving hand: when the hand makes a full round it corresponds to $2 \pi$ of the complex phase.

This can be easily used to explain the effect of interference: different paths between A and B lead to different travel times and thus to different final positions of the clock hand. Now, the hands obtained from all paths are added like vectors (clock hands look like arrows!). Similar angles add up constructively, opposite angles add up destructively.

The book contains many more examples based on this brilliant visualization idea.

Answer 5

Since you asked for non-mathematical intuition for the phase, I'd say that a classical wave is indeed a good analogy except for the fact that the global phase---as opposed to the relative phase---between two modes is completely indeterminate. Phenomena such as as interference between two modes are fully explained by visualizing them as classical waves that add up or cancel out, with the only caveat that the starting point of the "wiggling" for any of the individual modes isn't possible to determine.

Consider for example a Mach-Zehnder interferometer where only a single photon is fed in at one of the modes. Before it enters the first beam splitter, its (global) phase is unknown. However, as soon as it delocalizes after the first beam splitter, the relative phase between the two arms of the interferometer is well defined and fully accounts for which output mode the photon is likely to re-emerge from.

As a simple exercise, you could try to calculate how interaction-free measurements come about. You'll see that the classical wave picture is perfectly adequate to explain what happens between the two beam splitters, including the precise visualization of the relative phase, but it does not say anything about the global one outside the interferometer.

EDIT:

In a nutshell, if the two arms of the interferometer make up the two modes, you can start with a photon in a single mode only, i.e., the input state could be $e^{i\theta}\mid10\rangle$ where $\theta$ is the (unknowable) global phase. After the first 50/50 beam splitter, we end up with a "delocalized" photon $\frac{1}{\sqrt{2}}\left(\mid10\rangle+e^{i\phi}\mid01\rangle \right)$ where $\phi$ is the relative phase between the two arms. After you pass the second 50/50 beam splitter, you get a probability of $\frac{1}{2} \left( 1+\cos\phi \right)$, hence the "discretized" interference pattern between the two modes modulated by $\phi$. As you can see, nowhere in this discussion has the global phase entered---and that's the main difference with classical waves. (The addition of an obstacle in one of the arms, as in the interaction-free experiment, is just a bonus scenario to better understand the concept of phase.)

Answer 6

The short answer is no. No one can give a physical intuition for the meaning of phase, because it has no physical meaning. To clarify this, it may help to recognise that the wave function of a plane wave state in quantum mechanics is not simply a sin wave. It is a helix in the complex plane.

When the helix rotates (as it does under time evolution), the apparent waves on the real and imaginary parts appear to move like waves. But the rotation does not take place in physical space. It takes place only mathematically in a complex configuration space, and the absolute angular position of the helix has no physical meaning.

Wave functions are better called probability amplitudes. They are not real, but are part of the calculation of probabilities for measurement results. The reason we need them is buried deep in the mathematical foundations of quantum mechanics and is not covered in undergraduate (or even most graduate level) courses of quantum theory, which are concerned with applications not conceptual foundations. They are solutions of the Schrodinger equation, and it can be shown that the general form of the the Schrodinger equation is required in a probability theory for indeterminate processes, as distinct from classical probability theory in which outcomes are determined by unknown variables.

(figure from The Mathematics of Gravity and Quanta)

Answer 7

The short answer is that nobody really knows. To understand what the phase physically means would required a detailed physical understanding of the wave function, that is, of quantum mechanics. Alas, it is not known why point particles such as electrons are described, in a probabilistic manner, by - complex - wave functions. We only understand the phase in terms of the wave function. If that is enough for you then the answers above should be sufficient.

Answer 8

Besides what Andrew already said, I want to add that you cannot expect to have human intuition about every physically meaningful concept or quantity or property. For example, you cannot have human intuition about the energy states of an electron in a hydrogen atom; it just falls out of the mathematics. That said, you can think of phase of a wavefunction as analogous to the phase of the electric field vector in the classical description of a light beam. It is there, and may rotate over time (as in circularly polarized light), and can be observed using detectors that are specifically designed to react differently to different polarization (such as a polarizing filter followed by a light detector).

Answer 9

I understand the mathematical argument that the phase doesn't matter: the complex exponential cancels out when you calculate the probability distribution, etc.

This is not true always, as you've seen in this thread, when the particle is in superposition of two or more wavefunctions.

Maybe my confusion stems from a misunderstanding of what phase even is in quantum mechanics. When I visualize phase, I think of a sine wave and how much it has been shifted to the left or right (relative to some origin).So what information does the phase encode here? I'm clearly missing something...

This shift can be also determined for a $e^{-i(kx -\omega t)}$ which is independent of the imaginary part and it can be used to calculate the group velocity and phase velocity of the wavefunction. And as many other have stated the physical meaning of the phase is not so important, even some wavefunctions are imaginary, the most important part is the physical meaning they represent.

Answer 10

I've been revisiting the same thing for myself right now, and stumbled upon just a great (and very short!) mathematical explanation, which made a perfect sense to me.

The even shorter explanation is this:

We must keep the total probability to be 100%
all the time.

All the time.

Physically it means that for any given initial property of a particle (say, it's orientation along physical X, or Y axis, or any other property) - we can predict whether this is going to change in any way, and calculate exact (!!) probability of that to happen.

How? We treat any property of a particle as an abstract vector, located in an abstract vector space: this vector always has a fixed length, and it can be rotated.

What?.. Why (physically) - ??

First reason is, because any physical property can exist in a number of options. Usually there's just 2: "up/down" (as in quantum spin), "clockwise/counterclockwise" (as in polarisation), etc.; but there might be many, even nearly infinite:

• this, or that, or any other position of a particle on a continuous screen (say, in a double slit experiment);
• this, or that, or any other position of a particle in our continuous space-time (say, on its way to a screen, or to the slits, or any other way);
• this, or that, or any other orientation (axis) of a particle in our continuous space-time (say, not just "up/down", but any arbitrary - relative to former), etc.

Second, and most important reason is: these options cannot be realized simultaneously - and that's that! The vector form on itself - is just a convenient way to express such "not simultaneousness" mathematically: we merely assign to each of the 2 (or whatever) of our options its own orthogonal axis (meaning that it is perpendicular to any other axis, along which our state-vector's coordinates are defined; there, again, might be any number of axis - in a realm of mathematics), and then we just measure the length of our state-vector along these option-axis: that length (its square really, which in fact is just a pure Pythagorean theorem) would be our probability.

So, wavefunctions just live in such continuous vector spaces with many perpendicular axis, evolving (more often by rotation) among one of these "not simultaneous" axis with its phase.

Ultimately, phase is just an artifact of a wavefunction, rotating in that vector space; and the nature of this rotation purely depends on which property of a particle you've chosen to analyze: it might represent a change in space-time coordinates, or a change in spatial orientation (axis), or whatnot; therefore, a phase - is just a rate of that change.

But why do we need to express these discrete, countable options in such continuous way?

Short answer is, because it is an experimental fact: we can calculate exact (!!) probability for any arbitrary option by just knowing what option our physical system is in right now. Even if it is uncertain.

Say, if a particle is "up" right now - we can calculate and find that it is definitely (100%) going to be still "up" if we will measure it in the same "up/down" direction; but, if we will choose to measure that same "up" particle in another direction (say, "left/right", or if the particle was "uncertain") - we will find an even (50/50 - for the set with 2 options) probability.

But more often probability between 2 or more options is not even - if we were to choose an arbitrary direction of measurement; or, due to interference (like in a double slit) - which is literally wavefuncion overlapping with its own different parts.

But either way, probability must always add up to 100%.

All the time,
no matter the arbitrariness.

Again: it is just an experimental fact, which turned out to be best described in a vector space; and we need that vector space to be continuous exactly for its predictive power - not just for "up/down" or "left/right", but any arbitrary angle: that is especially clear in the case of physical orientation (axis) in space-time.

Long answer is: this is also more commonly known as Heisenberg's uncertainty principle.

---

EDIT: also, in my own research, there was another very common physical example for the meaning of a phase - the fact that it is somehow related to a charge of a particle:

In quantum mechanics the scalar field is equivalent to a phase shift in the wavefunction of the charged particle

It has deep physical meaning, for example as an explanation to why do particles annihilate with their antiparticles (since latter are having their phase rotating in opposite direction).

And, I do not know much else about that :) in fact, I revisited the whole thing exactly because I've been researching charges.

But, I do believe that the same argument as above still applies: something is changing as time goes on.

The fair objection then might be: if we choose our "property" freely, and a phase merely shows a change in that property, does that mean that change of any property is related to charge - ?..

Honestly, I do not know: I can bet that a change in "spatial/momentum" property might be just luckily coincident with charge; and yet, there might be other momenta that partice can have (say, angular momentum), which can have whole different set of "change rates" of its own.

But ultimately, there's a "time/energy" property relation for any particle:

$$\Delta E \Delta t \geq \frac{\hbar}{2}$$

$-$ where $E$ is a total energy: I bet, that is the right property to look at "change rates" of, if one is interested in phase-charge relation.

Answer 11

That's because the absolute phase is not physically meaningful.

It only acquires any meaning when compared to a reference, another path that also has a phase, for instance another path from a beamsplitter, or reflected from a boundary. Then the two paths can interfere, constructively or destructively, as dictated by their phase difference.

Answer 12

tl;dr– You can think of how sounds can combine to form louder sounds or cancel out. Whether sounds add or cancel depends on their phase.

---

Phase is a more general mathematical concept. It comes up in all sorts of stuff beyond quantum-mechanics, e.g. sounds, electric-current, and radio-waves. Probably easier to understand the concept itself outside of quantum-mechanics.

---

Example: Phase determines if sound-waves add or cancel.

Say you set up a microphone that listens for sounds and then replays them at the same volume on a speaker. Does this result in louder sounds or noise-cancellation?

Depends on the phase!:

• If the sound-waves are lined up to peak together, the sounds'll add.

• If the sound-waves have their peaks in opposition, then they'll tend to cancel out. Which is how active noise-cancellation works.

---

Some observations...

Contrary to the above descriptions, sound-waves don't actually have phases in an absolute sense. We can just talk about sound-waves as though they had phases as one mode of thinking about them.

If we do choose to think of two sets of sound-waves as having phases, then the big thing is that we correctly consider their phases relative to each other. But if we choose to say that one's in the default-phase while the other's in an anti-phase or something, well, that'd be pretty arbitrary.

Sound-waves were probably the simplest example. Once you feel comfortable with phases with respect to them, the next analogy to consider might be alternating electric current (AC electricity), e.g. in three-phase electric systems.

As for math, probably best to start by learning about the Fourier transform. The gist is to redescribe things in repeating signals of different frequencies (often described as the frequency domain). In plain English, this basically just means that instead of directly describing the value of a function, we instead describe the function as a bunch of waves that add up to that value. For example, instead of describing a sound in terms of its pressure at any given moment at each point in space, we might describe it as a sum of various sound-waves with their own frequencies and amplitudes.

As for the connection to quantum-mechanics, it'd all go back to the double-slit experiment:

The experiment belongs to a general class of "double path" experiments, in which a wave is split into two separate waves that later combine into a single wave. Changes in the path lengths of both waves result in a phase shift, creating an interference pattern.

—"Double-slit experiment", Wikipedia

One may've expected light-sources to add up, sorta like how one might assume that adding more sound always creates a louder noise (rather than noise-cancellation), but historically they were surprised by the interference pattern that heavily implicated wave mechanics, with a connection to the concept of phase as in the quote above.

---

In short..

When combined, waves can add or cancel. They add when they largely share the same phase, while they can cancel if they're anti-phased.

So "phase" describes how a wave'll interact with others when combined.

Answer 13

The OP clarified:

It's been a while since I've read QED, but I do recall discussion of 'little clocks'. I'm just having trouble envisioning what those little clocks actually are. I have seen phase as color visualizations. They're very cool to watch, but I don't get what's physically changing in the wavefunction, if that makes sense.

I liked Feynman's way of explaining that. I don't have the book here but as I remember he explains that the dials are just a way to describe they behavior of particles in the quantum world. There is no way to say what those little clocks "actually are". Feynman deliberately chose a very unphysical representation. In the video that the OP linked to these arrows are turning. It is difficult to make such movies, and I find it hard to watch them.

In the same way one cannot say what is "physically changing" in the color visualizations. It is much easier to make such movies and they can also help create intuitions about the behavior of wave functions.

Both these visualisations represent the phase factor $e^{iEt/\hbar}$ but that is also just a mathematical description of what is observed: diffraction and interference. The empirical observation is that particles behave like waves at the quantum scale, can interfere destructively. We are not used to that in our everyday experience. That is why quantum mechanics is weird.

Answer 14

Consider Zeno's paradoxes in light of the Einstein's thought experiment of the two lightnings hitting a train. The "paradoxes" show that we can't really talk about objects outside the context of observation and the problems that arise if we postulate existence of object outside of an observer's frame of reference and thus not subjected to the mechanics of perception.

To understand perceived objects we must understand how perception works because anything we think about as a real object is included in the scope of perception, is perceived and is absolutely subjected to it's mechanics.

Zeno's paradoxes are given in terms of what is seen or otherwise perceived, such as a race seen by the eye. When we observe a race where some fast moving object passes by [overtakes] a slower moving object at some point we can't tell who is ahead but after that point the faster moving object is perceived to be ahead, measurement is a matter of precision but is likewise an act of observation.

As I understand it, the wave function is a predictive model describing a to-be-perceived collapse of the function rather than an object's non perceived state.