In the Maxwell's demon thought experiment, initially, the gases in both boxes have the same temperature. The devil uses the door in the middle to allow the fast (hot) molecules on the left to pass to the right. But, we said the gases in both boxes have the same temperature. So, the right box is not completely hot. There exist still cold gas molecules on the right. But according to the thought experiment, the cold gas molecules are all collected on the left side of the chamber. Is this a contradiction in the paradox? How do slow molecules move as fast as other hot gas molecules?
$\begingroup$
$\endgroup$
3
-
4$\begingroup$ The demon can also open the door to make the cold gas molecules go to the left. Or, the right side has the cold gas molecules from the right, plus the hot gas molecules from the right, plus the hot gas molecules from the left, and the left only has the cold gas molecules from the left. $\endgroup$– Criticizing Israel not allowedCommented Jul 21, 2020 at 19:44
-
1$\begingroup$ Maxwell's demon enjoys breaking the laws of thermodynamics, but is unwilling to break Gay Lussac's law, and is going to have extra trouble keeping the hot side hot and the cold side cold. The demon can open the trap door both directions and let the cool gas move left. $\endgroup$– user662852Commented Jul 21, 2020 at 20:53
-
1$\begingroup$ They're A) living in a segregated ghetto, kept down by The Man! B) huddling together for warmth, C) planning an invasion of the Warm Side, D) all of the above, E) none of the above. $\endgroup$– Bob Jarvis - Слава УкраїніCommented Jul 21, 2020 at 22:00
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
2
Individual gas molecules are neither cold nor hot: They have kinetic energy.
The absolute temperature of a gas is proportional to the average of the kinetic energies of its molecules, and what's important here, is that the kinetic energies are not all the same. There is a statistical distribution of different energies in any given body of gas. Even so when the gas is all one "temperature."
https://en.wikipedia.org/wiki/Maxwell%E2%80%93Boltzmann_statistics
-
1$\begingroup$ Not to nit pick - just testing my own understanding. Isn't it the average of their energies calculated using the velocity with respect to their center of mass? Because their kinetic energy depends on the reference frame you are using. $\endgroup$ Commented Jul 21, 2020 at 7:44
-
4$\begingroup$ @MikeWise The frame is the frame where the center of mass of the ensemble of molecules it at rest. A box with gas sitting on a table, The kinetic energy of the box if in motion does not contribute to the kinetic energies averaged of the ensemble. $\endgroup$– anna vCommented Jul 21, 2020 at 8:58
$\begingroup$
$\endgroup$
3
Suppose, initially the gas on both sides has the temperature $T_0 = 273K$. In thermal equilibrium the velocity distribution of each side is given by the Maxwell-Bolzmann distribution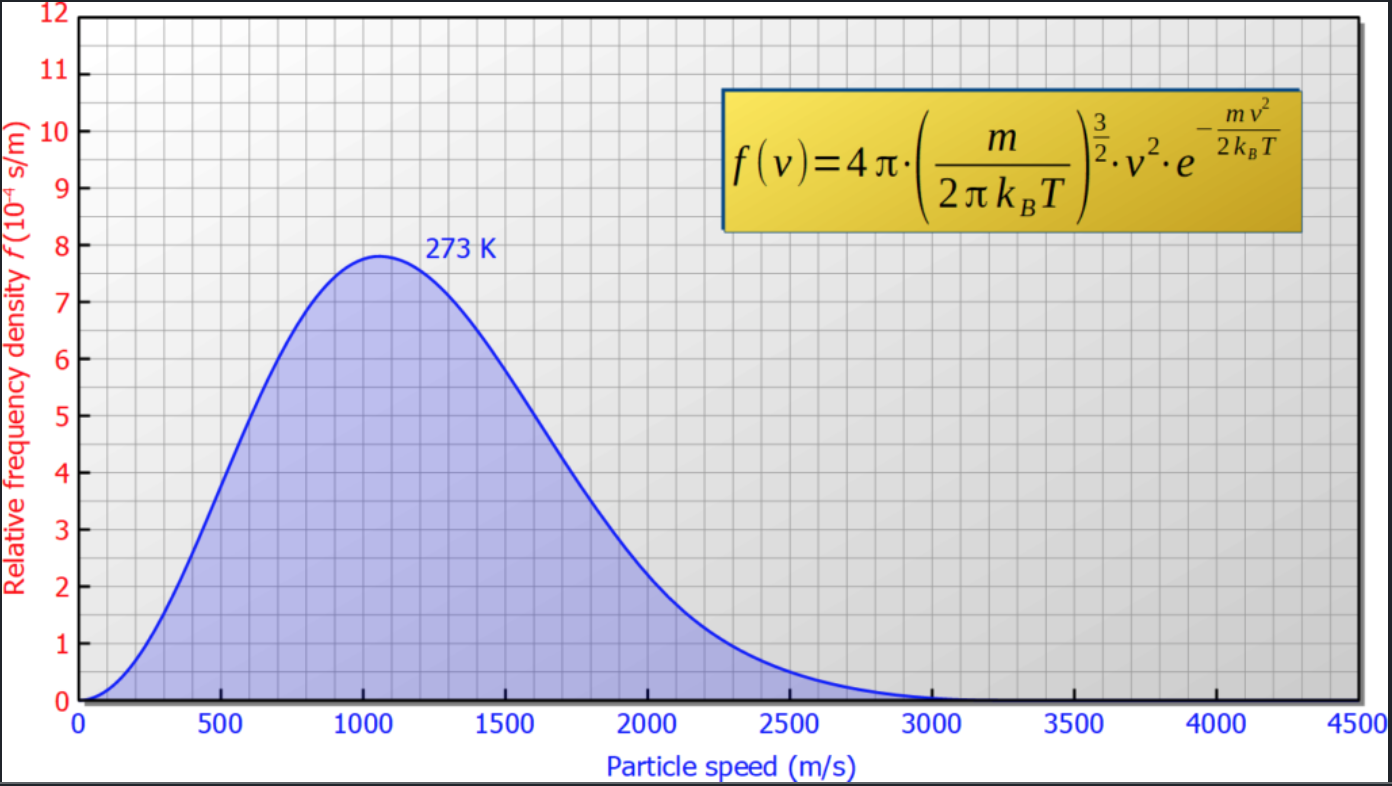 
The average velocity is around $\bar v = 1500m/s$.
Now, suppose that only the particles with veclocity $v>2500m/s$ are allowed to pass from the left to the right chamber. Since the average velocity of these particles is higher than the overall average velocity $\bar v$, these particles carry energy from the left chamber into the right chamber. Thus, after rethermalisation the temperature on the left side has reduced, say to $T_1^{(left)}=270K$, while the temperature on the right side has increased, say to $T_1^{(right)}=276K$.
While this concept is forbidden in the context of Maxwell's devil, it is effectively at work in ultra-cold atoms labs around the world: Trapping atoms in either magnetic or an optical dipole potentials, and removing only the high energetic atoms of the trap, we reduce the temperature of the remaining atoms. Another example for this so called evaporation cooling is your coffee mug.
-
3$\begingroup$ please note that it is for isolated systems that the second law would seem violated. Your examples are not isolated systems., the coffee in the mug is not split in two, it comes into contact with a different temperature system. $\endgroup$– anna vCommented Jul 21, 2020 at 9:05
-
$\begingroup$ My intention was to keep the text simple. The concepts of thermodynamics are of course true in every system. $\endgroup$– NotMeCommented Jul 22, 2020 at 4:52
-
$\begingroup$ Maxwell's Demon, if implemented in the real world, can in fact separate hot molecules from cold ones. It's just that, as with the optical dipole trap, the effort of doing so increases entropy more than the separation decreases it. $\endgroup$– MarkCommented Jul 22, 2020 at 21:54