General Relativity: is there a better way to get spherical coordinates?

Question

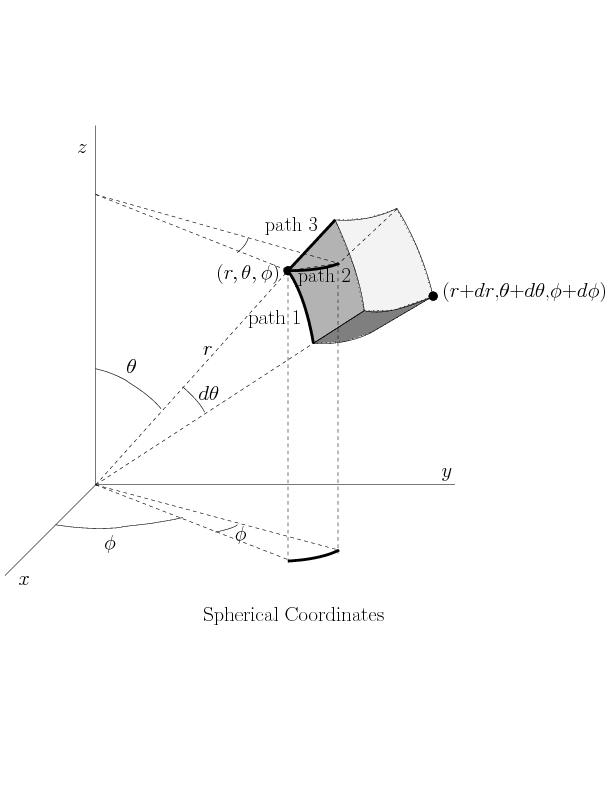
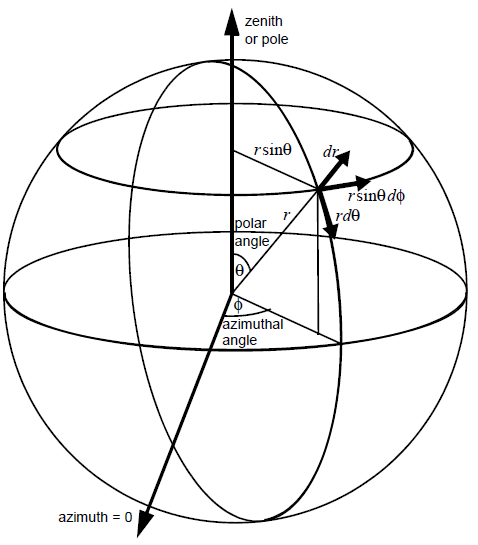
We know that (see this wikipedia page) in the metric of Minkowski spacetime: $$ds^2=(dt)^2-(dx)^2-(dy)^2-(dz)^2 \tag{1}$$ and we also know that in spherical coordinates this same metric becomes: $$ds^2=(dt)^2-(dr)^2-r^2(d\theta)^2-r^2\sin^2{\theta}(d\phi)^2 \tag{2}$$ Let's prove this last statement:
We have that: $$\begin{cases}t=t \\ x=r\sin{\theta}\cos{\phi} \\ y=r\sin{\theta}\sin{\phi} \\ z=r\cos{\theta}\end{cases}$$ we can think of $x,y,z$ as functions of $r,\theta,\phi$; so we get: $$dx=\sin{\theta}\cos{\phi}dr+r\cos{\theta}\cos{\phi}d\theta-r\sin{\theta}\sin{\phi}d\phi$$ and so on for $dy,dz$; then we can square to get $(dx)^2,(dy)^2,(dz)^2$ written in terms of $(dr)^2,(d\theta)^2,(d\phi)^2$. Now we can put our findings back into equation (1) and if all goes right we should find equation (2).

However I didn't get to the end of this calculation because the algebra gradually becomes unbearable, especially when you get to the squaring part, where terms with mixed differentials start to pop out. However seems to me that this method should work out fine.

My questions are: This method will lead to the correct solution (2)? And even if this method is indeed correct: is there a better method to demonstrate (2) from (1)? Where better here means simply less algebra.

Answer 1

Yes! There's a way more simple method of converting the metric into spherical co-ordinates. In cartesian co-ordinates, the expression of the metric is of the form

$$\mathrm ds^2=-c^2\mathrm dt^2+(\text{infinitesimal displacement})^2\tag{1}$$

In cartesian co-ordinates,

$$\text{infinitesimal displacement}=\sqrt{\mathrm dx^2+\mathrm dy^2+\mathrm dz^2}$$

So now, our task is to find such an infinitesimal displacementin spherical co-ordinates. This is a purely mathematical task. Let's start of with a figure.

^{Image source}

In the above image, all the three paths are mutually perpendicular/orthogonal, so the net displacement will just be the

$$\text{infinitesimal displacement}=\sqrt{(\text{path 1})^2+(\text{path 2})^2+(\text{path 3})^2}\tag{2}$$

But it's easy to see that

\begin{align} \text{path 1}&=r\mathrm\: d \theta\\ \text{path 2}&=r\sin \theta \: \mathrm d\phi\\ \text{path 3}&=\mathrm dr \end{align}

And voila, substitute the above expressions into equation $(2)$ and subsequently into equation $(1)$ to get the desired result.

Answer 2

$\newcommand{\vect}[3]{\left[ \begin{array}{c} #1 \\ #2 \\ #3 \end{array}\right]} \newcommand{\mat}[9]{\left[ \begin{array}{ccc} #1 & #2 & #3 \\ #4 & #5 & #6 \\ #7 & #8 & #9 \end{array}\right]} \def\st{\sin\th} \def\ct{\cos\th} \def\sf{\sin\f} \def\cf{\cos\f} \def\f{\varphi} \def\th{\theta} \def\VX{{\bf X}} \def\VY{{\bf Y}} \def\MM{{\bf M}} \def\MD{{\bf D}} \def\id{\mathbb{I}}$The other answers provide good geometric intuition. Here we give one way to do the brute force work in an organized fashion. Algebra is good for the soul!

We have \begin{align*} d\VX &= \vect{dx}{dy}{dz} \\ &= \mat{\st\cf}{r\ct\cf}{-r\st\sf} {\st\sf}{r\ct\sf}{r\st\cf} {\ct}{-r\st}{0} \vect{dr}{d\th}{d\f} \\ &= \underbrace{\mat{\st\cf}{\ct\cf}{-\sf} {\st\sf}{\ct\sf}{\cf} {\ct}{-\st}{0}}_\MM \underbrace{\mat{1}{0}{0} {0}{r}{0} {0}{0}{r\st}}_\MD \underbrace{\vect{dr}{d\th}{d\f}}_{d\VY}. \end{align*} Thus, \begin{align*} dx^2+dy^2+dz^2 &= d\VX^T d\VX \\ &= d\VY^T \MD \MM^T \MM \MD d\VY. \end{align*} The krux of the calculation is then in finding $\MM^T \MM$. By inspection, the columns of $\MM$ are orthogonal. By further inspection, the columns are orthonormal. Thus, \begin{align*} dx^2+dy^2+dz^2 &= d\VY^T \MD \id \MD d\VY \\ &= d\VY^T \MD^2 d\VY \\ &= dr^2+r^2d\th^2+r^2\st^2d\f^2. \end{align*}

Answer 3

There is no time transformation and you can read the metric directly from a diagram. Small coordinate changes $dr$, $d\theta$, $d\phi $ correspond to displacement vectors with magnitudes $dr$, $rd\theta$, $r\sin\theta d\phi $. This is an orthogonal triad, so you can write down your eq $(2)$ immediately

Answer 4

I understand $(2)$ literally geometric. At any point of a spherical surface of radius r like the earth surface, it is possible to get 3 perpendicular small vectors. One that is vertical to the local surface: $\Delta r$. One locally parallel to North-South direction: $r\Delta \theta$. And finally one parallel to the West-East direction: $r sin(\theta)\Delta \phi$.

Any other direction can be obtained by Pythagoras from that $3$ orthogonal base vectors.

But the algebric brute force method certainly works.

Answer 5

you can obtain it like this with:

$$\vec{R}=\begin{bmatrix} t \\ x \\ y \\ z \\ \end{bmatrix}=\left[ \begin {array}{c} t\\ r\sin \left( \theta \right) \cos \left( \phi \right) \\ r\sin \left( \theta \right) \sin \left( \phi \right) \\ r\cos \left( \theta \right) \end {array} \right] $$

and

$$\vec{q}=\left[ \begin {array}{c} t\\ r\\ \theta\\ \phi\end {array} \right] \quad, \vec{dq}=\left[ \begin {array}{c} dt\\ dr\\ d\theta\\ d\phi\end {array} \right] $$

the metric $G$ is:

$$G=J^T\,\eta\,J$$

where $$\eta=\left[ \begin {array}{cccc} 1&0&0&0\\ 0&-1&0&0 \\ 0&0&-1&0\\ 0&0&0&-1\end {array} \right] $$ the signature Matrix

and $$J=\frac{\partial \vec R}{\partial \vec q}=\left[ \begin {array}{cccc} 1&0&0&0\\ 0&\sin \left( \theta \right) \cos \left( \phi \right) &r\cos \left( \theta \right) \cos \left( \phi \right) &-r\sin \left( \theta \right) \sin \left( \phi \right) \\ 0&\sin \left( \theta \right) \sin \left( \phi \right) &r\cos \left( \theta \right) \sin \left( \phi \right) &r\sin \left( \theta \right) \cos \left( \phi \right) \\ 0&\cos \left( \theta \right) &-r\sin \left( \theta \right) &0\end {array} \right] $$

thus:

$$G=\left[ \begin {array}{cccc} 1&0&0&0\\ 0&-1&0&0 \\ 0&0&-{r}^{2}&0\\ 0&0&0&-{r}^{2} \left( \sin \left( \theta \right) \right) ^{2}\end {array} \right] $$

and the line element

$$ds^2=\vec{dq}^T\,G\,\vec{dq}={{\it dt}}^{2}-{{\it dr}}^{2}-{d\theta }^{2}{r}^{2}-{d\phi }^{2}{r}^{2 } \left( \sin \left( \theta \right) \right) ^{2} $$

Answer 6

When I do these calculations, I do not expand the squares but do book-keeping and simplifications in my head.

We get $$ \begin{cases} dx = \color{red}{dr \sin\theta \cos\phi} \color{green}{+ r \cos\theta\,d\theta \cos\phi} \color{blue}{- r \sin\theta \sin\phi\, d\phi} \\ dy = \color{red}{dr \sin\theta \sin\phi} \color{green}{+ r \cos\theta\,d\theta \sin\phi} \color{blue}{+ r \sin\theta \cos\phi \, d\phi} \\ dz = \color{red}{dr \cos\theta} \color{green}{- r \sin\theta \, d\theta} \end{cases} $$

When you calculate $dx^2+dy^2+dz^2$ the $\color{red}{\text{red}}$ parts squared will sum to $\color{red}{dr^2},$ the $\color{green}{\text{green}}$ parts squared to $\color{green}{r^2 \, d\theta^2},$ and the $\color{blue}{\text{blue}}$ parts squared to $\color{blue}{r^2 \sin^2\theta \, d\phi^2}.$ Then, checking the cross-terms, e.g. $\color{red}{\text{red}}$-$\color{green}{\text{green}}$ one can see that they all cancel.

Thus, $dx^2+dy^2+dz^2 = dr^2 + r^2 \, d\theta^2 + r^2 \sin^2\theta \, d\phi^2.$

If you can not handle the calculations in your head, take at least one kind of terms at a time:

$\color{red}{\text{red squared}}$: $$ (dr \sin\theta \cos\phi)^2 + (dr \sin\theta \sin\phi)^2 + (dr \cos\theta)^2 \\ = dr^2 \sin^2\theta \cos^2\phi + dr^2 \sin^2\theta \sin^2\phi + dr^2 \cos^2\theta \\ = dr^2 \sin^2\theta + dr^2 \cos^2\theta \\ = dr^2 $$

$\color{red}{\text{red}}\text{-}\color{green}{\text{green}}\text{ cross-term}$: $$ 2 \, dr \sin\theta \cos\phi \, r \cos\theta \, d\theta \cos\phi + 2 \, dr \sin\theta \sin\phi \, r \cos\theta \, d\theta \sin\phi - 2 \, dr \cos\theta \, r \sin\theta \, d\theta \\ = 2 \, r \, dr \, d\theta \sin\theta \cos\theta \cos^2\phi + 2 \, r \, dr \, d\theta \sin\theta \cos\theta \sin^2\phi - 2 \, r \, dr \, d\theta \sin\theta \cos\theta \\ = 2 \, r \, dr \, d\theta \sin\theta \cos\theta - 2 \, r \, dr \, d\theta \sin\theta \cos\theta \\ = 0. $$ and so on.