0
$\begingroup$

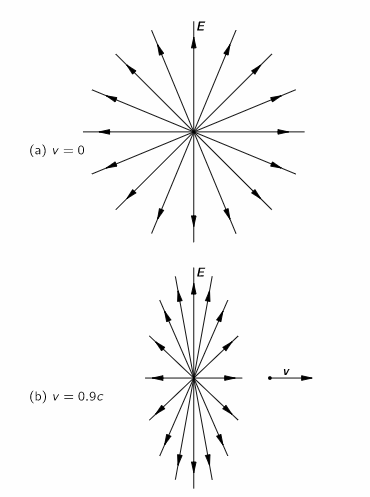
Between two stationary charges, Newton's third law holds. But what if one of the charge is moving? Like, in moving charge, electric field is different with the field generated by stationary charge.

enter image description here

So let's think there are two charges. Charge with $q_1$ moves toward to another charge with $q_2$, that is stationary. The relative velocity is $v$. In this condition, what would be the force between two charges? Will the force be different with two stationary charges? Or will the force be same? Will the Newton's third law apply?

Improve this question
$\endgroup$
1

1 Answer 1

Reset to default
4
$\begingroup$

Newton's third law does not apply directly to moving electric charges. Newton's third law is derived from conservation of linear momentum. For moving charges, the changing momentum in the electromagnetic field has to be taken into account, so $\bf F_{21}=-F_{12}$ does not always apply for the moving charges

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks for your answer! Can you give a formula of $F_{12}$ and $F_{21}$? $\endgroup$
    – littlegiant
    Commented Jul 17, 2020 at 9:48
  • 1
    $\begingroup$ ${\bf F_{12}}= \frac{q_1q_2[{\bf r_{12}}+{\bf v_1\times(v_2\times r_{12})}]} {\gamma_2^2[{\bf r_{12}}^2-({\bf v_2\times r_{12}})^2]^{\frac{3}{2}}}$ $\endgroup$
    – Jerrold Franklin
    Commented Jul 17, 2020 at 14:18
  • $\begingroup$ Interchanging 1 and 2 does not give $\bf F_{21}= -F_{12}$. $\endgroup$
    – Jerrold Franklin
    Commented Jul 17, 2020 at 14:19

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.