Why do we use energy conservation to obtain maximum displacement of the block in spring block system whereas for displacement of the mean position(equilibrium position) we use $F_{net} = 0$ on the block?
The below image describes the actual problem
$\begingroup$
$\endgroup$
7
-
$\begingroup$ can you explain the question more clearly, are you asking about how to derive? $\endgroup$– user243016Commented Jul 17, 2020 at 9:22
-
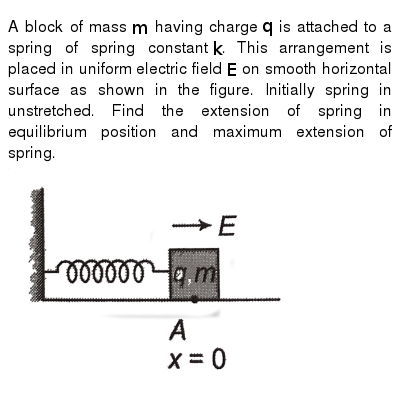
$\begingroup$ @MukunthA.G the question is that a mass m is connected to a spring of spring constant k and that the mass has a charge Q. It is kept in an electric field E . The question then asks what is the maximum displacement and the displacement of the equilibrium postion. $\endgroup$– user270156Commented Jul 17, 2020 at 9:27
-
$\begingroup$ @MukunthA.G hold on I am trying to add an image of the question but its failing to add it. $\endgroup$– user270156Commented Jul 17, 2020 at 9:30
-
$\begingroup$ you could just paste the link location of the image, no need to upload $\endgroup$– user243016Commented Jul 17, 2020 at 9:32
-
$\begingroup$ @MukunthA.G images.app.goo.gl/rtm1RYTcMzAedLGH8 $\endgroup$– user270156Commented Jul 17, 2020 at 9:35
|
Show 2 more comments
2 Answers
$\begingroup$
$\endgroup$
8
The block will remain at rest when the net force on it is zero is a flawed statement
Hence, $$qE - kx = 0$$ only gives the displacement when the net force on the block is zero.
$$x_{F_{net}=0} = \frac {qE}{k}$$
But, $\vec a_{net} = 0$ does'nt mean $\vec v = 0$, there is some velocity of the block which it carries and moves forward but once you have a displacement greater than $x_{F_{net}=0}$ the spring force becomes dominant and the body decelerates.
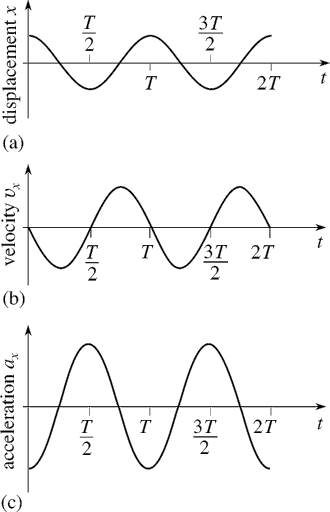
I am skipping the equations of motion for simple harmonic motion but the below graphs may help
Note that at a time $\frac T4$, $\vec a = 0$ but $\vec v = max$. You can think of it like the body moves further because it has built up that much inertia.
Here comes the importance of work energy theorem, since it's hard to find the equations of motion, we use this theorem which states that,
The net external work done on a body is equal to it's change in kinetic energy
In our case $\Delta K = 0$ starting from rest initialy and ending up at rest.
Hence,
$$W_{ext, net} = 0$$ $$W_{spring} + W_{Elec.} = 0$$ $$\int_0^D kx\,\text{d}x - \int_0^D qE\, \text dx = 0 $$
Solving it we get $$D_{block} = \frac {2F}{k}$$
Now you could compare the inital final states of spring, you could say that the mean position has displaced half the displacement of the block.
Another nice thing to mention here is that $kx = qE$ also gives you the same displacement for mean position but you are allowed to do it only after you solve the equations of motion for the block.
-
$\begingroup$ Note that the work done by electric force is negative because cos(180°) = -1 $\endgroup$– user243016Commented Jul 17, 2020 at 10:20
-
$\begingroup$ Also not that this is not an perfect simple harmonic because a constant external force acts on the body $\endgroup$– user243016Commented Jul 17, 2020 at 10:24
-
-
$\begingroup$ you could accept the answer if it really satisfied you, by clicking on that tick mark $\endgroup$– user243016Commented Jul 17, 2020 at 10:28
-
$\begingroup$ I don't see any reference to electric charge in the original question. $\endgroup$ Commented Jul 17, 2020 at 14:08
$\begingroup$
$\endgroup$
5
The force exerted by a spring is determined by its displacement. We use energy when we are given or are looking for a velocity. Referring to the diagram, at equilibrium, $qE = kx_o$. At maximum, $qEx = \frac12kx^2$.
-
$\begingroup$ But that's not O.P's demand, the O.P is a new contributor hence i faired the question for him/her but it still needs to be approved $\endgroup$– user243016Commented Jul 17, 2020 at 14:16
-
$\begingroup$ His original question asked about the difference between using force and energy. $\endgroup$ Commented Jul 17, 2020 at 14:53
-
$\begingroup$ exactly! but he had a peculiar case which i also had, see here , hence he probably needed more facts which explain your statement. $\endgroup$– user243016Commented Jul 17, 2020 at 14:56
-
$\begingroup$ what do you mean by equilibrium? $\endgroup$– user243016Commented Jul 17, 2020 at 15:20
-
$\begingroup$ oh yes! thank you for pointing the difference between static and dynamic equilibrium...i thought equilibrium just meant static. $\endgroup$– user243016Commented Jul 17, 2020 at 15:24