One thing to consider when thinking about quantum-mechanical experiments is the fact that there is very rarely only a single particle involved. Instead, one typically has a beam of particles, and our confidence that single-particle processes are taking place come from observing that very few particles per unit of time are arriving.
The classical example of a spin measurement is the Stern-Gerlach experiment. In this a beam of silver atoms leave a surface to be deflected by an inhomogeneous magnetic field.
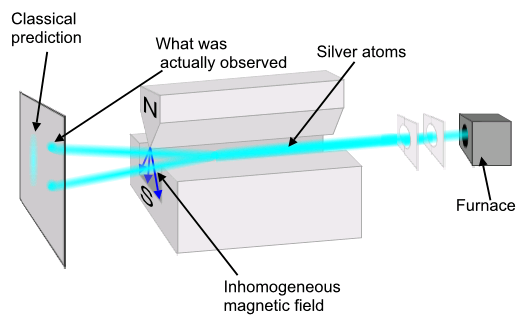
The point is that silver atoms do not have charge which would contribute to the deflection, but the number of electrons in them is such that they have a non-zero total spin $\pm\hbar/2$ and a corresponding magnetic moment. The angle of deflection is thus a measurement of the magnetic moment (which is proportional to spin).
So now you have measured spin and this has split your beam into two. All he electrons in the top one have spin $+\hbar/2$ and the bottom one $-\hbar/2$ (the sign may be switched depending on the splitting magnetic field geometry). In the simplest setup, you verify the split by putting a screen behind the apparatus and observe flashes or dots on it. Once those dots or flashes are created, you have no chance of "remeasuring" the spin though, the silver atoms have reacted in a complex reactions and certainly do not have their original spin.
But you can also choose not to put a screen behind. You can take the top beam and put another apparatus like this behind it. If you align it again in the $z$ direction, in the idealized case it will not split (you can verify by a screen). If you put one oriented in the x direction, it will spit again. If you put another z-aligned apparatus behind that, the beam would split yet again as shown schematically below.

The point is, really, that you make no true observation of whether the beam truly split into two after the first apparatus if you put a second one behind. The only way you are "repeating the measurement" is by putting the same apparatus you have verified to split the beam behind each other twice and then making the final measurement by using the screen that truly destroys any coherent quantum state.
I should also note about your worries about experimental accuracy. In reality, the first Stern-Gerlach apparatus did a little more than just perfectly sorted the beam into two groups. It did it imperfectly (e.g. because there were small but macroscopic imperfections in the field), and it also changed the quantum state in the resulting beams beyond what idealized quantum theory tells you. And you will never align the second apparatus perfectly the second time, but the results should be close if you align it close enough. And you verify this statistically, since you have a semi-continuous beam of particles.
That is how experiments work, idealized quantum theory just tells you about the fundamental limit to how little the effect of a measurement can be. In fact, real quantum experiments work with the notion of a density matrix (or density operator) which expresses our statistical uncertainty about the quantum state the system is in, in a similar way we know it from classical experimental theory.


