Objects rolling down inclined plane at different speeds

Question

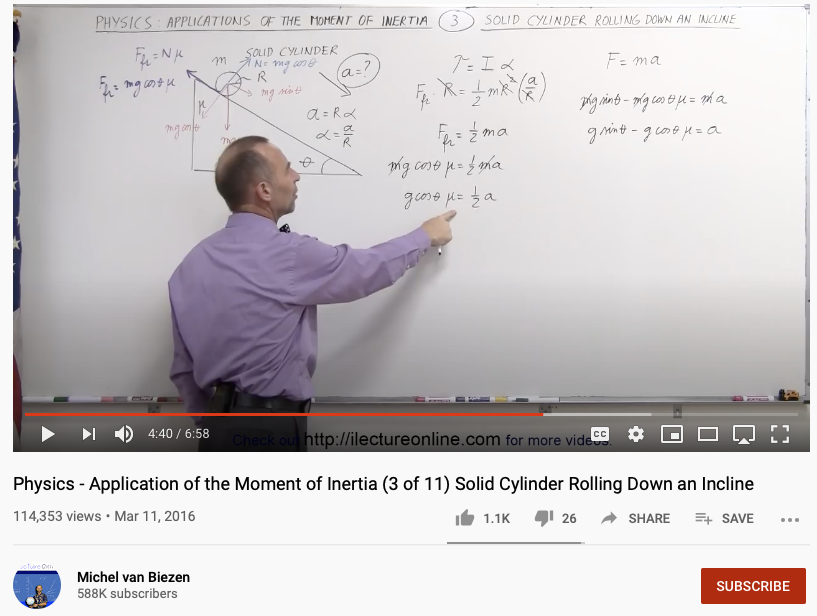
Consider the case of objects rolling down an inclined plane at different speeds depending on their moment of inertia. Some basic equations are shown in this screenshot from Michel van Biezen. He is considering the specific case of a solid cylinder but my question is more general:

Let's say we are comparing a metal solid sphere with a shell of the same radius made with the same metal. We know from demonstrations that the objects do not have the same speed as they move down the plane and don't arrive at the bottom at the same time.

Focusing on the equation of motion along the surface of the plane,

g sin𝜃 - g𝜇 cos𝜃 = a

we see that for the acceleration to be different for the two objects the coefficient of friction, 𝜇, has to be different. If 𝜇 were the same then both objects's center of mass would reach the bottom of the plane at the same time.

So that leads me to ask why is 𝜇 different for a solid sphere and a shell, as it appears it must be?

I have looked at What factors determine the coefficient of friction? and don't think it answers my question. In the case I'm asking about, the materials of the sphere and shell are the same, as are the total mass and radius.

Answer 1

So that leads me to ask why is $\mu$ different for a solid sphere and a shell, as it appears it must be?

The $\mu$ don't have to be different or the same. Depending on the inclination $\theta$ only a part of the potentially available friction force is used to prevent sliding. Assuming roughness is sufficient, neither rolling objects will start slipping, even if the friction force will in both cases be smaller than the potential friction force.

Consider the simpler case: a block that might slide down an incline:

For a stationary block the net force would appear to be:

$$mg\sin\theta-\mu mg\cos\theta=0$$

But of course it cannot be negative! That would mean the block would start moving to the left!

In reality, only the useful part of the friction force is used:

$$F_f \leq mg\sin\theta$$

---

Assuming there's enough friction to prevent slipping, then inertia comes into play. As potential energy $U$ is converted to kinetic energy $K$, we can write:

$$\Delta U=\Delta K$$ $$mgh=\Delta K_{trans}+\Delta K_{rot}$$ $$mgh=\frac12 mv^2+\frac12 \gamma mv^2$$ where $\gamma$ is a factor, dependent on the inertial moment $I$.

We get:

$$\boxed{v^2=\frac{2gh}{1+\gamma}}$$

For a full cylinder $\gamma$ is higher than for a shell-type cylinder, so $v$ is higher for the latter case.

Answer 2

While Gert's answer implies this, your main issue is in assuming that static friction is equal to $\mu N$ here. For static friction, $\mu N$ is the maximum value static friction can take, but generally static friction will be less than this value.

Therefore, all we can say for the net force acting on the object along the incline is $$ma=mg\sin\theta-F_s$$

And the net torque acting on the object must be $$I\alpha=RF_s$$

Noting that for simple rolling objects $I=\gamma mR^2$, and for rolling without slippping $a=\alpha R$, one can show that

$$F_s=\frac{\gamma}{1+\gamma}\cdot mg\sin\theta$$

So as we can see, we do get different static friction forces for objects with different moments of inertia, even if they are made of the same material on the same incline.

If you do want to look at slipping conditions, then we see what $\theta=\theta_\text{slip}$ needs to be such that slipping occurs, i.e. where $F_s=\mu N=\mu mg\cos\theta$. This gives us $$\theta_\text{slip}=\tan^{-1}\left(\frac{1+\gamma}{\gamma}\cdot\mu\right)$$

So, we also see that the angle of slippage depends on both $\mu$ and $\gamma$.