Confused about the direction of the Magnus effect force

Question

From what I've read, the cause for the force acting as the result of the Magnus effect is the formation of a bended wake behind the moving sphere.

Take a look at that picture:

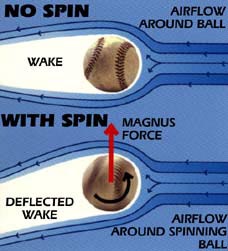
_{(source: jschetz at www.dept.aoe.vt.edu)}

From that picture, it appears to me that since the pressure gradient is directed into the wake region, the vertical force acting on the ball should be directed downwards, and not upwards how it is indicated in the picture.

Is this picture wrong and the wake should be reflected about the x-axis?

From my understanding, the effect responsible for detachment of the boundary layer is the development of turbulence, and turbulence should occur faster on the side where the relative velocity of the air is bigger - on top of a backspinning ball, since in that case the ball will be giving its momentum to the surronding air faster, through friction, therefore reaching the speed where turbulence occurs at the point of the x-axis located more to the right (and not as indicated on the picture, where it's more to the left (on the top side of the ball)).

Edit: Sorry, the last paragraph is wrong. I incorrectly determined where the relative velocity is bigger. My question therefore changes to the following - If this picture of the wake is correct, then why is the force directed upwards, and not downwards?

Answer 1

The direction of force is determined by the Bernoulli's theorem. If I have to explain briefly Bernoulli Theorem suggest that in a fluid, if one region of the fluid have more kinetic energy than the other then the former have less pressure than the latter (if the region is on the same level i.e.no gravitation energy and atmospheric pressure is constant). So you can see that in the figure the upper region of the air will be faster due to the frictional force exerting by the ball on the air as compared to the lower region. This means that upper region has less pressure as compared to lower one. We know that fluid tend to move from higher pressure region to lower one and that's why fluid exert upward pressure on the ball.

Answer 2

The figure shows that the fluid is being directed downward. Some force on the fluid has to be doing this by giving the fluid downward momentum. By Newton's third law the fluid must be giving the thing pushing it a push in the opposite -- i.e upward -- direction. Hence the fluid pushes the ball upward.

Answer 3

The answers by mike stone and sslucifer give two parts of the full explanation, respectively Newton's laws of motion and Bernoulli's principle. The third part of the explanation is the circulation theory of lift.

For a conventional airfoil travelling forwards, the pressure differences create a circulation component of flow; backwards over the top, down at the rear, forward underneath and up round the leading edge. This circulation creates a positive feedback which increases the lift several times compared to a flat plate. The faster you fly, the greater the effect.

A spinning rotor creates its own circulation through friction across the boundary layer. It only needs to travel forwards relatively slowly for the circulation to create and magnify the Bernoulli effect, deflect a significant amount of air downwards and, in Newtonian reaction to that, create useful lift. This is the Magnus effect.

However with respect to the tennis ball illustrated, it was the then Lord Rayleigh who first experimented with spinning tennis balls and established that their curved flight was due to the Magnus effect, back around 1850.

Answer 4

The picture is correct because backspin balls go up.

The reason is related to air drag. For usual ball velocities in tennis, table tennis or football, the drag force is proportional to the square of the velocity. This force is distributed along the surface facing the flow: $F = \int Pds$, where $P$ is the pressure on a point of this surface.

When the ball spins around an axis orthogonal to its translational velocity, the pressure at a point momentarily rotating against the air flow (the bottom region in the case of a back spinning ball) is greater than in the side rotating with the flow. And the reason is: the relative velocity with respect to the air flow is greater, and the drag is proportional to the square of that velocity.

So, the pressure below the centre of the ball along the hemisphere facing the flow is greater than above the centre. The result of $F = \int Pds$ is not exactly opposite to the flow direction, but has a component upwards.