Finding the amplitude for the pair production $\gamma(p_1) + \gamma (p_2) \to e^- (p_3) + e^+ (p_4)$

Question

I'm trying to find the amplitude for:

$$\gamma(p_1) + \gamma (p_2) \to e^- (p_3) + e^+ (p_4)\tag{1}$$

(My questions are stated in the end)

The possible answers are:

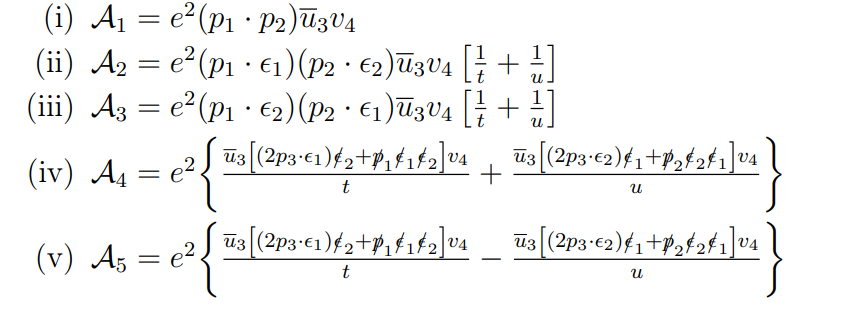
My take on it:

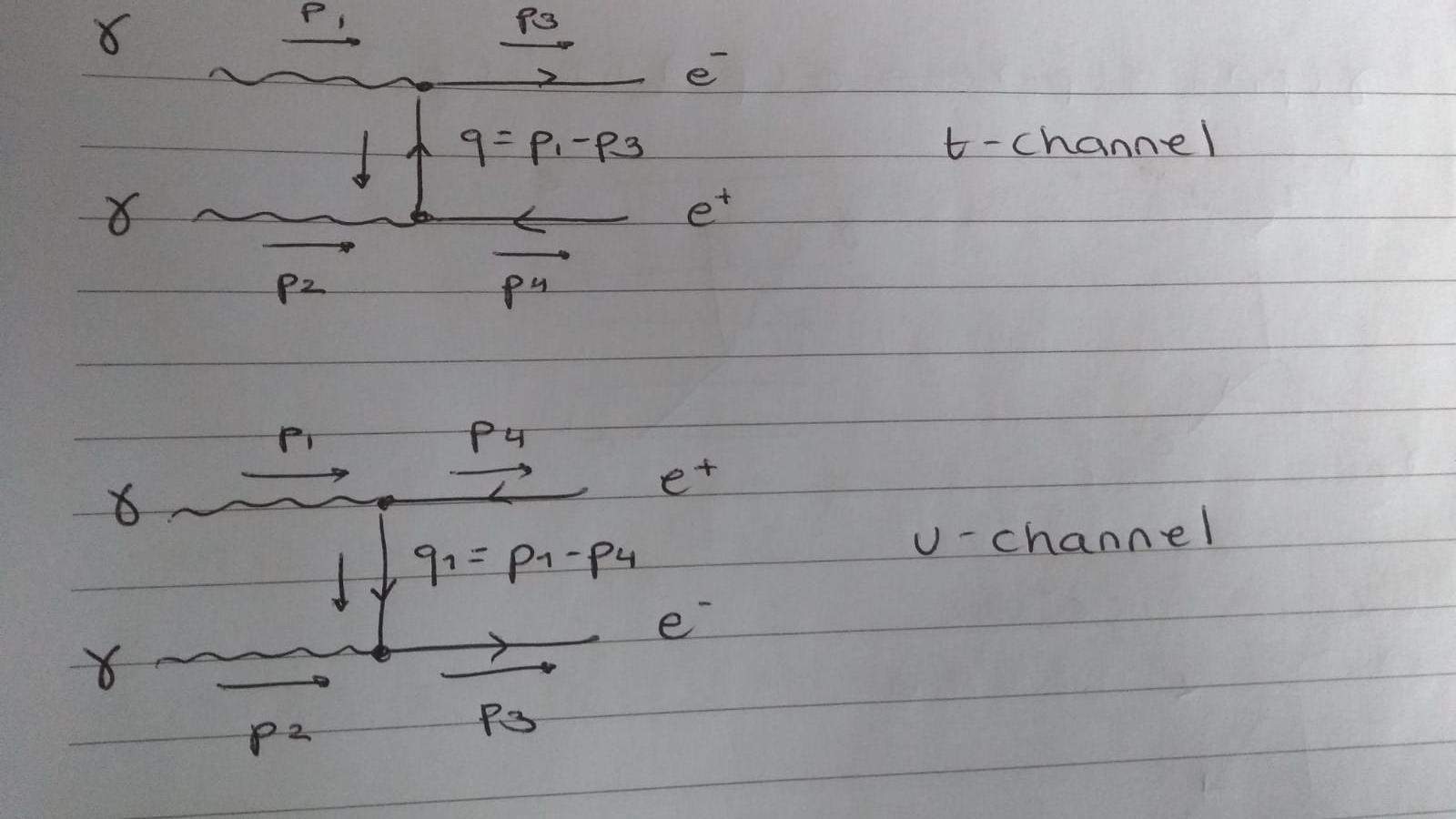
and so

$$\tag{2} \require{cancel} \mathcal{M}= e^2\{ \epsilon_1 \gamma \bar{u}_3 \frac{(\cancel{p_3}-\cancel{p_1})}{t} \nu_3 \gamma\epsilon_2 - \bar{u}_3 \gamma \epsilon_2 \frac{\cancel{p_2}-\cancel{p_3}}{u}\epsilon_1 \gamma\nu_4 \}$$

I know it is (v) but I don't' understand the following (as my answer is not present in the same form as the ones presented):

Where does the factor of 2 in $(iv)$ and $(v)$ come from?

Answer 1

Where does the factor of 2 in $(iv)$ and $(v)$ come from?

It comes from the anticommutation relation for the Dirac gamma matrices,

$$\gamma_\mu\gamma_\nu+\gamma_\nu\gamma_\mu=2\eta_{\mu\nu}I_4\tag{a},$$

where $\eta_{\mu\nu}$ is the “mostly minus” Minkowski metric and $I_4$ (which is often omitted but implied) is the $4\times 4$ identity matrix.

In terms of "slashed" matrices ($\not a\equiv a^\mu\gamma_\mu$), this is

$$\not a\,\not b+\not b\,\not a=2(a \cdot b)I_4\tag{b}.$$

Your expression (2) has several errors. Once you correct them, you can use (b) to get the slashed matrices in the order that the answer wants.