2
$\begingroup$

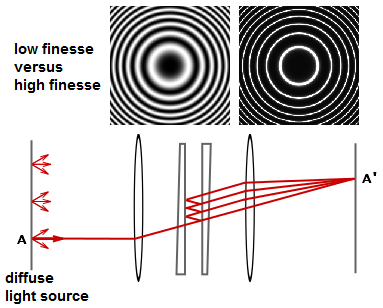
let's consider a Fabry-Perot interferometer:

enter image description here

Illumination is provided by a diffuse source, and its rays are focused towards the cavity by a lens. Inside the cavity there are multiple reflections and transmissions of rays. Rays transmitted towards right will have a certain phase shift depending on the input wavelegth. Therefore, we may see only certain wavelegth on a screen at right, because the cavity acts like a filter because the output rays intensity is expressed by the Airy function, which has very strict peaks:

enter image description here

So, the wavelengths that correspond to those peaks are seen on the screen (rays interfere constructively), others are not seen (rays interfere destructively).

Now my question is: why do why see the circles in the first picture on the screen? It seems to me that the lens at right focuses all the transmitted rays in the same point A'. So I'd say that at A' we will see "light" from certain wavelength, "dark" from others. No other points of the screen receive rays, in that picture.

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ Edited: Each point on the screen is the image of each angle $ \theta $ of incidence of the light. That particular point A' (it's ring) corresponds to some particular $ \theta $. Each wavelenght will have a ring pattern (long wavelenghts will have shoter one). When you add up those patterns you should get a light (when all wavelenghts peaks match) / dark (when they doesn't). I'm not familiar with the notation on those graphics. I saw them on Wikipedia but I haven't read the article. $\endgroup$
    – Gilgamesh
    Commented May 10, 2020 at 8:29
  • $\begingroup$ @MarcoCiafa Thank you! I think I have understood: fixed the angle theta of incidence, we have a circle (theta corresponds to 1 point on the screen) whose intensity depends on theta and on the superposition of all wavelenghts coming from the cavity. If we repeat this analysis for each value of theta, we get many points on the screen, and for each of them there is a circle. So the resulting image is a set of circles with same center. Correct? $\endgroup$
    – Kinka-Byo
    Commented May 10, 2020 at 10:45
  • 1
    $\begingroup$ To be clear, for each $\theta$ maps into a circle. I agree that if $\theta$ is om the same plane than the y-axis (screen axis) you will get the point on that plane. Turning on the x-axis (perpendicular to the screen) with the same $\theta$ you will get all the circle. Repeat por each angle of incidence. $\endgroup$
    – Gilgamesh
    Commented May 10, 2020 at 16:09
  • $\begingroup$ I will post it as an answer if that helped you. $\endgroup$
    – Gilgamesh
    Commented May 10, 2020 at 16:10

1 Answer 1

Reset to default
1
$\begingroup$

Let the x-axis be perpendicular to the screen passing through the center of both lenses and the y-axis be on the screen on some arbitrary direction. Let $\theta$ be the angle formed by the ray and the x-axis after the first lens. Let's think it in 2D (xy plane).

The interference of the ray with angle $\theta$ will be imaged on the point who forms an angle $\theta$ with the center of the second lens and the x-axis (that's because of how convex lens works). As we have symetry of rotation on the x-axis for each $\theta$ we have a ring. Each point on the screen is the image of the single ray that is contained on the plane that contains the x-axis and the point and has angle $\theta$.

That explanation goes for a single wavelenght. As you know, different wavelenghts do not interfer with themselves due to their temporal incoherence. Each wavelenght will have a ring pattern (long wavelenghts will have shoter one, rings will be closer). When you add up those patterns you should get a light (when all wavelenghts peaks match) / dark (when they doesn't) pattern.

Improve this answer
$\endgroup$
6
  • $\begingroup$ I am thinking again about this topic. Precisely, consider a single ring on the screen: we will see only some wavelengths (let's call them lambda_First_i: so lambda_First_1, lambda_First_2, ...). Now consider another ring: we will see only some wavelengths, which are different from the previous ones because of different incidence angle (let's call them lambda_Second_i: so lambda_Second_1, lambda_Second_2, ...). So, I do not understand why do we see on the screen some dark and light rings. I'd say we will see rings of different colours (yellow ring, blue ring etc),not of different intensity $\endgroup$
    – Kinka-Byo
    Commented May 23, 2020 at 7:48
  • $\begingroup$ So I do not understand why rings of different wavelengts are seen as rings of different intensity $\endgroup$
    – Kinka-Byo
    Commented May 23, 2020 at 7:49
  • 1
    $\begingroup$ Because the maxima rings are close enought that you can't distinguish them and you only see white light. If your lenses are big enoght and you take a $\theta$ as big as you can, you should see different rings for each wavelenght . But I don't know at what ring number preciselly that would happen. $\endgroup$
    – Gilgamesh
    Commented May 23, 2020 at 16:54
  • $\begingroup$ And what do dark rings represent? All light wavelenghts interfere destructively? $\endgroup$
    – Kinka-Byo
    Commented May 23, 2020 at 17:36
  • 1
    $\begingroup$ All wavelenghts interfere (with themselves, in the interferometer) destructively. $\endgroup$
    – Gilgamesh
    Commented May 23, 2020 at 18:35

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.