1
$\begingroup$

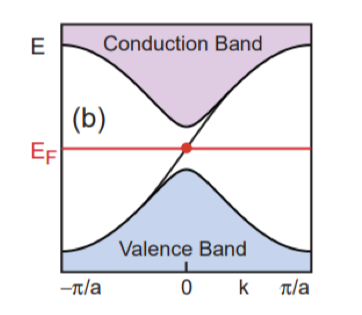
Consider one edge state for a Chern insulator. It could have the band structure as shown below. enter image description here

We can see that the band for the edge state does not obey $E_{-\pi/a}=E_{\pi/a}$. I remember the property: $E_{k}=E_{k+G}$ (where G is a reciprocal lattice vector) is a consequence of Bloch theorem which is guaranteed by the periodicity of the lattice. Since the periodicity along the direction of k is not broken here, why is this band not periodic by $2\pi/a$?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
2
$\begingroup$

As this is an edge state, your system must have open boundary conditions in at least one direction (otherwise the system will not have an edge). This breaks translational symmetry, and so Bloch's theorem is not applicable in this direction.

Improve this answer
$\endgroup$
3
  • $\begingroup$ But the translational symmetry is not broken along the other direction. Suppose we have a strip with translational symmetry along the x-direction. The good quantum number k in the figure above should be $k_x$ and its direction is also the x-direction. Bloch theorem seems to be applicable to this one-dimensional system? $\endgroup$
    – Yang
    Commented May 7, 2020 at 10:31
  • $\begingroup$ Ah, I think I understand your question better now. I believe this figure is for a system with a single boundary, and so the edge state only propagates along it in one direction (from the figure you can see that it has a positive group velocity). There is no reciprocal lattice vector that can transform this into a state propagating in the opposite direction. If you had two boundaries, like a system on a cylinder, there would be two edge states, one going to the left and one to the right, and your condition $E_k = E_{k+G}$ would be satisfied. $\endgroup$
    – Clara Díaz Sanchez
    Commented May 7, 2020 at 12:28
  • $\begingroup$ Exactly! If there are two boundaries in the system as you mentioned, there are two bands corresponding to the two edge states. Then, we can view the upper part of the two bands as one band and the lower part as the other band so that $E_{k}=E_{k+G}$ is meet in both bands. However, for the one boundary situation, there is no such explanation. And I think the one boundary situation is not an unphysical one. For example, we can have half of the space with zero Chern number and the other half with non-zero Chern number as long as the space we study is small enough compared with the whole sample. $\endgroup$
    – Yang
    Commented May 7, 2020 at 13:07
0
$\begingroup$

Bloch's theorem states that if there is a state at a given energy at momentum k, there is a state at the same energy at momentum $k+2\pi/a$. This property is perfectly well obeyed by the picture you drew, if you take into account all the bands.

I think your confusion is in trying to treat the edge mode as a separate band and ignore all the other bands. You can't do this, as the edge mode merges into the valence and conduction bands and does not constitute a well-defined band on its own.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.