5
$\begingroup$

There appears to be a confusion on what the Tyndall effect actually is.

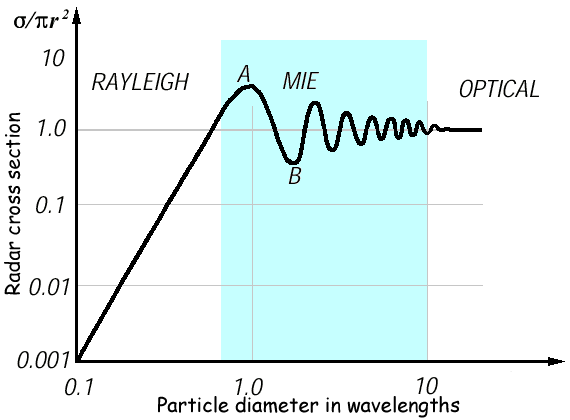
In every textbook in my native language (Polish) I have read, the 'Tyndall effect' is simply a name for a cone of light that arises when light passes through a colloid. As it is said to be independent of wavelength (contrary to Rayleigh scattering) and it happens for particles of roughly similar size to the wavelengths of visible light, it seems the underlying mechanism is suggested to be Mie scattering. The same description goes for Russian, German and French Wiki.

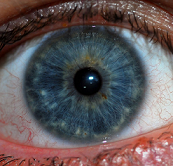
Meanwhile, English and Spanish Wiki tells that it is actually a phenomenon akin to Rayleigh scattering (that is, shorter wavelengths are scattered more), yet it concerns particles of similar size to the wavelengths indeed. Examples given are blue irises, exhaust smoke and opalescent glass.

So what actually the Tyndall effect is? If shorter wavelenghts are scattered more (the latter approach), then wouldn't it agree well with Rayleigh scattering mechanism? If not, then how is it different from Rayleigh scattering? And if it apparently occurs for particles of diameter comparable to the wavelength (as in Mie scattering), then why is blue light scattered more?

Edit: I shouldn't have referred only to Wikipedia in my question. My biggest concern is the discrepancy between the sources. He et al. (DOI: 10.1063/1.3068473) emphasize that Tyndall scattering arises for particles much larger than the wavelength (it is then independent of wavelength), whereas Flammer et al. in Basic Sciences in Ophthalmology point out that Tyndall effect is indeed light scattering due to submicroscopic particles. Yet I don't see why changing particle size would give two distinct phenomena (Rayleigh's & Tyndall's) if, according to the latter approach, you get Rayleigh-like dependence.

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ the english wiki explains the difference to Rayleigh scattering, But in short, the particles which make Tyndall scattering are in the order of the wavelength of light the Rayleigh particles are much smaller $\endgroup$
    – trula
    Commented Apr 18, 2020 at 17:59

2 Answers 2

Reset to default
4
$\begingroup$

Actually, as Wikipedia says,

Also known as Willis–Tyndall scattering, it is similar to Rayleigh scattering, in that the intensity of the scattered light is inversely proportional to the fourth power of the wavelength, so blue light is scattered much more strongly than red light.

The difference from Rayleigh scattering is that Tyndall's is scattering on colloidal particles, which have sizes on the order of the wavelength of light. This results in several specifics:

  • Total scattering cross section per particle is much larger
  • Shape of a particle can play a considerable role in shaping the differential cross-section
  • Actual total scattering cross section isn't always proportional to $\lambda^{-4}$ as it always is in the Rayleigh regime: it's more complicated (image source):

enter image description here

Here you can see that in smaller-particle colloids (iris of the eye, milk) you get Rayleigh-like dependence on particle size relative to wavelength, while for larger-particle ones (cumulus clouds) you get less dependence. In colloids with intermediate-sized particles or with simply large range of sizes you can even get not-well-defined dependence on wavelength: e.g., in iridescent clouds.

Improve this answer
$\endgroup$
4
  • $\begingroup$ I shouldn't have referred only to Wikipedia in my question. My biggest concern is the discrepancy between the sources. He et al. (DOI: 10.1063/1.3068473) emphasize that Tyndall scattering arises for particles much larger than the wavelength (it is then independent of wavelength), whereas Flammer et al. in Basic Sciences in Ophthalmology point out that Tyndall effect is indeed light scattering due to submicroscopic particles. Yet I don't see why changing particle size would give two distinct phenomena (Rayleigh's&Tyndall's) if, according to the latter approach, you get Rayleigh-like dependence. $\endgroup$
    – Daniel
    Commented Apr 19, 2020 at 3:23
  • $\begingroup$ @Dyaego well, apparently, different sources confuse what to call Tyndall scattering. In any case, these are not distinct phenomena (if we are talking about spherical particles): they are different regimes of the same phenomenon: scattering by spheres. And Mie scattering is not even a separate regime, since Mie theory works for spheres of any size, thus being applicable to both Rayleigh and Tyndall regimes, as well as for description of rainbows etc.. $\endgroup$
    – Ruslan
    Commented Apr 19, 2020 at 6:27
  • 1
    $\begingroup$ @Dyaego BTW, Wikipedia entry on John Tyndall has a note: "The term Tyndall Scattering is subject to some definitional overlap with the terms Rayleigh Scattering and Mie Scattering." $\endgroup$
    – Ruslan
    Commented Apr 19, 2020 at 6:27
  • $\begingroup$ I guess everyone has to settle this predicament themselves and opt for a specific option (or refrain from using the term at all). The fact that Tyndall's research preceded Rayleigh's or Mie's and that he gave only a general description (without solid physical argumentation) might be the reason for the disagreement. Nonetheless, thank you for your contribution, it helped me a lot undoubtedly! $\endgroup$
    – Daniel
    Commented Apr 19, 2020 at 15:52
0
$\begingroup$

Scattering as the name suggests happens when the light is scattered due to particles. Now depending on the wavelength of light scattered and size of the particles which scatter the light, you can have Rayleigh or Tyndall scattering.

Tyndall scattering happens due to colloidal particle.

And, Rayleigh due to atmospheric particles.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.