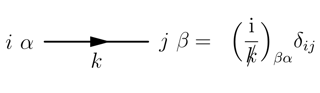5
$\begingroup$

I'm having hard times in finding the Feynman rules for the Gross-Neveu model in two dimensions: $$ \mathcal L = \bar \psi^i (i \gamma^{\mu} \partial_{\mu} ) \psi^i + g^2 (\bar \psi^i \psi^i )^2 \; ,$$ where $i = 1, \ldots, N$ and $\mu = 1, 2$. Moreover $\gamma^0 = \sigma^2$ and $\gamma^1 = i\sigma^1$ where $\sigma^i$ are the Pauli matrices.

The propagator is obvious: fermion propagator

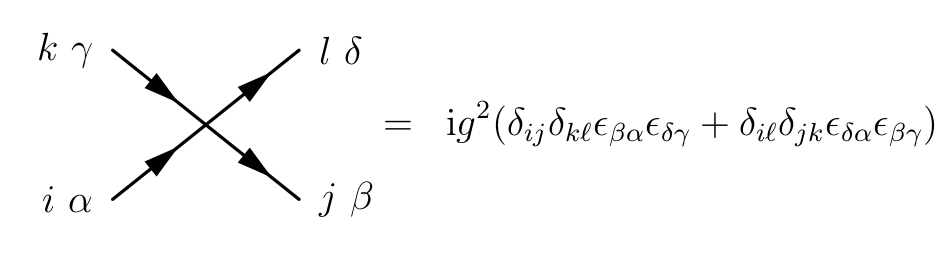
while the 4-point diagram should be: 4 point Feynman diagram

Question: How are the $\epsilon$'s defined? Where they come from?

Moreover, since any propagator brings two Dirac index, one at the beginning and one at the end of the propagator, there shouldn't be another Dirac index at the center of the four-point diagram?

Improve this question
$\endgroup$
7
  • $\begingroup$ Are you sure those are $\epsilon$s and not $\gamma$s? $\endgroup$
    – Sounak Sinha
    Commented Apr 16, 2020 at 12:47
  • $\begingroup$ @SounakSinha Yes, moreover it seems they obey the following relation: $$ \epsilon_{a b} \frac{1}{\gamma^{\mu}_{bc}} \epsilon_{cd} = \gamma^{\mu}_{ad} $$ This is the source: zzxianyu.files.wordpress.com/2017/01/peskin_problems.pdf see from page 93 $\endgroup$
    – ACA
    Commented Apr 16, 2020 at 13:09
  • 1
    $\begingroup$ The gamma matrices are $2\times2$. So I guess those are the Levi civita tensors of two dimensions. $\endgroup$
    – Sounak Sinha
    Commented Apr 16, 2020 at 13:18
  • 1
    $\begingroup$ For what it's worth, I think those should be Kronecker deltas in the spinor indices, not Levi Civita symbols. I think the author of those homework solutions was intending to include the $\gamma^0_{ab}=-i\epsilon_{ab}$ part of $\bar{\psi}$ in the vertex, but if you do that you would need an extra factor of $\gamma^0$ in the propagator. I am not writing this as an answer because I am not 100% sure, but I have indeed found mistakes in those solutions to Peskin problems before so beware. $\endgroup$
    – octonion
    Commented Apr 16, 2020 at 19:24
  • 1
    $\begingroup$ I have worked out the confusion about the Kronecker deltas in the answer to this question. $\endgroup$
    – mkn
    Commented Feb 17, 2023 at 0:09

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.