Addition of angular momentum and (anti)symmetry under exchange of constituent angular momenta

Question

I have two potentially distinguishable particles, each with spin $s_1=s_2=s$. I'll only be looking at spin degrees of freedom. I'll write the total spin eigenvectors $|s_{tot} m\rangle$ in terms of the constituent spin eigenvectors $|s_1 m_1\rangle|s_2 m_2\rangle$ by means of the Clebsch-Gordan coefficients.

A look at a table of Clebsch-Gordon coefficients shows that my total spin eigenvectors are either symmetric or antisymmetric under exchange of the constituent spins.

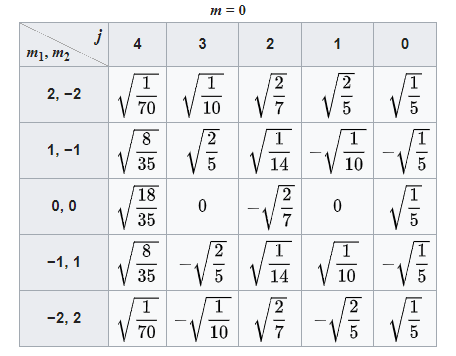
For example, for $s_1=2$ and $s_2=2$, with $m_1 + m_2=0$, we have the following table (with $s_{tot}=j$):

For even $s_{tot}$, we have that exchanging the labels $m_1$ and $m_2$ leaves the state unchanged, while for odd $s_{tot}$, exchanging the labels $m_1$ and $m_2$ causes us to pick up a minus sign. It is clear by scanning Clebsch-Gordon tables that this is true for all integer constituent $s$, while the opposite is true for all half-integer constituent $s$: half-integer $s$ leads to antisymmetric even $s_{tot}$ states and symmetric odd $s_{tot}$ states, as can be seen in the example of the spin addition of two spin 1/2 particles.

What is this "symmetry or antisymmetry of total angular momentum states under exchange of the constituent angular momenta" property called? It reminds me a bit of parity, especially with the signs depending on the evenness and oddness of the total angular momentum. What's a speedy way to prove that the paragraph above is true?

Answer 1

I don't think this property has a name, but it's not too hard to show. In fact, it pops out automatically if you revisit how the Clebsch-Gordan coefficients are derived in the first place, keeping track of the symmetry.

If you start with spins $s$, then the maximum total $m$ you can get is $2s$, from the state $|m_1 = s, m_2 = s \rangle$. This state must be the highest $m$ state of a spin $2s$ representation, and the state is symmetric under exchanging the spins. Now, the spin lowering operator treats the two spins symmetrically, so if you keep lowering, you can recover the rest of the representation, and all states are symmetric under exchanging the spins.

Next, consider the states with $m = 2s-1$. This is a two-dimensional space, spanned by the vectors $$|m_1 = s, m_2 = s-1 \rangle, \quad |m_1 = s-1, m_2 = s \rangle$$ The symmetric combination of these states is already accounted for, since it's in the spin $2s$ representation. The antisymmetric combination is thus the highest $m$ state of a spin $2s-1$ representation. Again, by lowering, we find that all the other states in this representation are also antisymmetric.

Next, consider the states with $m = 2s-2$. This is a three-dimensional space, spanned by $$|m_1 = s, m_2 = s-2 \rangle, \quad |m_1 = s-2, m_2 = s \rangle, \quad |m_1 = s-1, m_2 = s-1, \rangle.$$ This space has a two-dimensional symmetric subspace and a one-dimensional antisymmetric subspace. The latter is already spoken for by the spin $2s$ component, and the spin $2s-1$ component takes up one of the symmetric states, leaving a symmetric state that must be the highest $m$ state of a spin $2s-2$ representation. Now you can see how the pattern continues.