Has a reflected wave an arbitrary phase shift?

Question

Let an EM wave propagate in the $\hat{z}$ direction - $$\vec{E_I}(z,t)=E_0e^{i(kz-\omega t)}\hat{x}$$ it hits a conducting surface at $z=0$ so there is a reflected wave - $$\vec{E_R}(z,t)=E_{0R}e^{i(-kz-\omega t)}\hat{x}$$ Since the total field must vanish on the conducting surface we conclude that - $$E_{0R}=E_0e^{i\pi}$$ However, if the conducting plane was placed at $z=L$ we would find - $$E_{0R}=E_0e^{i(2kL+\pi)}$$ It appears that the phase difference (which is physical?) between the incoming and reflected wave is arbitrary. On the other hand, our choice of coordinates is also arbitrary. As far as the waves are concerned, the position of the conducting plane should not matter at all, so there is an apparent conflict.

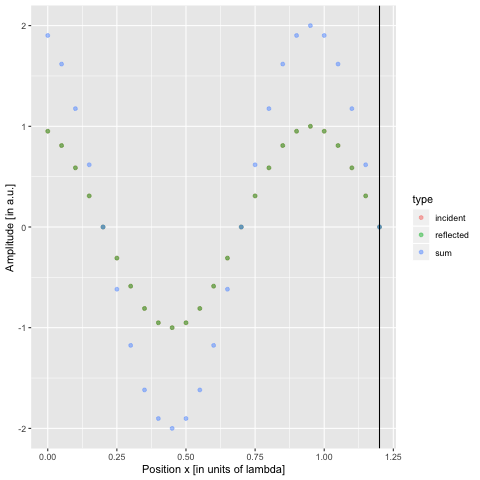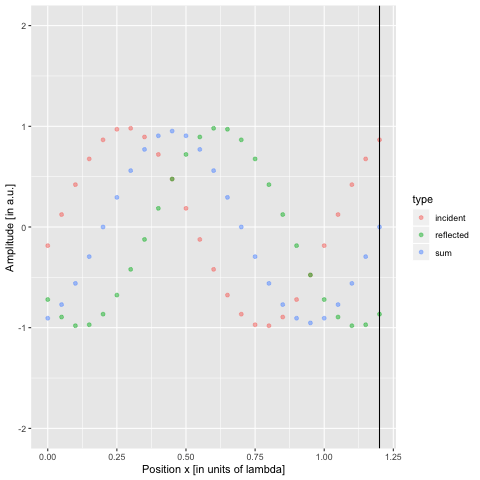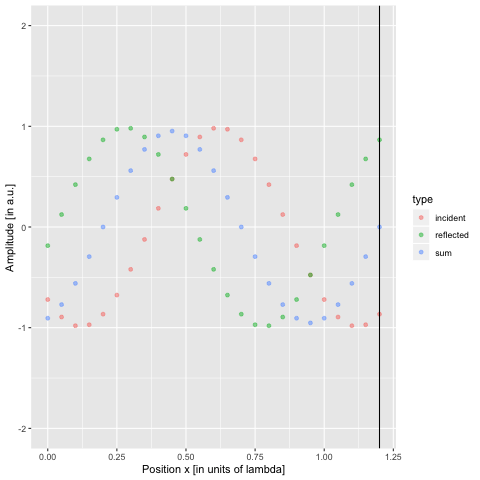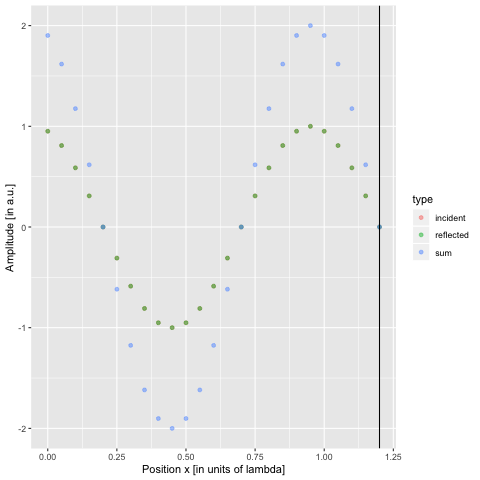
Edit: We found the two waves to be - $$\vec{E_I}(z,t)=E_0e^{i(kz-\omega t)}\hat{x}$$ $$\vec{E_R}(z,t)=E_0e^{i(k(2L-z)-\omega t + \pi)}\hat{x}$$ Their sum is a standing wave - $$\vec{E_I}(z,t) + \vec{E_R}(z,t)= E_0(e^{i(kz-\omega t)} + e^{i(k(2L-z)-\omega t + \pi)})\hat{x}= E_0e^{i(kL-\omega t)}(e^{ik(z-L)} - e^{-ik(z-L)})\hat{x}= 2iE_0e^{i(kL-\omega t)}\sin(k(z-L))\hat{x}$$ And the difference in their phases is - $$\Delta \phi(x)=2k(L-z)+\pi$$ which at $z=L$ comes out as $\pi$ so the boundary conditions are satisfied. However in some other points the phase difference is not $\pi$

Edit2: If the reflected wave gains only an additional phase $\pi$ an immediate conclusion is that the wavenumber must be quantised. This is odd, because if the surface is moved just a little bit further away, the standing wave will be destroyed. This will lead to a violation of the boundary conditions at the interface.

Answer 1

The so-called Polarization-Twisting Reflector takes advantage of this by making parallel grooves whose depth and separation is designed so that the wave that enters the grooves has an equivalent depth that gives approximately $\pi/2$ shift relative to the one reflected at the front surface so the round trip difference is $\pi$. When the plate is illuminated with a linearly polarized, say vertical, wave and the grooves are tilted at $\pi/4$ relative to the vertical then the reflected wave will be horizontally polarized, hence the name polarization-twisting reflector! The scheme is used in Cassegrain and similarly constructed two-reflector antennas.

Answer 2

Consider two such reflecting surfaces facing each other such that a standing wave is set up between them. Clearly, the phase of the reflected component of the standing wave is not arbitrary.

As has been pointed out, if you take an arbitrary coordinate origin then all absolute phases are as arbitrary as your choice of origin. Relative phases between waves remain are unchanged.

Answer 3

Firstly, the condition of the relative phase $\varphi_2(x,t) - \varphi_1(x,t) = \pi$ applies only to the point in space, where the mirror is located. Hence, it applies only to $x=L$, but for all times $t$. If it would apply to all points in space, the sum of the two waves would be zero. Hence, we would not obtain a standing wave, but zero amplitude everywhere in space.

Secondly, let's start @ $x=0$ with a wave travelling to the right, $ y_1(x,t) =e^{i(\omega t - kx)} = e^{i \varphi_1(x,t)} $, and a wave travelling to the left, $ y_2(x,t) =e^{i(\omega t + kx + \phi)} = e^{i \varphi_2(x,t)} $. Please note, that $\phi$ is the phase of the reflected wave at position $x=0$ (and time $t=0$ -- as time is irrelevant for the following arguments, I will omit it in the further discussion). Now, let's apply the stated boundary condition. For the point $x=L$ we get $$ \pi = \varphi_2(L,t) - \varphi_1(L,t) = 2kL + \phi $$ which leads to $\phi = \pi - 2kL$. Let's consider each of the two terms separately:

• The first term, $\pi$ is phase shift due to the reflection on an optical denser medium.
• The second term, $2kL$, is the phase of the reflected waves at position $x=0$.

Look at it this way: The reflected wave, which is at time $t=0$ at $x=0$ is the "incident wave of the past" ($t<0$). This "incidence wave of the past" has travelled the distance $2L$. Therefore, it has picked up the phase $2kL$ in addition to the phase shift.

Finally, note that a clever way to express the phase of the incident and reflected wave is to use \begin{align} y_1(x,t) &=e^{i(\omega t - k(x-L))} \\ y_2(x,t) &=e^{i(\omega t + k(x-L)+ \pi)} \end{align} E.g. using $L=1.2\lambda$ yields the following

Answer 4

The absolute phase of any plane wave is arbitrary because you can always translate your coordinate system, that is correct.

However, what is not arbitrary is the phase difference between two waves, as the arbitrary phase of the two waves will cancel when you consider the phase difference, leaving only an intrinsic phase shift between the two. In the case of the metal slab as you mentioned, the $\pi$ phase shift between the incident and reflected beam will always occur no matter your choice of coordinates.

This is really no different than the case of two objects at positions $x_1$ and $x_2$. A friend down the street will say they are instead positioned at $x_1'$ and $x_2'$, but both will agree on the distance between the two is $\Delta x = \vert x_2-x_1\vert= \vert x_1' - x_2' \vert$ (unless you're moving near the speed of light of course).