How does even water rise along a glass plate?

Question

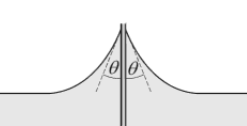
So, I was studying about general properties of matter and topics like surface tension. I came across the phenomenon of water rising along a glass plate like in the picture. I looked for some mathematical interpretation of this on the internet and in some books.

I looked for some mathematical interpretation of this on the internet and in some books. I found some mathematical understanding of the phenomenon in the book Capillarity and Wetting Phenomena: Drops, Bubbles, Pearls, Waves and also elaborate answers on StackExchange like this one: How far can water rise above the edge of a glass?

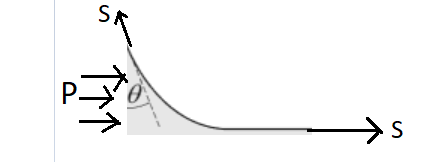
But I decided to find the height along which the water climbs on the glass by balancing forces on the infinitely long water element:

It is to be noted that the height of this water element is $h$ and it has an infinite length in the horizontal direction.

Now the pressure force $P$ can be calculated as $P=\int_0^h \rho gz dz=\frac{1}{2}\rho g h^2 $

On balncing forces in the horizontal direction, we get $$P+S =S\sin \theta$$ $$\Rightarrow \frac{1}{2}\rho g h^2= S(\sin \theta -1)$$ which is surely a contradiction as the term in the left hand side is bound to be positive. Hence I believe that I have apparently disproved the fact that water would rise along the glass plate. But I also know that this is true that water has to rise as evident from daily experiences. So, where does my math go wrong?

Answer 1

If depth z is measured downward from the upper tip of the meniscus, then at depth z below the tip, the liquid pressure is given as: $$p(z)=p(0)+\rho g z$$where p(0) is the liquid pressure at the tip (It is not equal to atmospheric pressure because of the curved interface between the liquid and the atmosphere).

And at depth z = h, representing the lower flat surface of the liquid, the pressure is atmospheric: $$p(h)=p_a=p(0)+\rho g h$$So, combining these two equations, we get: $$p(z)=p_a-\rho g(h-z)$$From this, it follows that the pressure force on the fluid (per unit width) from the left boundary in your figure (acting to the right) is $$P=p_ah-\rho g \frac{h^2}{2}$$And the force (per unit width) from the air on the right boundary of your fluid (acting to the left) is just $p_ah$. So the net force on the fluid (acting to the right) is just $-\rho g h^2/2$. The remainder of your analysis is correct.