0
$\begingroup$

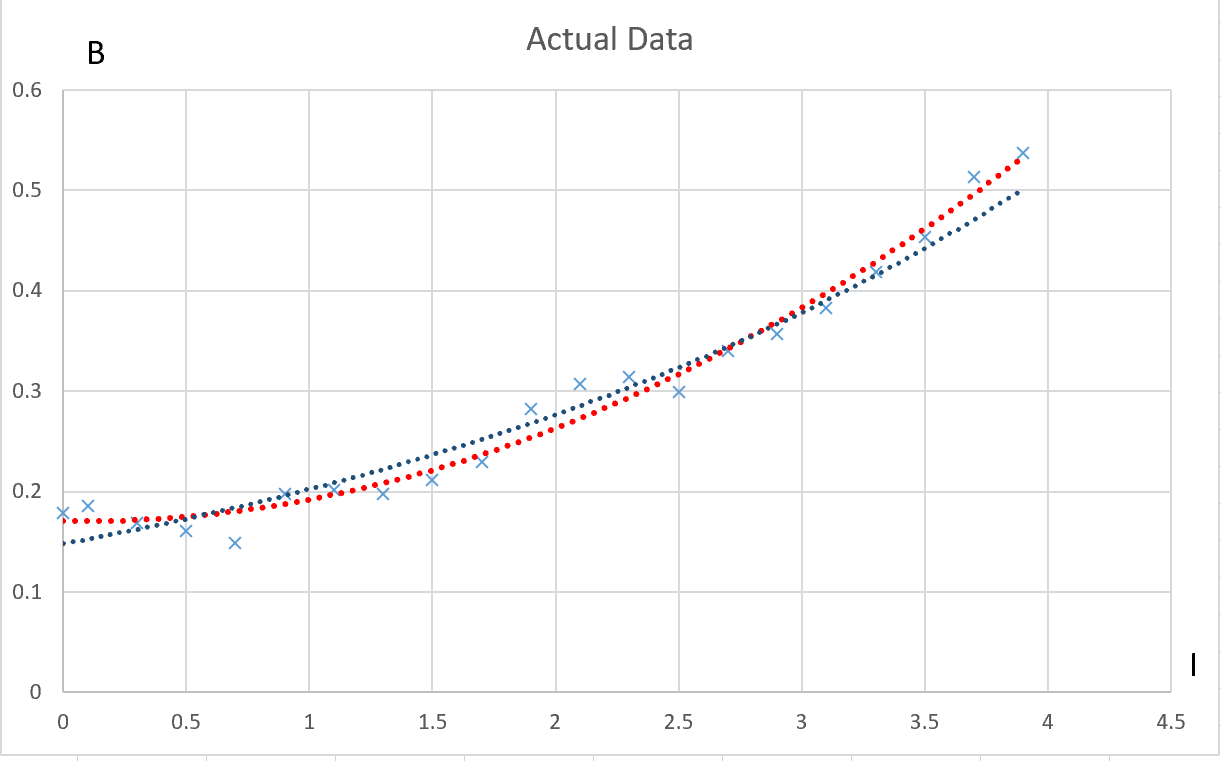
I in the experiment, I have tried to find a relationship between a quantity B and quantity I. First I plotted the raw data into a graph and tried different curve fits in excel. This is the graph that I got:

enter image description here

As you can see, B is plotted vertically and I is plotted horizontally. I also added the two curve fits; the red curve is a second-degree polynomial, and the blue curve is exponential. It would appear that the polynomial curve fits much better. However, then I did a trick we were taught. I assumed that the relationship would be of the form B=kI^c, where k and c are constants. Then I "took" the logarithms of both sides: lnB=ln(kI^c)=lnk + cln(I). If ln(I) is plotted against lnB, I get the following graph:

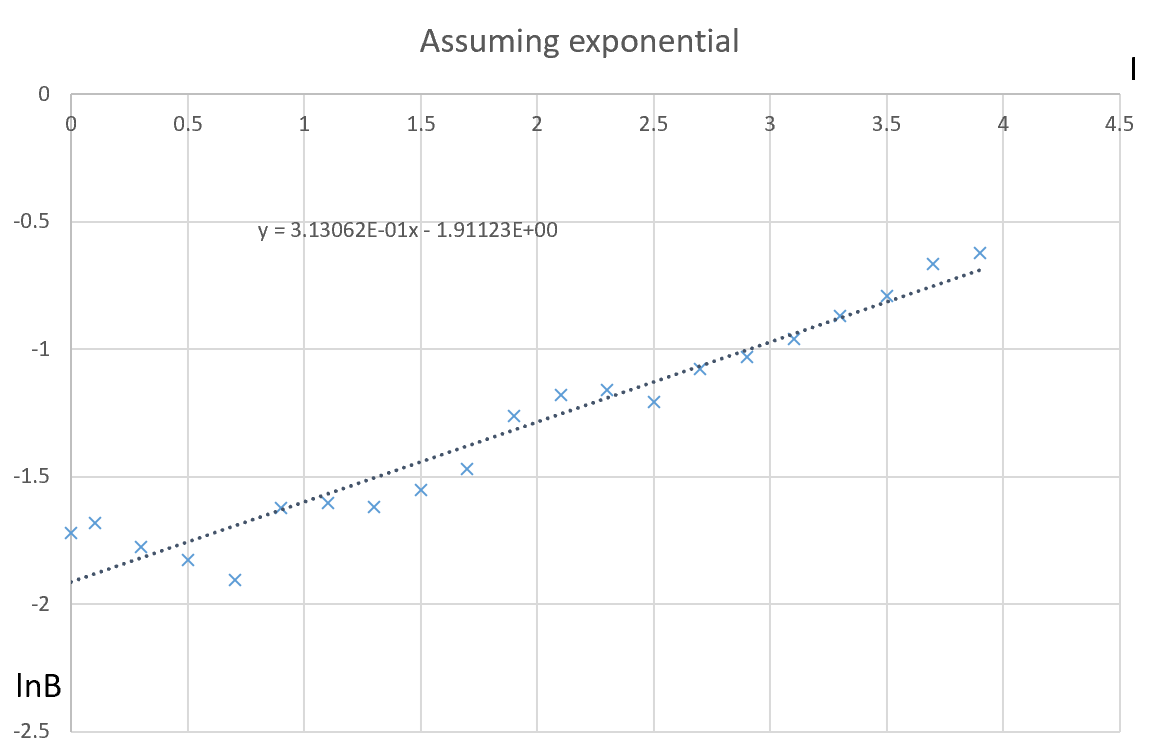
enter image description here

As you can see, this is nowhere close to a line, and what more it actually kinda looks like the original polynomial curve. This was what first got me to think that the relationship was exponential. When I did the same trick but assumed that B=e^(cI), therefore lnB=cI*lne. So I plotted I against lnB and I got this curve:

enter image description here

Although the trendline does not pass through all the points, the overall trend seems to be linear. But in the first graph, the polynomial seemed to be the clear favorite. Why? Which one should I go with?

Improve this question
$\endgroup$
8
  • $\begingroup$ Is $B$ magnetic field and $I$ current? $\endgroup$
    – Qmechanic ♦
    Commented Mar 1, 2020 at 19:59
  • $\begingroup$ Yes, I is current, but no, B is not a magnetic field. B is the decay constant in the equation Ae^(-Bt)cos(Ct+D)+E. I apologize I should have used a different letter, it did not occur to me that it could get confused with the magnetic field. The investigation was to determine how much retarding force can be generated using an electromagnet for different currents. $\endgroup$
    – NX37B
    Commented Mar 1, 2020 at 20:07
  • 1
    $\begingroup$ Note that $y(I)=a I^b$ is not a polynomial, it is a power law. A polynomial would be of the form $y(I) = a + b I + c I^2 + \ldots$. Performing that logarithm trick with a polynomial would only yield a linear fit if the polynomial had only a single term. $\endgroup$
    – J. Murray
    Commented Mar 1, 2020 at 21:12
  • $\begingroup$ Is there something I could do to check if there are any more terms? $\endgroup$
    – NX37B
    Commented Mar 1, 2020 at 21:58
  • $\begingroup$ Why do you need the relationship? Are you using it for interpolation in some engineering application? Or do you want to have an equation with a first-principled basis? Indeed, do you have a first-principled reason why B should depend on I? $\endgroup$
    – Jeffrey J Weimer
    Commented Mar 1, 2020 at 23:37

1 Answer 1

Reset to default
0
$\begingroup$

the blue curve seems to be an exponential curve like y=ae^(bx) so B=ae^cI ist the correct equation of your first curve, completely different fron B=aI^c so the second linearization is the only one which makes sense . To test the alternativ quadratique curve you could take B=cI^2 so plot sqrt(B) against I

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.