I did read the following questions: "Flux linkage inside of a conductor", "Derivation of self-inductance of a long wire" and "Trouble understanding fractional flux linkage"
The answers to them are based on the concept of "flux linkage":
$\lambda = N \cdot \phi$
where $\lambda$ is the flux linkage; $N$ is the number of turns; $\phi$ is the magnetic flux
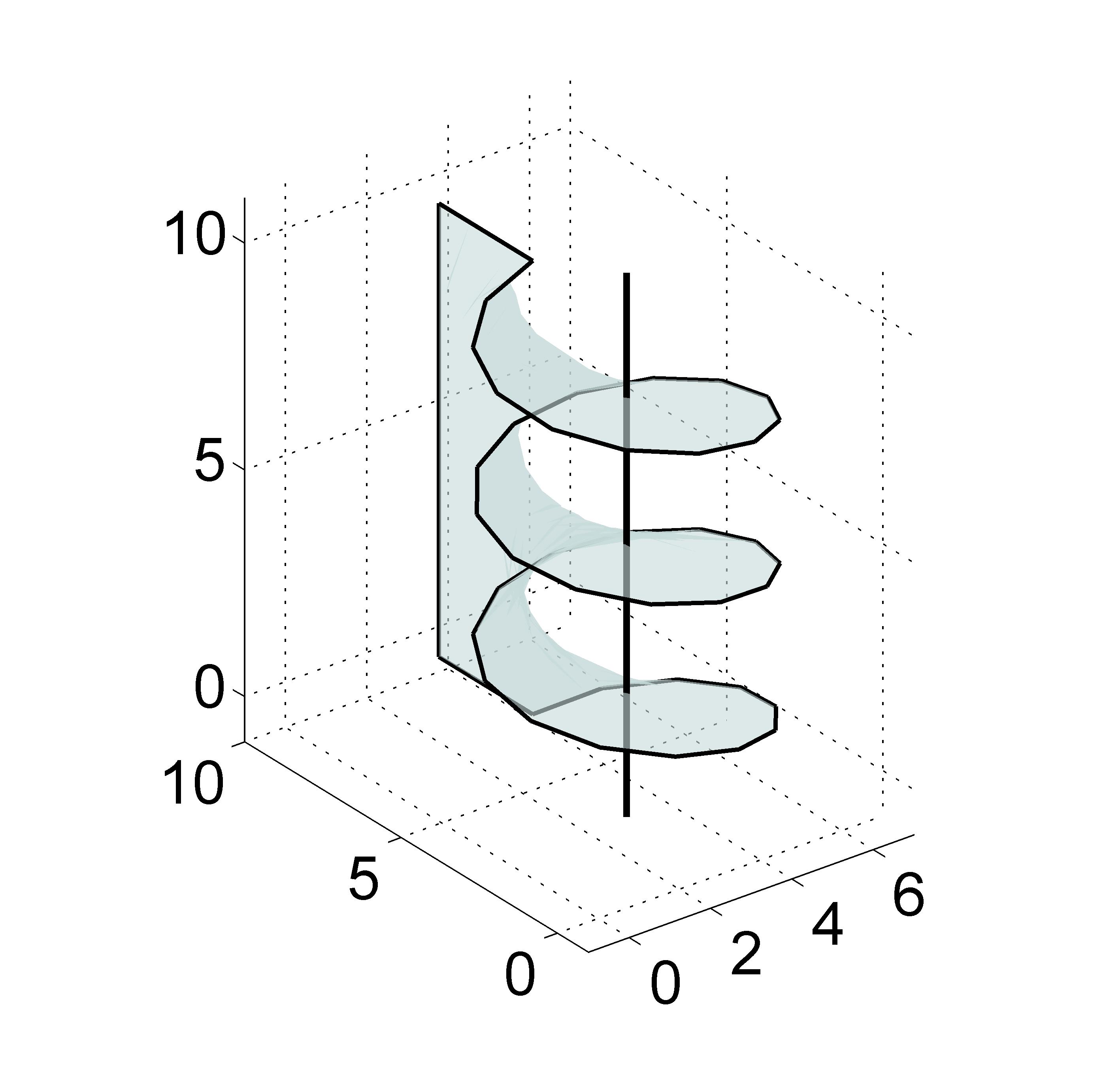
I always thought that flux linkage was an just an "artifice" used in order to avoid explaining integration surfaces like the picture below when calculating the magnetic flux.
If I am correct, there is a way of calculating the internal inductance of a long wire without the concept of flux linkage. The obvious attempt gives the wrong answer (same obtained by the authors of the aforementioned questions) and I have no idea how to make it right.