Thermal vibrations move at the speed of sound, but since they are move randomly, bouncing from atoms and dislocations, they spread out according to the (heat)diffusion equation: $$\frac{\partial T}{\partial t} = K\frac{\partial ^2T}{\partial x^2}$$ where $K=k/\rho C$ is the ratio of heat conductivity to the density and specific heat capacity. This does not have constant velocity solutions.
Borrowing from Carslaw and Jaeger 1959, the solution for an infinitely long rod initially at zero temperature with $x=0$ held at temperature $V_0$ for $t>0$ is: $$T(x,t) = V_0 \mathrm{erfc}\left(\frac{x}{2\sqrt{K t}}\right).$$ Here is a plot of some solutions for $V_0=1$:
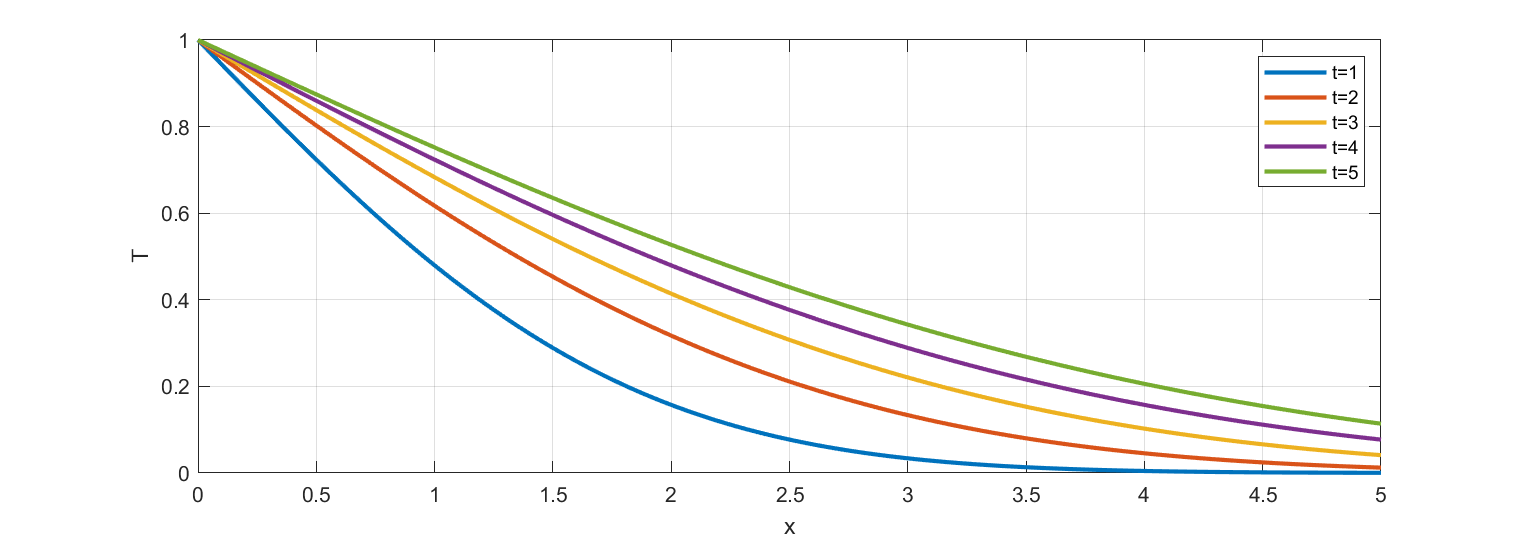Note how the point where the temperature is 1/2 (or any other level) at first moves fast to the right, and then slows down. This is because diffusion tends to move things a distance growing as $\sqrt{Kt}$ rather than at a linear speed.
An interesting special case is when there is a solid where the surface is heated by a periodic temperature $T(t)=\sin(\omega t)$. Then the temperature in the solid is a damped oscillation where the waves move with velocity $\sqrt{2K\omega}$. In a sense you can get very fast heat signals by having a large $\omega$, but the damping increases exponentially with the frequency so in practice rapid heat waves do not penetrate deeply into the solid.
In summary, heat moves with variable speed.
