0
$\begingroup$

Einstein used light signals to synchronize clocks in the same reference frame to the same time no matter the distance separation between those clocks to come up with some kind of perspective "present". But can the opposite be done? Can you use light signals from clocks in different reference frames to calculate what your proper time "present" was when your twin-paradox twin sent you a light signal with his proper time indicating the moment he sent it?

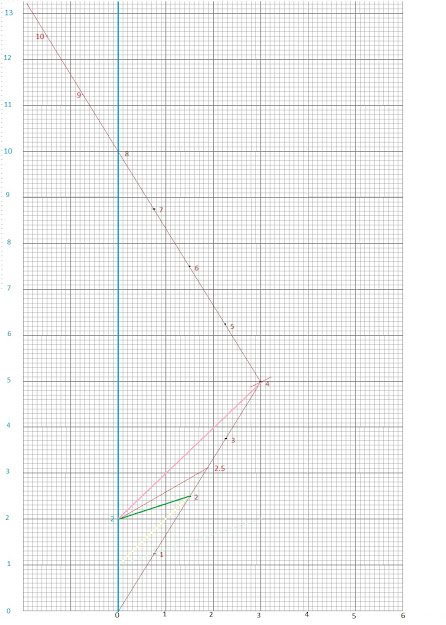
Below is a Minkowski diagram of a twin paradox example for Alice doing a round trip at 0.6c. Bob sends out a pink light signal to her when his proper time is 2. She receives it when her proper time was 4. She wants to figure out what was her proper time when he sent it.

enter image description here

From her perspective, Bob's light signal is sent when she is 2.5 and Bob's 3 (Bob) yr light signal was only 1.5 (Alice) years long from the time she's 2.5 until she receives it at 4. So her proper time was 4 - 1.5 (light travel time) - 0.5 (the relativity of simultaneity from when Bob actually sent the signal to when she saw him send it) = 2. Her proper time was 2 when Bob sent the signal so we draw a green proper time line of simultaneity to signify the answer.

Now I can figure out the rest of the answers for each light signal from Bob and Alice but my math method is not appreciated here. Does relativity have a method to calculate proper time simultaneity using light signals from each year in the diagram? You don't even need to do every year just from Bob = 5. The answer I get is Alice's proper time was 4.5 when he sent his light signal when his proper time was 5. The reciprocal also seems to be true, that if Alice sends out a signal at 4.5, Bob's proper time was 5 when she sent it.

For bonus points figure out the light travel time from Alice's perspective. The remainder should be her relativity of simultaneity of when she sees Bob release the light signal. I'm trying to figure that out myself right now.

Improve this question
$\endgroup$
8
  • $\begingroup$ What is your question? $\endgroup$
    – sammy gerbil
    Commented Jan 12, 2020 at 9:51
  • 1
    $\begingroup$ I've clarified and focused my question but it won't matter because I've never had a question reopened once it's closed. $\endgroup$
    – ralfcis
    Commented Jan 12, 2020 at 23:27
  • 1
    $\begingroup$ @ralfcis that it why it is important to write a question well the first time! You have been at this long enough to know what is expected. I have advised you on clarity and focus multiple times, and you have shown multiple times that you can do it. Just do it right first and let’s skip the perpetual drama $\endgroup$
    – Dale
    Commented Jan 13, 2020 at 0:49
  • 1
    $\begingroup$ When you know how to write a good question and don’t do it (as is the case with you) then it seems that you want your questions to be closed. Since that seems to be the case now I will let you know in advance that this is the last question of yours that I will ever vote to reopen. I think this is the third or fourth, and I tire of the drama $\endgroup$
    – Dale
    Commented Jan 13, 2020 at 1:16
  • 1
    $\begingroup$ Ok I have a fix for this. I will write my questions on my other forum first instead of vice versa. This will give the question time to percolate before I put it here. Oh while you're here, could you upvote the question as well? Just kidding. $\endgroup$
    – ralfcis
    Commented Jan 13, 2020 at 1:23

2 Answers 2

Reset to default
3
$\begingroup$

She receives it when her proper time was 4. She wants to figure out what was her proper time when he sent it.

Unfortunately, this is undefined. The proper time for an observer is only defined for events on the worldline of that observer. So Alice's proper time is only defined for events on Alice's worldline. The event when Bob sent the signal is an event that is not on Alice's worldline, so her proper time is undefined at that event.

At that event Bob's proper time would be defined, but any other time that would be defined at that event would be a coordinate time, not a proper time. What I believe that you want is the coordinate time in Alice's frame at that event. Alternatively, you may want the proper time on Alice's worldline at the same coordinate time as that event in some other frame besides Alice's frame. In either case, a coordinate time will be necessary and it will be necessary to uniquely identify the reference frame for that coordinate system. That reference frame will define the simultaneity convention for mapping events on Bob's worldline with the corresponding events on Alice's line.

Her proper time was 2 when Bob sent the signal so we draw a green proper time line of simultaneity to signify the answer.

Again, her proper time when Bob sent the signal is undefined, it is not 2.

However, there is a reference frame where the event that Alice's proper time was 2 (which defines a unique event on her worldline) is simultaneous with the event that Bob sent the signal. This reference frame is moving at v = 1/3 c to the right with respect to Bob and at v = 1/3 c to the left with respect to Alice. In that frame both Bob and Alice are moving at 1/3 c in opposite directions.

This reference frame is not anything special, it is just another reference frame like any other. However, it is the unique reference frame where these two events are simultaneous and it is also the unique reference frame where Bob and Alice are equally time dilated. Since Bob sends the signal when his proper time is 2 then the reference frame where that event is simultaneous with Alice's proper time being 2 is clearly the reference frame where they are equally time dilated.

Does relativity have a method to calculate proper time simultaneity using light signals from each year in the diagram?

No, "proper time simultaneity" is an undefined term, and I would not recommend trying to propose a personal definition for the term. "Proper time" is an invariant that is defined only on a given worldline and "simultaneity" is frame-variant and is defined over all spacetime so "proper time simultaneity" is nearly as oxymoronic a name as could be conceived.

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ I did not see this answer coming but it is a great answer. As you may know, many of my previous questions were about this and I think I finally have closure. $\endgroup$
    – ralfcis
    Commented Jan 13, 2020 at 21:51
  • $\begingroup$ If my other question about "proper age difference" gets opened up I can correct it based on the info here. I just need to replace everywhere I use the adjective "proper" with the term "half-speed perspective". I can also go back and correct any wrong terms in my other questions can't I? Is half-speed or middle or balanced perspective an acceptable term because I will use it quite often in future questions. $\endgroup$
    – ralfcis
    Commented Jan 14, 2020 at 0:58
  • $\begingroup$ I think it would be fine, early in the question, to say “I will use the term ‘half speed perspective’ to refer to the reference frame where Alice and Bob are traveling in opposite directions at the same speed”. $\endgroup$
    – Dale
    Commented Jan 14, 2020 at 1:14
  • 1
    $\begingroup$ After digging for info to answer my previous question I've come up with the perfect term for everything previously labelled as "half-speed". This sentence on Loedel diagrams in wiki, "Suppose there are two collinear velocities v and w. How does one find the frame of reference in which the velocities become equal speeds in opposite directions?" So half-speed is the Loedel reference frame so I'll use Loedel perspective, Loedel simultaneity, and Loedel velocity in all my future posts and expect everyone will know what I'm talking about. Case closed. $\endgroup$
    – ralfcis
    Commented Jan 15, 2020 at 0:20
  • $\begingroup$ So I guess the title of this question should be changed to, "In the twin paradox, can light signals be used between twins to figure out what their times were from the Loedel perspective when the light signals were sent? I guess I'll have to open this as a separate question. I'm working on figuring out the answer first. So far it's not easy to solve. $\endgroup$
    – ralfcis
    Commented Jan 16, 2020 at 18:57
1
$\begingroup$

Suppose you have three inertially-moving clocks A, B and C.

Albert always stays half way (in his own frame of reference) between A and B, and confirms A and B always show him the same time.

Barclay always stays half way (in his own frame of reference) between B and C, and confirms B and C always show him the same time.

Albert and Barclay argue because each thinks the other is wrong. Albert does not see clocks B and C keeping the same time, and Barclay does not see clocks A and B keeping the same time.

Callum keeps quiet. He is always half way between C and A but he wants a peaceful life.

Improve this answer
$\endgroup$
7
  • $\begingroup$ Sorry why would Albert and Barclay argue about something they can't see? $\endgroup$
    – ralfcis
    Commented Jan 11, 2020 at 17:48
  • $\begingroup$ Surely Albert can see clock C and Barclay can see clock A, or have I missed something in the question? $\endgroup$
    – DrC
    Commented Jan 11, 2020 at 18:04
  • $\begingroup$ Oh, ok. These green lines of simultaneity are just segments of the proper time hyperbola. So all 3 segments that the boys are at the center of are different and Albert's doesn't extend to C and Barclay's doesn't extend to A. So you are correct but I don't see this as an answer, more a side note. $\endgroup$
    – ralfcis
    Commented Jan 11, 2020 at 18:13
  • $\begingroup$ Agreed - but I'm not sure where you are trying to take your analysis. While Albert sees clocks A and B keeping time together, time now is a local concept except where you use Einstein's synchronisation to extend it to an inertial frame. While clocks A and B are seen by Albert to be keeping time together, they will not be showing him the same time as other clocks in frame A or frame B, because there is are different x- terms in the two Lorentz transformations - the relativity of simultaneity. $\endgroup$
    – DrC
    Commented Jan 12, 2020 at 0:31
  • $\begingroup$ Albert's proper time perspective allows me to easily calculate A's perspective of B's time and B's perspective of A's time. In my example when Bob's time is 2.5, his perspective simultaneity of Alice's time is 2. When Alice's time is 2.5, her perspective of of Bob's time is 2. The question began with how to tell the other's time using light signals and ended up using only Albert's proper time perspective to easily convert what Bob and Alice's perspective times of each other were. $\endgroup$
    – ralfcis
    Commented Jan 12, 2020 at 2:11

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.