Work done by adiabatic pumping ideal gas between two vessels

Question

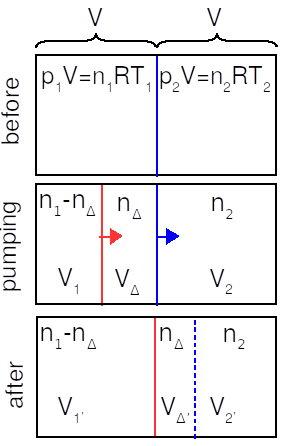
Lets consider I have two vessels of the same volume $V$ each of which contains ideal gas at different pressures and temperatures $(p_1,T_1)$, $(p_2,T_2)$. I pump certain amount of the gas $ n_\Delta$ from one vessel to the other. What is the work required for the pumping (assuming adiabatic process, without friction or other losses).

Is there some elegant solution or simple equation using only pressure, temperatue and molar amount?

---

Not elegant solution:

We can use well known formula for adiabiatic process

$p V^\gamma = const = K$

We calculate volume fraction of each gas before and after from

$pV=nRT$

so we calculate molar amounts in each vessel before pumping

$n_1 = p_1 V /( R T_1 )$

$n_2 = p_2 V /( R T_2 )$

and attribute volume fraction to each molar amount ($V \propto n$)

$V_1 = V(n_1-n_\Delta)/n_1 $ ... gas left in vessel 1

$V_2 = V$ ... gas already present in vessel 2

$\Delta V = V n_\Delta/n_1 $ ... gas pumped between vessels

Than we can calculate adiabatic compression/expansion for each volume independently $[V_1 \rightarrow V_1'; V_2 \rightarrow V_2'; V_\Delta \rightarrow V'_\Delta]$. To do so we have to determined final volume and pressure.

After the compression we have

$V_1' = V$

$V_2' + V'_\Delta = V $

Therefore

$V'_\Delta = V n_\Delta /(n_2+n_\Delta) $

$V_2' = V n_2/(n_2+n_\Delta) $

Now we know volume of each fraction of gas, so we can use $p V^\gamma = const$ and

$W = (p'V' - pV)/(1-\gamma) $

... but this starts to be rather complicated ... so I wonder if there is more elegant way

NOTE : In addition I'm not sure about entropy when the temperature of pumped gas after compression is different than the gas originally in vessel ( => mixing gas of different temperatures)

EDIT : To make is a sketch of the pumping translated to compression by imaginary piston

Answer 1

OK. This is a two-step process. In the first step A the red wall is inserted (materializes) and the blue wall is removed (de-materializes). In the 2nd step B, the gas in the left compartment is caused to expand adiabatically and reversibly to the initial volume V while the gas in the then right compartment is caused to be compressed adiabatically and reversibly to the initial volume V.

Let the subscript A represent the conditions after step A and the subscript B represent the conditions after step B, and let the subscripts 1 and 2 represent the parameters for compartments 1 and 2.

STEP A

At the end of Step A, the number of moles in each of the two compartments is: $$n_{1A}=n_1-\Delta n$$ $$n_{2A}=n_2+\Delta n$$

The change in internal energy for the system is zero during step A. So, $$n_{1A}C_v(T_{1A}-T_{ref})+n_{2A}C_v(T_{2A}-T_{ref})=n_1C_v(T_1-T_{ref})+n_2C_v(T_2-T_{ref})$$where $T_{ref}$ is an arbitrary reference temperature. Since there is no change in the temperature in the left compartment during Step 1, $$T_{1A}=T_1$$ From these equations, it follows that $$T_{2A}=\frac{(\Delta n) T_1+n_2T_2}{\Delta n+n_2}$$ The final volumes and pressures in the two chambers after this step are: $$V_{1A}=V\left(1-\frac{\Delta n}{n_1}\right)$$$$V_{2A}=V\left(1+\frac{\Delta n}{n_1}\right)$$ $$P_{1A}=P_1=\frac{n_1RT_1}{V}$$ $$P_{2A}=\frac{n_{2A}RT_{2A}}{V_{2A}}$$

STEP B For the adiabatic reversible compression and expansion in Step B, $$V_{1B}=V_{2B}=V$$$$P_{1B}=B\left(\frac{V_{1A}}{V}\right)^{\gamma}=P\left(1-\frac{\Delta n}{n_1}\right)^{\gamma}$$ $$P_{2B}=P_{2A}\left(\frac{V_{1B}}{V}\right)^{\gamma}$$ I leave it up to you to get the work done on each gas and the net work done by the pump in this step.