What interpretation of quantum mechanics do Conditional Probabilities feature in and how is it different from the Many-worlds Interpretation?
The definition of the word interpretation in quantum physics means that the words and possibly deterministic mathematics describing the specific interpretation may be different than the mainstream interpretation, but the end of the calculations are the same , modeling the physics results. For example the Bohmian mechanics has deterministic functions but the final result is to reproduce the probability amplitudes of the Copenhagen interpretation( at least for non relativistic states).
The many worlds interpretation comes from the quantum field theory mathematics of the path integral method of calculating measurable quantities.
The path integral formulation of quantum mechanics is a description of quantum theory that generalizes the action principle of classical mechanics. It replaces the classical notion of a single, unique classical trajectory for a system with a sum, or functional integral, over an infinity of quantum-mechanically possible trajectories to compute a quantum amplitude.
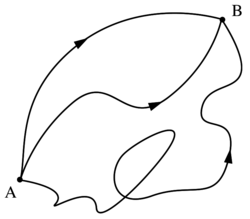
These are just three of the infinitely many paths that contribute to the quantum amplitude for a particle moving from point A at some time t0 to point B at some other time t1
In the mainstream interpretation this is a mathematical tool to calculate results that can be predicted by experiment. In the same way that wavefunctions are not considered measureable by themselves but posited as giving rise to probability amplitudes that can give measurable results, or that virtual particles are just mathematical tools, these paths are just considered mathematics.
The many worlds interpretation considers the paths real, creating infinite worlds with each path change. This makes no difference to the numbers coming out of the calculation, so it is an interpretation.
Once one starts to believe in the reality,i.e. the possibility of measuring a different world, it becomes a different theory that has to be validated for the new predictions it is making.
I read the paragraph of Grifith you are quoting, and he is saying the same thing in different words. He believes that the path integral method of calculating quantum mechanical probabilities is a cleaner way of describing the theory, but he also says that all interpretations lead to the same results.
The "conditional part" comes from probability theory, and probailities are the same in quantum and classical dynamics.
In probability theory, conditional probability is a measure of the probability of an event occurring given that another event has (by assumption, presumption, assertion or evidence) occurred.1 If the event of interest is A and the event B is known or assumed to have occurred, "the conditional probability of A given B", or "the probability of A under the condition B", is usually written as P(A | B), or sometimes PB(A) or P(A / B).
All boundary conditions set for a quantum mechanical problem, are conditions which will affect the wave functions and the path integrals .
The reason Griffiths likes the path integral method is that one can ignore the continuous misinterpretation of "collapse". The wave function is not a balloon to collapse. In physics the word means that "new wavefunctions have to be calculated". That is why he likes the path integral method, because the interactions are already in the calculation.

