0
$\begingroup$

First, let's define what I mean by a Carnot cycle. I call any reversible cyclic transformation of any system (composed of any working substance) going through two distinct isotherms via two adiabatic curves, a Carnot cycle; and the cycle run in the direction to obtain positive work in one cycle is what I call a Carnot engine working between the two temperatures of the two isotherms.

Now the Carnot Theorem is the following:

Any engine working between two temperatures is less efficient than the Carnot engine working between the same temperatures.

Question 1: What does"an engine working between two temperatures" mean? I think that this engine, during its cycle, exchanges heat only with the heat sources (at the two temperatures) and nowhere else. And hence, this uniquely is a Carnot cycle, if reversible. Am I correct?

Question 2: Does the Carnot Theorem apply only to the above-mentioned cycles, or does it make a statement about any engine whose maximum and minimum temperatures during one complete cycle coincide with the isotherm temperatures of the Carnot cycle working between those temperatures?

Improve this question
$\endgroup$
17
  • $\begingroup$ It appears that you accepted my answer and thenunaccepted it. May I ask why? $\endgroup$
    – Bob D
    Commented Dec 6, 2019 at 11:22
  • $\begingroup$ @BobD Was in the middle of commute. Now I can focus. I really appreciate the answer and do agree with what you say. But can you please provide me some hardcore evidence that what you say is really correct, which I do believe is. Cuz nowhere is this explicitly mentioned. $\endgroup$
    – Atom
    Commented Dec 6, 2019 at 11:27
  • $\begingroup$ Not sure what you think would constitute “hard core evidence”. I’m on my mobile device now. When I get to my computer I’ll look for some examples $\endgroup$
    – Bob D
    Commented Dec 6, 2019 at 11:33
  • 1
    $\begingroup$ see physics.stackexchange.com/questions/300347/… $\endgroup$
    – hyportnex
    Commented Dec 6, 2019 at 12:20
  • 1
    $\begingroup$ see page 39 "If there are several cyclic heat engines, some of which are reversible, operating around cycles between the same temperatures t1 and t2 , all the reversible ones have the same efficiency, while the nonreversible ones have efficiencies which can never exceed the efficiency of the reversible engines." So this would be the same four legs as of a Carnot cycle but on page 46 he proves the Clausius inequality (Eq 61) for any type of cycle and the two together will get your answer to your problem. $\endgroup$
    – hyportnex
    Commented Dec 6, 2019 at 12:54

1 Answer 1

Reset to default
1
$\begingroup$

I believe the two temperatures referred to in the Carnot Theorem are the maximum and minimum temperatures of the range of temperatures between which the engine operates.

In the case of the Carnot heat engine it operates between two fixed temperatures and not a range of temperatures. If a heat engine operates over a range of temperatures instead of fixed temperatures then the value for $T_H$ In the Carnot efficiency equation would be some mean value less than the maximum and the value of $T_L$ would be some mean value greater than the minimum. That makes the ratio $\frac{T_L}{T_H}$ larger and the efficiency less than the Carnot heat engine.

UPDATE:

This updates my answer based on our exchange of comments since my original answer.

Question 1: What does"an engine working between two temperatures" mean? I think that this engine, during its cycle, exchanges heat only with the heat sources (at the two temperatures) and nowhere else. And hence, this uniquely is a Carnot cycle, if reversible. Am I correct?

I believe it means an engine that takes heat from a single temperature reservoir to produce work in an isothermal expansion process and then rejects heat to another single (lower temperature) reservoir in an isothermal compression process.

However, it is only uniquely a Carnot cycle if the isothermal processes as well as the adiabatic processes that link them, are reversible. This requires that (1) the temperature differences between the temperature reservoirs and the working fluid during the isothermal processes are infinitesimally small and (2) the pressure differences between the system and surroundings during the adiabatic processes are infinitesimally small as well and (3) no friction is involved in any of the processes.

Question 2: Does the Carnot Theorem apply only to the above-mentioned cycles, or does it make a statement about any engine whose maximum and minimum temperatures during one complete cycle coincide with the isotherm temperatures of the Carnot cycle working between those temperatures?

As I see it, the only way a reversible cycle whose maximum and minimum temperatures coincide with the isothermal temperatures of the Carnot cycle can have an efficiency less than the Carnot cycle is if the heat exchanges are occurring over a range of temperatures between the max and min, and not just two fixed temperatures. Because all reversible cycles operating between the same two fixed temperatures necessarily have the same efficiency, per the theorem.

The reversible Rankine power cycle is an example of a reversible cycle operating in a range between a maximum and minimum temperature, as opposed to operating between a single high and low temperature reservoirs. By using mean temperatures in the Carnot efficiency equation it shows how the efficiency of the reversible Ranking cycle operating between the same max and min temperatures as the Carnot cycle is less efficient. The example is worked out in the following link from the MIT.edu website: http://web.mit.edu/16.unified/www/SPRING/propulsion/notes/node65.html

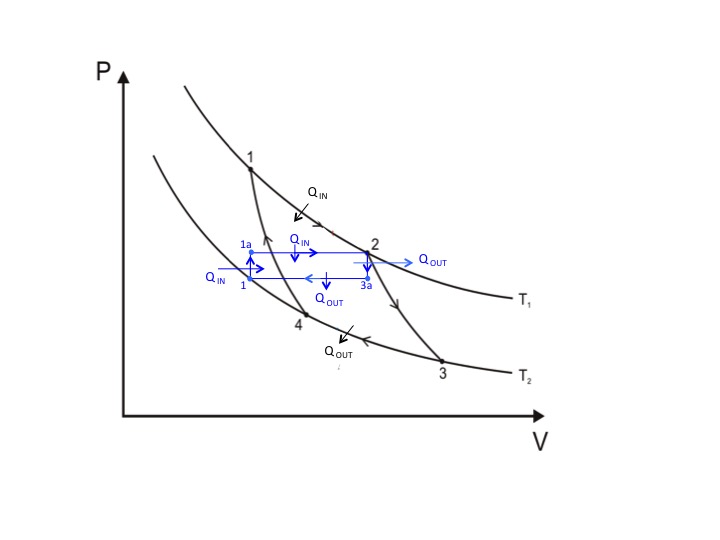
The diagram below shows a reversible cycle consisting of two reversible isobaric processes and two reversible isochoric processes, shown in blue, superimposed on a Carnot cycle. The cycle operates between the max and min temperatures of the Carnot cycle, $T_1$ and $T_2$, respectively. But the reversible heat transfers occur over a series of thermal reservoirs between the max and min and not just a single high and low temperature reservoir. To determine the efficiency using the Carnot efficiency equation, one would have to use the mean values of the temperatures. This results in a lower efficiency than the Carnot efficiency.

Hope this helps.

enter image description here

Improve this answer
$\endgroup$
9
  • $\begingroup$ And if you look at the derivation of this given by Fermi in his Thermodynamics, you'll see that he assumes that heat is exchanged only with the two reservoirs at two temperatures. So that derivation doesn't work for what you say. $\endgroup$
    – Atom
    Commented Dec 6, 2019 at 11:40
  • $\begingroup$ Found anything @BobD? $\endgroup$
    – Atom
    Commented Dec 6, 2019 at 12:45
  • $\begingroup$ @Atom so far nothing that contradicts my analysis but it’s hard to read on my phone. I’ll finish it on my computer and got back to you. $\endgroup$
    – Bob D
    Commented Dec 6, 2019 at 12:53
  • $\begingroup$ the temperatures in Carnot's theorem are not the temperatures at which "the engine operates" as those are not really well defined for a complex system but rather the temperatures at which heat is exchanged with a heat source or a heat sink, and that temperature is well-defined for it is the temperature of the thermostat. $\endgroup$
    – hyportnex
    Commented Dec 6, 2019 at 12:58
  • $\begingroup$ @Atom I read the section. I could not locate your quote of the Carnot Theorem in Fermi. If you can give me the specific location it would be helpful. The closest I could come is the statement he makes in italics on PDF page 46. Is this what you are referring to? $\endgroup$
    – Bob D
    Commented Dec 6, 2019 at 14:41

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.