What does it means that a particle with mass can be a wave without mass? [closed]

Question

I read that electron can exhibit particle and wave like property, but in particle form it has mass then when in wave form... Where did it mass go?

Answer 1

For waves, "mass" is just finite frequency at zero wave number. The free particle dispersion relation (with $\hbar = c = 1$) is:

$$ \omega = \sqrt{\omega_0^2 + ||\vec k||^2}$$

so at zero wave number $\omega = \omega_0 > 0$.

If we multiply by $\hbar$ and insert $c$:

$$ E = \sqrt{(\hbar\omega_0)^2 + (||\vec p||c)^2}$$

so at zero momentum:

$$E = \hbar \omega_0$$

Instead of talking about an object's frequency at zero wave number, we prefer "mass" (for various reasons):

$$E = \hbar \omega_0 = mc^2$$

Note that in this picture, mass isn't "stuff" and it's not equivalent to energy, it is energy. The fields don't have "stuff", they have conserved quantities like lepton number, so there is no concern about "where does the mass go".

Answer 2

Your intuition about what "mass" is is what's causing your problem. If you try and take mathematical descriptions of the world too literally you will hit a dead end pretty fast. In this example you're right in thinking that given your usual understanding of what mass is it seems strange that a wave would have such a property since we usually consider mass to be localised and yet waves are somehow spread out over space, making their definition of mass a little more abstract.

We could sit here and derive the concept of mass in a number of different ways, but its exact definition will always depend on how you approach it. Remember that all theories in physics are just abstract mathematical models at their core, they don't tell us the true nature of anything they are just ways of predicting the future based on observations of the past.

I hope this isn't an unhelpful answer, but the question you're asking doesn't have a solution that doesn't immediately open up a million other questions.

Answer 3

I read that electron can exhibit particle and wave like property,

The crucial term is "property". All elementary particles have a fixed mass, given by the length of the four vector that is defined by the energy and momentum they carry. and

In quantum mechanics all particles , composite or elementary, display wave properties in their interactions. Particles have to be described with the mathematics of quantum mechanics, and the wave property comes in the probability distribution of the particles interacting with the same energy and momentum and boundary conditions. It is the probability distributions that wave.

but in particle form it has mass then when in wave form... Where did it mass go?

The particle always has its mass when it interacts, and the mass enters the quantum mechanical equations that give the probability of interaction. This is fundamental in the postulates of quantum mechanics,

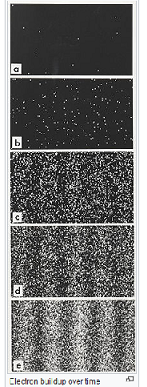
This is seen clearly in the experiment "single electrons going through a double slit , with definite width and distance apart".

The top frames show the particles adding one by one, to the bottom distribution which shows interference effects expected from waves! This is what is meant by the double nature. The accumulation of data shows it, not the individual particle which keeps its mass intact. The mass enters the differential equations that can explain frame e.

This brings us to thetitle:

What does it means that a particle with mass can be a wave without mass?

It is a misconception. The probability of detecting ( through interactions which are the only way to "see" a particle) depends on a wave equation, not the energy and momentum of the particle, which define its mass always .

Answer 4

Classical,non-relativistic, wave mechanics describes the mechanics of the wave associated with a moving particle of mass m , velocity v,i.e. momentum p=mv. You can find the momentum of the particle through the linear momentum operator applied to the wave function but naturally there is no mass operator.A mass at rest is not recognized in quantum mechanics.