Feynman's analogy about Snell's law doesn't always work

Question

Today I learned a feynman analogy about snell's law, but it didn't seem right and I would like to prove it. For this reason, I have tried to prove its falsehood and I would like to know if my analysis is correct or I have failed at something. Thanks in advance for taking the time to read and respond.

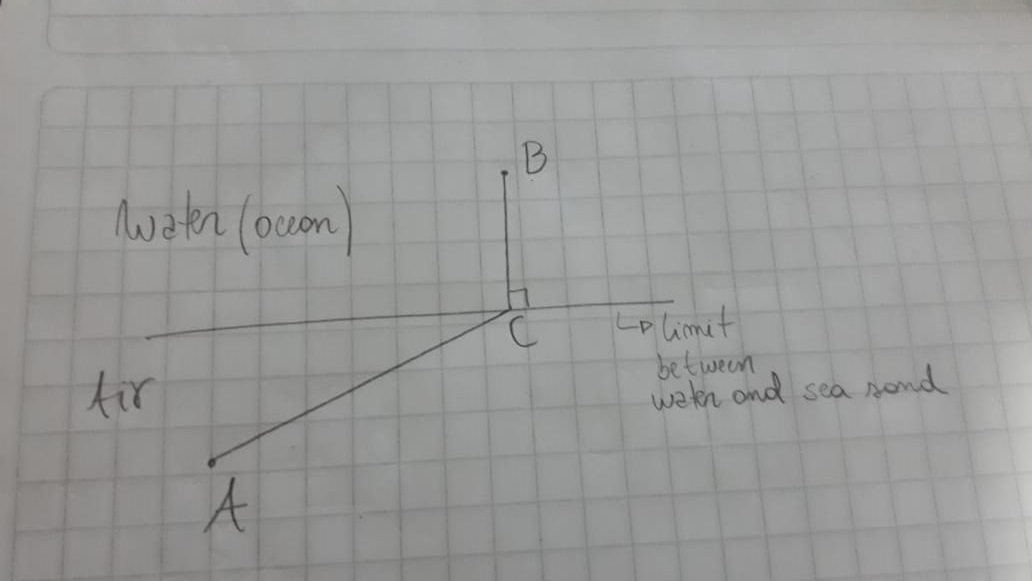
Feynman made an analogy about snell's law. It consists of two mediums being replaced by sand and water. If you are in the sand and would like to get as quickly as possible to a point in the sea, you should not go in a straight line, if not, try to travel the greatest distance in the medium in which the speed is greater (in this case in the air) and travel the shortest distance in the medium in which the speed is lower (in this case the water).

But I had a thought that this analogy is not always fulfilled, but only fulfilled when the "speed gained" compensates for the distance that has been traveled (since it has NOT gone in a straight line).

The first thing is to see how feynman's analogy would work.

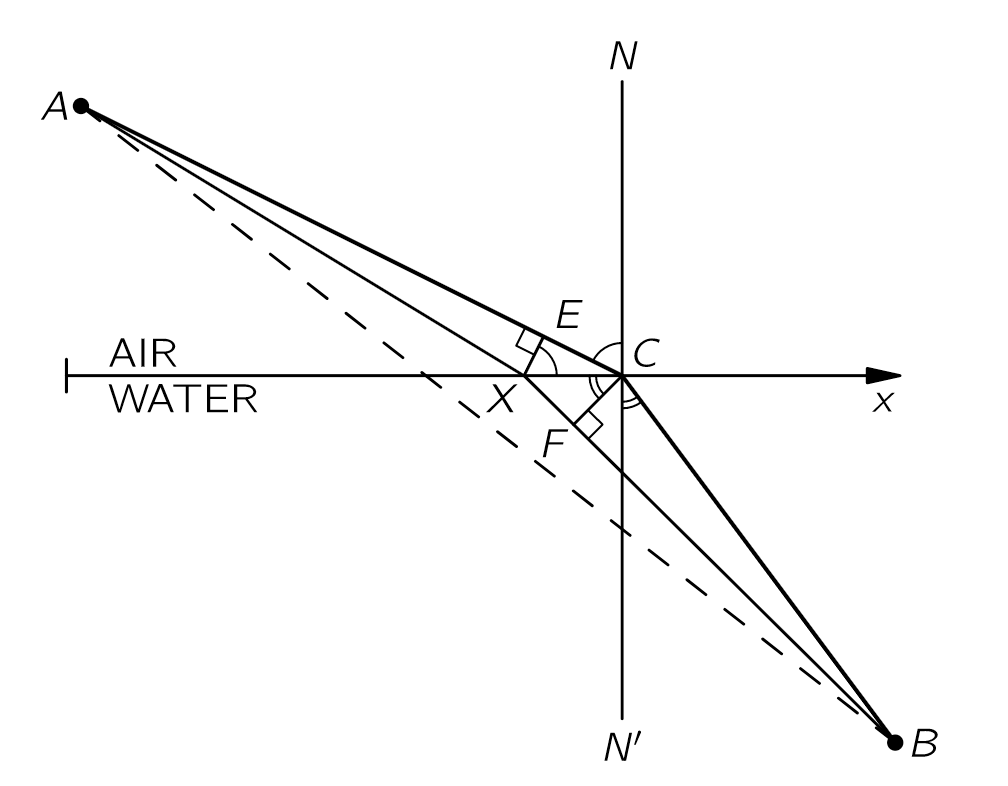
Since the shortest distance in the water must be traveled, we draw a perpendicular from the point in the sea (let's call this point $B$) to the limit between the sea and the sand (let's call this point $C$). Then a line is drawn from the point in the sand (let's call this point $A$) to the same point $C$. As drawn in this diagram:

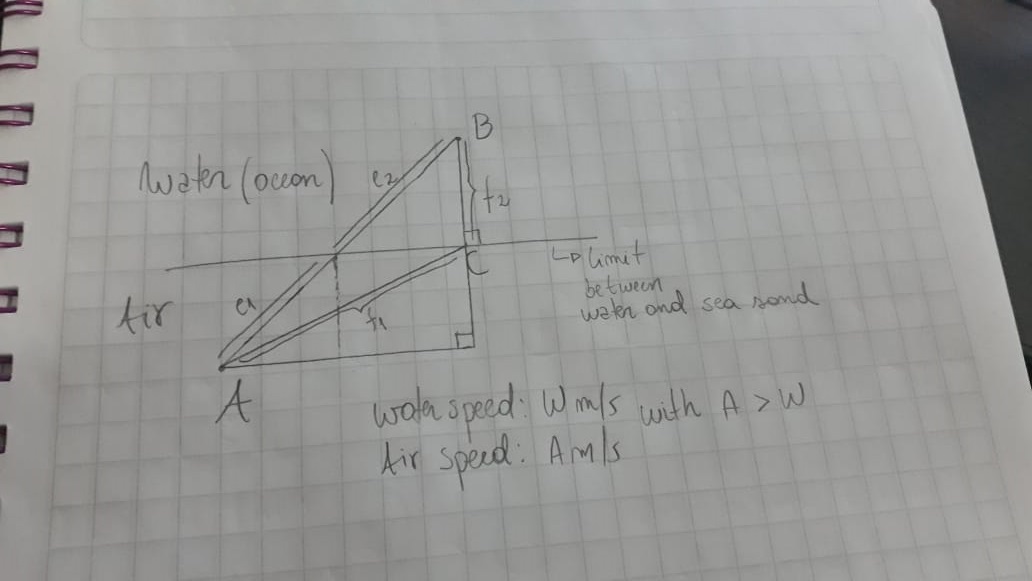
My idea will be to look for the necessary conditions, so that traveling in a straight line is faster than traveling as indicated by the Feynman analogy.

I create some segments, where $e_1, e_2, f_1, f_2$ are distances in meters.

The speed in the water is $W$ m/s and in the air is $A$ m/s, where the speed in the air is higher than the speed in the water, i.e $A > W$.

In addition to properties of triangles, we can establish certain inequalities between the distances created.

$f_1 > e_1$ and $f_2 < e_2$

We can say that if the time it takes for Feynman's analogy is longer than the time it takes for my counterexample, then my counterexample is faster.

Feynman's analogy time: $\frac{f_1}{A} + \frac{f_2}{W} = \frac{f_1 \cdot W + f_2 \cdot A}{AW}$ seconds.

Counter example time: $\frac{e_1}{A} + \frac{e_2}{W} = \frac{e_1 \cdot W + e_2 \cdot A}{AW}$ seconds.

So i need that, $f_1 \cdot W + f_2 \cdot A > e_1 \cdot W + e_2 \cdot A$.

Since $f_1 > e_1$ i can write $f_1 = e_1 + c$ with $c$ some positive number.

Since $f_2 < e_2$ i can write $f_2 = e_2 - q$ with $q$ some positive number.

replacing in the inequality $(e_1 + c) \cdot W + (e_2 -g) \cdot A > e_1 \cdot W + e_2 \cdot A$.

To get: $c\cdot W > g \cdot A$

So, the analogy does not work when this inequality is fulfilled.

Some example, $e_1 = 12, e_2 = 13, f_1 = 24, f_2 = 11, A = 3, W = 2$

Answer 1

Your first drawing (minimizing the distance traveled in the water) is not what Feynman is suggesting. The path that actually minimizes the travel time between the two points has you enter the water at a point between your point C and the point where your straight line path enters. I refer you to the following figure taken from the Feynman lectures. (Vol I, Chapter 26)

It is a straightforward exercise in calculus to make the point where you enter the water arbitrary and then minimize the travel time to find the optimal path. You will then have derived Snell's law from Fermat's principle.

Answer 2

This is supposed to be a comment. He never said that the shortest distance must be traveled in the shower medium, he said that lesser distance must be travelled in the shower medium

Answer 3

You’ve constructed a straw man: yes, you’ve found a better path than f1;f2, but f1;f2 isn’t how light would travel. The f1 and f2 you’ve drawn clearly don’t obey Snell’s law.

It’ll take a bit more trigonometry than I have time to typeset here, but you can construct the actual light path for f1;f2 using Snells law, then use your method to show there isn’t a better path.