4
$\begingroup$

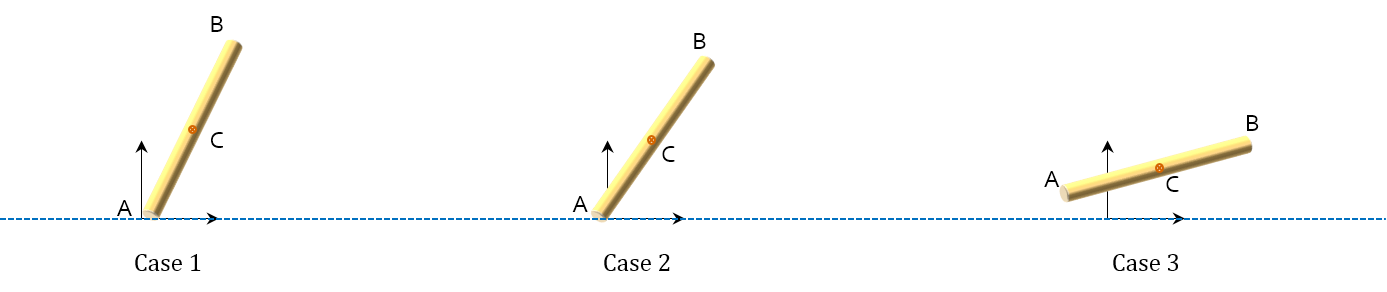
Consider an initially vertical thin rod of mass $m$ and length $L$ (i.e. with one end touching the ground) that is allowed to fall. Assume no friction between the rod and the ground and that gravity is uniform. Will one end of the rod always remain in contact with the ground?

I've borrowed the graphic above from this post which seems relevant enough to what my question is asking. I tried to condense the main features of my question into the more textbook-y sounding version above, but the gist of it is

  1. Under what conditions can a sliding rod enter into case 3 (in which the normal force vanishes)?

  2. Additionally, can a falling rod with no initial velocity (just outside of unstable equilibrium) ever reach that state?

  3. What would the locus/equation of motion of some point initially $y$ off the ground look like?

I tried to approach this problem in a couple of ways, but I kept getting stuck at finding equations of motion for some non-CM point. I'm comfortable with use of formalisms for the more concrete questions (my initial method was finding the angle for which the constraint $\lambda(t)$ equals zero), but I think the bulk of it might be more intuitive with forces/torques on the center of mass.

If you'd like, I can add some of my non-constructive attempts at answering these questions, but really I'm just curious as to the intuition behind it, since some physical experimentation makes it seem like case 3 never happens.

Thanks!

Improve this question
$\endgroup$
2
  • $\begingroup$ In an ideal free fall, the left end will never suddenly lift up relative to the centre. All parts of the stick fall equally fast. When the ground is in the way, ideally the same. But, you might wish to consider elastic "bouncing" off of the ground, in which case scenario 3 is possible - but since we are not talking about an impact with the ground, but something standing and falling over, this wouldn't be the case. $\endgroup$
    – Steeven
    Commented Nov 12, 2019 at 8:26
  • $\begingroup$ Point A, B & C should fall at the same rate, T&C applies. $\endgroup$
    – user6760
    Commented Nov 12, 2019 at 8:42

1 Answer 1

Reset to default
0
$\begingroup$

In falling suppose that the rotational speed of the rod about its centre of mass reaches a value so that the end of the rod leaves the surface.
At that "instant" there is then no net torque on the rod about its centre of mass and so the speed of rotation of the rod is now constant but the downward speed of the centre of mass of the rod is still increasing.
At the next "instant" of time the end of the rod hits the surface and the process (might) repeats itself.
This "hand waving" argument leads me to believe that the rod will always stay in contact with the surface.

I did an experiment but not having a frictionless surface or a suitable lump of ice, I used $30\,\rm cm$ and $60 \,\rm cm $ steel and $1\,\rm m$ wooden rulers falling onto a stainless steel sink surround surface.
There was no evidence of the ends of the rulers leaving the surface as they fell.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ I have made a suggestion as to the answer and will be extremely interested to know what is the error(s) that I have made to warrent the -1. $\endgroup$
    – Farcher
    Commented Nov 12, 2019 at 9:58

Not the answer you're looking for? Browse other questions tagged or ask your own question.