1
$\begingroup$

Diagram

By book defines SHM as

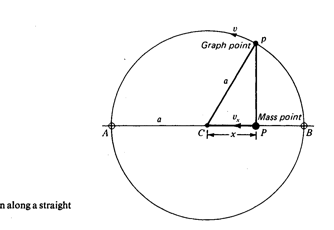
Simple harmonic motion is defined as the projection on any diameter of a graph point moving in a circle with uniform speed.

but in the next line it says -

Moving back and forth along the line AB, the mass point is continually changing speed vx' Starting from rest at the end points A or B, the speed increases until it reaches C.

Can somebody explain - If the original particle is moving with a constant speed then how come it's mass point have different velocity ? Also for a simple harmonic motion resorting force should be proportional to displacement (towards mean position) .In this diagram i see no force acting on mass point?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
1
$\begingroup$

This isn't the definition of SHM I would go with, but it is equivalent so we'll run with it for now.

The point is that the particle moving around the circle at uniform speed is purely fictitious, the real particle is its 'shadow' moving back and forth along the $x$ axis. If you visualise this for a bit, you should see that when the particle is at the points crossing the $x$-axis its velocity is purely vertical - that means the horizontally projected particle has no velocity. Conversely, at the top and bottom of the circle the reference particle is moving purely horizontally and so the projected particle is moving at its maximal speed.

An equivalent (and I think simpler) way to define harmonic motion is simply any motion that is sinusoidal in time, that is, any motion $x(t)$ in the form:

$$x(t) = A cos(\omega t + \phi)$$

where $A$ is the amplitude (radius of the circle in your diagram) of oscillation, $\omega$ is its 'angular frequency' (named since its the angular frequency of the reference particle) and $\phi$ is just some offset since we don't know where in the cycle the particle started.

Improve this answer
$\endgroup$
4
  • $\begingroup$ are you trying to say that the sum of tangential and centripetal velocity is constant but the individual tangential velocity is constantly changing? $\endgroup$
    – user246374
    Commented Nov 9, 2019 at 19:56
  • $\begingroup$ The velocity is always tangential, but its direction rotates. The projected particle has a velocity that's the horizontal component of the reference particle's velocity. $\endgroup$
    – jacob1729
    Commented Nov 9, 2019 at 20:07
  • $\begingroup$ okay.And what is the cause of force here? or it just a physical representation ? $\endgroup$
    – user246374
    Commented Nov 9, 2019 at 20:12
  • $\begingroup$ This way of viewing things doesn't really emphasise the forces. The particle moving in the circle is a complete fiction - really this is just a way of visualising trigonemetric functions. $\endgroup$
    – jacob1729
    Commented Nov 9, 2019 at 20:15
0
$\begingroup$

SHM is governed by the following equation:- $$\ddot x+\omega^2 x=0$$ Here $\omega$ is a positive constant which is also known as angular frequency of simple harmonic motion and $x$ is the displacement from the mean position.

The diagram given in the post gives an analogue of simple harmonic motion which simplifies the problems we deal with but the above definition clearly convinces us that the net force is always directed towards the equilibrium position and is proportional to its distance from the mean position.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.