0
$\begingroup$

So I am in my course of physical chemistry and in my book there is this image. And quite frankly i am struggling to understand it. I believe i understand the liquid drop model. This is supposed to illustrate the $\alpha$-decay of a nuclid. I don't understand the axes.

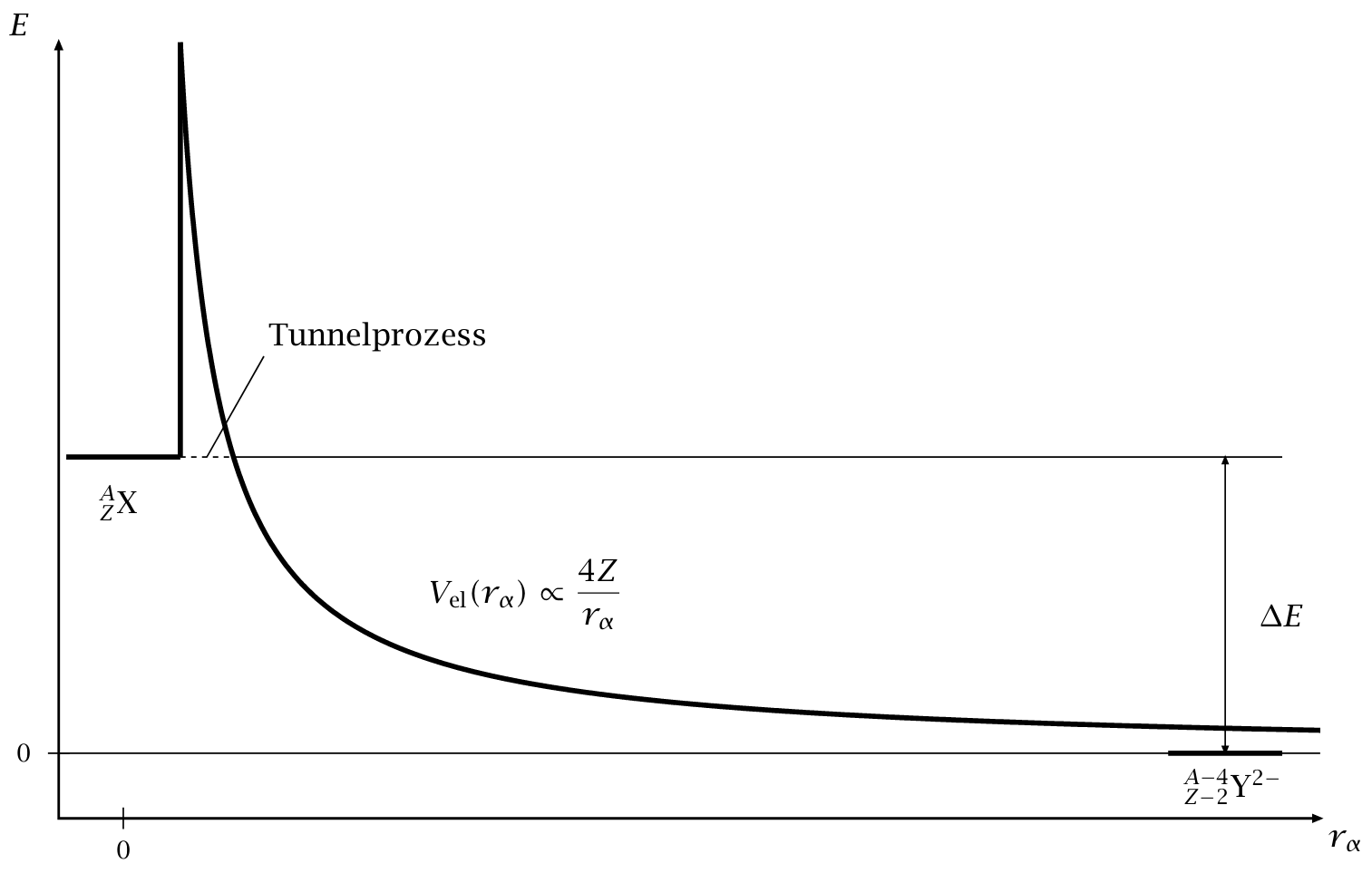
enter image description here

But why is it represented that way $\Delta E$ is the discretely emitted energy. Is $\Delta E$ the difference of the masses times $c^2$ ? Why is $Y$ at some $r_\alpha$ value and "so short". Why do you put the Electric potential of the $\alpha$-particles Kernel there?

Improve this question
$\endgroup$
4
  • $\begingroup$ Understanding the relationship between a diagram like this and a physical process is a multi-faceted thing, so "[I] am struggling to understand" is not a very helpful statement of your problem. Do you understand the meaning of the, axes, and lines in the figure? Do you understand how they related to the players in the dance (the alpha particle and the remnant nucleus? Do you understand the implications of the figure assuming the alpha was a classical particle? Do you understand why the quantum case is different? $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Oct 23, 2019 at 16:52
  • $\begingroup$ @dmckee For me $r_\alpha$ was interpreted as the distance from from the mother nuclid (which doesn't make much sense). $E$ is the total Energy of the atom (which also doesn't make much sense)... $\endgroup$
    – Pastudent
    Commented Oct 23, 2019 at 16:57
  • $\begingroup$ OK. This figure has a detail that I don't like, but I'll discuss that in my answer. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Oct 23, 2019 at 17:05
  • $\begingroup$ This site might help hyperphysics.phy-astr.gsu.edu/hbase/quantum/barr.html $\endgroup$
    – anna v
    Commented Oct 23, 2019 at 17:31

1 Answer 1

Reset to default
4
$\begingroup$

Interpreting the figure requires you to be thinking about the alpha particle as an independent entity moving around in (as part of) or near a nucleus.

The horizontal axis graphs the distance $r_\alpha$ between the particle and the rest of the nucleus (measured to the center).

The vertical axis graphs the energy $E$ "of" the alpha particle.0

The heavy line represents the potental energy of the alpha particle at various distances.1 The potential is made up of the electrostatic potential (with it's $1/r_\alpha$ dependence outside and slower variation inside), plus the effective nuclear force which is a strongly-negative and roughlty-constant value over a limited range but goes to zero quite suddenly. Classically this is the minimum energy the particle can have in any particular place. If the actual energy is higher than this the remainder will be kinetic energy.

By assumption the alpha starts in the nucleus (in the flat region near the horixontal axis) with energy $E_0$ that is lower than the peak value of the potential.2

Classically the alpha could never pass the peak.

But if you take this potential, assume a starting value of the wave-function confined to the nucleus and solve the time-dependant Schroedinger equation, you will find that the particle can escape. The quantum case is different from the classical one.

0 Strictly potential energies should be ascribed to the system as a whole, but we're treating the nucleus as a passive stage on which the alpha dances, so we use the same shortcut used in introductory physics and ascribe the potential to the mobile part of the system.

1 It should represent only the potential. It appears that this author of the figure has varied that meaning a little so that inside the nucleus it represents the ground-state energy (which is more than the pure potential by some amount). I don't like that.

2 In this figure $E_0$ is the upper thin horizontal line. It ought to be higher than the potential in the nucleus, but still lower than the peak. That's why I think the heavy lione is misdrawn.

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.