Surface Tension of Floating Object

Question

I was reading this thesis about surface tension and its role in floating bodies. I couldn't quite understand at page 10 how the author applied the 2D divergence theorem outside the region bounded by the contour. Shouldn't you use the divergence theorem for a region inside the boundary?

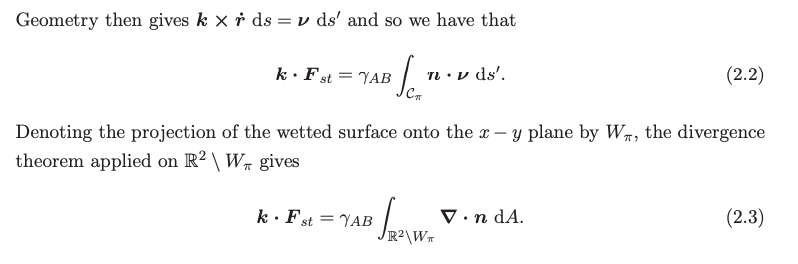
The line integral is evaluated around a boundary C \pi, but the double integral is evaluated outside the region bounded by C\pi

Here is my thinking. The divergence theorem in 2D is:

$\iint_S \nabla \cdot \textbf{F}\hspace{0.2cm} dA = \int_{\partial S} \textbf{F}\cdot d\textbf{n}$

Therefore, if $C_\pi$ the boundary of our region ($\partial S$ in the Divergence theorem), then I would expect the integral:

$\int_{C_\pi} \textbf{n}\cdot d\textbf{n}$

to be equal to:

$\iint_{W_\pi} \nabla \cdot \textbf{n} \hspace{0.2cm} dA$

where $W_\pi$ is the region bounded by $C_\pi$. However, the author sets this equal to:

$\iint_{\mathbb{R}^2 \setminus W_\pi} \nabla \cdot \textbf{n} \hspace{0.2cm} dA$

Then that implies that: $\iint_{\mathbb{R}^2 \setminus W_\pi} \nabla \cdot \textbf{n} \hspace{0.2cm} dA=\iint_{W_\pi} \nabla \cdot \textbf{n} \hspace{0.2cm} dA$

which can't possibly be right. Is there something I am missing?

Answer 1

IMHO your notation is misleading.

What do you mean by $\int_{\partial S} d\mathbf{n}\cdot\mathbf{F}$? I guess this is an integral along the edge of your surface, and then $\mathbf{n}$ is the normal to the surface, point outwards, but then I would say:

$\oint_{\partial S} dl \,\mathbf{\hat{n}}\cdot \mathbf{F}$, i.e. you need to have $dl$ as the small step along your edge. The normalized normal to the edge is here $\mathbf{\hat{n}}$. Moving on to the next integral

$\int_{C_\pi} \mathbf{n}.d\mathbf{n}$

Again, what do you mean? Is the $\mathbf{n}$ the edge normal in both cases? In this case you are basically interested in:

$\oint_{C_\pi} dl\, \mathbf{\hat{n}}.\mathbf{\hat{n}}=\oint_{C_\pi} dl=circumference$

Since $\mathbf{\hat{n}}$ is the normalized normal. By the way, the next integral, $\iint_{W_\pi} dA \,\boldsymbol{\nabla}\cdot\mathbf{n}$ makes no sence at all, in this case. The normal to the edge is only defined on the edge. How do you evaluate it in the bulk?

Perhaps you meant something like:

$\oint_{C_\pi} dl\,\mathbf{\hat{n}}\cdot\mathbf{F}$, for some generic field $\mathbf{F}$

In this case $\oint_{C_\pi} dl\,\mathbf{\hat{n}}\cdot\mathbf{F}=\int_{W_\pi} d^2 r \boldsymbol{\nabla}.\mathbf{F}$

But also, if you invert the boundary normal ($\mathbf{\hat{n}}\to -\mathbf{\hat{n}}$) and assume that $\mathbf{F}$ vanishes at infinity then the edge integral may be taken as the edge integral of $\mathbb{R}^2$ with $W_\pi$ removed:

$\oint_{C_\pi} dl\,\mathbf{\hat{n}}\cdot\mathbf{F}=- \oint_{\partial \left(\mathbb{R}^2\setminus W_\pi\right)} dl\,\mathbf{\hat{n}}\cdot\mathbf{F}=-\int_{\mathbb{R}^2\setminus W_\pi} d^2 r \boldsymbol{\nabla}.\mathbf{F}$

This suggestes that $\int_{\mathbb{R}^2} \boldsymbol{\nabla}.\mathbf{F} = \int_{\mathbb{R}^2\setminus W_\pi} d^2 r \boldsymbol{\nabla}.\mathbf{F} + \int_{W_\pi} d^2 r \boldsymbol{\nabla}.\mathbf{F} = 0$

Which makes sense since (with slight abuse of notation)

$\int_{\mathbb{R}^2} \boldsymbol{\nabla}.\mathbf{F} = \oint_{\partial \mathbb{R}^2} dl\,\mathbf{\hat{n}}.\mathbf{F} $

and we agreed that $\mathbf{F}$ vanishes at infinity.