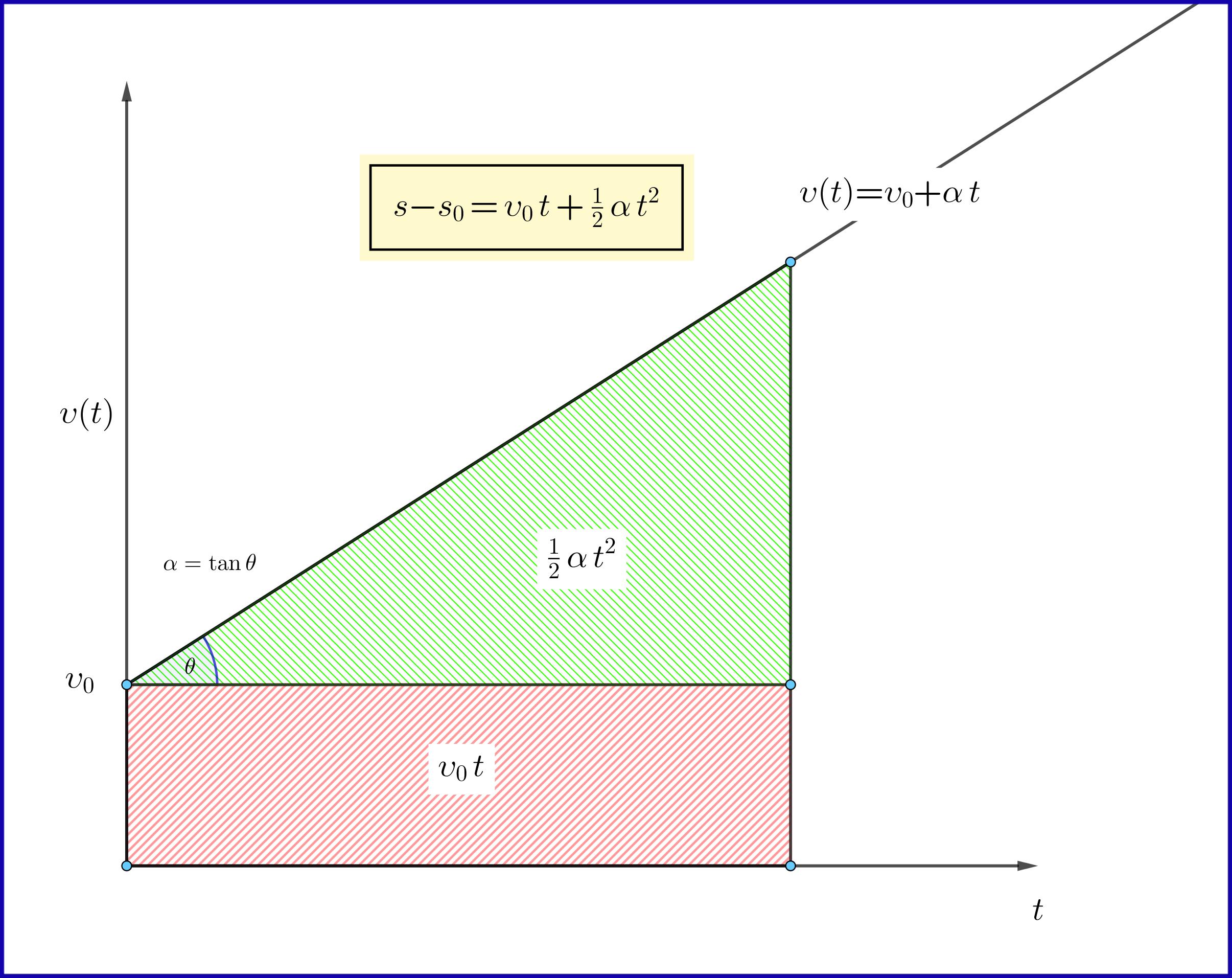
How is this equation derived? $$r = r_0 + ut + at²/2$$ where $r_0$ is the initial position of particle and $r$ is the position of the particle after all the motion it has undergone, $a$ and $t$ have their usual meanings of acceleration and time.
What I think of it is... that this should go on indefinitely like $r = r_0 + ut + at²/2+ jt³/3 +.....$ where j is 4th derivative of displacement. And $jt³/*3*$ because, following the pattern, the denominator is same as the power of time. I'm not sure about. Can you tell me something about it?