For equilibrium of a body on an inclined plane of inclination $45°$. The coefficient of static friction will be greater than one. Why?
My question is why do we use greater than sign instead of equality? The object is in equilibrium, so frictional force must be equal to $mg\sin\theta$?
P.s I am a beginner
The object is in equilibrium, so frictional force must be equal to mgsinø?
$mg$ sin θ is the force down the plane. It equals the maximum static friction force when motion is impending, but it is not the static friction force. The static friction force acts up the plane and is equal to $μmg$ cos θ where $μ$ is the coefficient of static friction. See the free body diagram below.
You can see from the equations on the free body diagram that the coefficient of static friction will always be the tangent of the incline plane angle when there is impending motion (i.e., when the static friction force is at a maximum).
So if the angle is $45^0$, for there to be impending motion, the coefficient of static friction would have to be the tan $45^0$=1. For any angle greater than $45^0$ the static coefficient of friction would have to be greater than 1 to prevent motion.
Hope this helps.
I will make an attempt to explain in my way.
Static friction is self adjusting in nature. This means whenever a force tries to move a body on a roguh surface, friction starts resisting this force as far as it can till the applied force overcomes the maximum static friction possible. Which in other words also means that during the time the applied force increases from zero to the value at which the body just starts moving, coefficient of static friction also increases from zero to the maximum possible value.
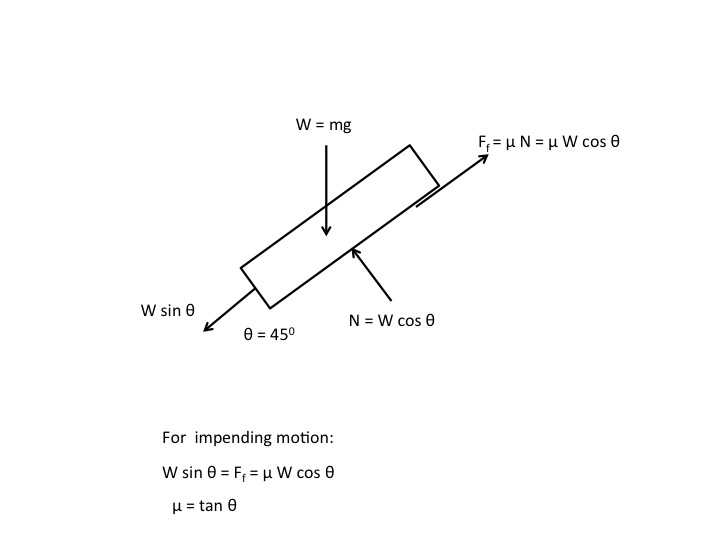
Now, with this concept in mind, just re look at your question. For a body to be in equilibrium on an inlcined plane of 45°, what is the only condition ? The answer is: The force which tries to move the body down the plane should be at all times less than the maximum value of static friction (i.e.the maximum force friction can resist). This mathematically looks like:
mgsinø < μN where μ= Maximum value of static friction.
Now as there is no motion perpendicular to the inclined plane, you can always use
N= mgcosø and substitute in the above equation to get:
mgsinø < μmgcosø i.e. tanø < μ or μ > tanø. Now as ø=45°, you arrive at your answer:
**μ > 1**
To summarize: your starting equation itself is with greater than/less than sign and not equality sign which was your only confusion. The equality sign equation here represents only the case where the applied force is equal to the maximum static friction possible or motion is impending or the body is just about to move which is something you dont want to analyse to answer your question.
