Finding Masses of Binary Star system w/o using Kepler's third law

Question

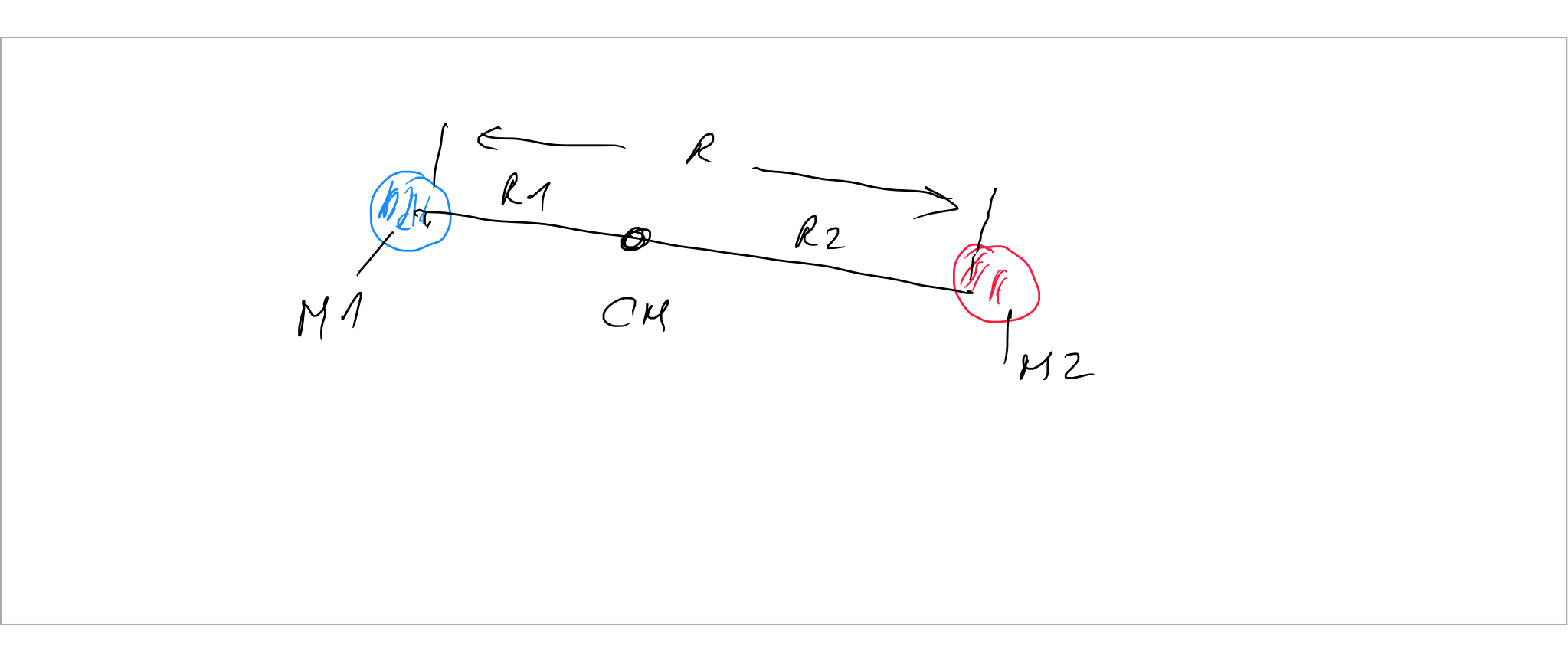
I'm struggling with what feels like it should be a pretty straight-forward intro physics problem, but I'm missing something fundamental.

The scenario is that there is a binary star system with two stars of unknown masses $M_1$ and $M_2$. We do know the radii of the orbits of these two stars. The orbits are both circular.

The problem asks that you draw a sketch of the system labeling the stars and the radii of their orbits. Part two asks to find the centre of mass of the system and to label it on the sketch. This should be straight forward given that $r_{cm} = \frac {(M_1R_1 + M_2R_2)}{M_1 + M_2}$. You end up with the result that $M_1R_1 = M_2R_2$ and that the centre of mass must be at the point of the centre of the observed orbits. Nothing to surprising there.

The next part of the problem tells us that observations of the system reveal an orbital period of 40 years. We know that the stars have the same orbital period.

The question asks for us to determine the masses of the two stars. Using Kepler's third law and the fact that $M_1 = \frac{M_2R_2}{R_1}$ this would be straight-forward. But this is an intro physics class and we're studying energy not orbital dynamics, and so I find myself on the struggle bus.

I've tried relating the total kinetic energy of the system (which we don't know directly) to the rotational energy via $K_{tot} = K_{trans} + K_{rel}$ where I assume $K_{trans} = 0$ (we're not given anything about the proper motion of the system, so I don't have any information about the motion of the centre of mass of the system). The problem with this approach is that you just end up with a trivial result and you can't isolate either $M_1$ or $M_2$ and then solve for it. Driving me mad. I just don't know what concept I'm missing or getting wrong here. Any guidance would be really appreciated. Thanks.

Answer 1

Circular motion makes it easy.

The force between the two masses:

$F=\frac{G\ m1 \ m2}{(R1+R2)^2}$

Knowing the period and the radii, we can get the orbital velocities:

$v1=\frac{2\ \pi\ R1}{T}$

$v2=\frac{2\ \pi\ R2}{T}$

And we have the circular motion relationship between the force and the orbital velocities:

$F=\frac{m1\ v1^2}{R1}$

and

$F=\frac{m2\ v2^2}{R2}$

from which we can get the masses. There is no need for Kepler's law for this method.

Answer 2

Kepler's III Law of planetary motion states that

$\frac{P^2}{R^3} = constant$

where $P$ is the period of the system and $R = R_1+R_2$ is the separation between both objects.

The only thing that Kepler knew was that the square of the orbital period was proportional to the "size" of the orbit, $R$, cubed (here I said size of the orbit because for a planet orbiting the Sun this is almost the same as the Sun-planet separation but in your case consider $R$ to be the separation between the stars). So a change in the size of the orbit corresponded to a larger change in the orbital period.

Kepler didn't knew what that constant was but thanks to Newton's law of universal gravitation now we know that

$constant = \frac{G(M_1+M_2)}{4\pi^2}$

In general the mass of the planets $M_2$ was very small compared to the mass of the sun $M_1$ so Kepler didn't realized that the constant was slightly different for each planet of the Solar System, but as you can see (and NEwton saw) it depends on $M_2$ so it is. Also Kepler only had our Solar System to make observations (no exoplanet was known nor any binary system had ever been observed moving) so he thought that this constant should also be the same for any planetary system by extrapolation. Thanks to Newton we now know that this constant also depends on the mass of the Sun $M_1$ thus it is a unique constant for each two bodies that are gravitationally interacting.

There is a trick to help in the calculation. We can measure $R$ in AU (astornomical units) and $P$ in years, and finally we can say $G = 4\pi^2$ for some specific units of measurement that would allow us to make this. Overall this gets simplier and thus Kepler's III Law can be stated as:

$P^2=constant\cdot R^3 = \frac{R^3}{M_1+M_2}=\frac{(R_1+R_2)^3}{M_1+M_2}$

only if we always stick to consider $P$ to be measured in years and $R_1$, $R_2$ in AU.

Combining this knowledge with your $M_1 = M_2R_2/R_1$ we can obtain

$M_2 = \frac{(R_1+R_2)^3}{P^2(R_2/R_1+1)} = R_1\left(\frac{R_1+R_2}{P}\right)^2$

and

$M_1 = M_2R_2/R_1=R_2\left(\frac{R_1+R_2}{P}\right)^2$

Since you know $P = 40\; years$ and $R_1$, $R_2$ are given, you can get both masses.

---

In my opinion it is unfair if some teacher wants you to derive this if you haven't done Kepler's laws. Also, you not only need Kepler III Law but also Newton's Universal Law of gravity to know the constant of Kepler's III Law (considering it in its original form).

Answer 3

Kepler Third Law

$${\omega}^{2}={\frac {G \left( M_{{1}}+M_{{2}} \right) }{{R}^{3}}}\tag 1$$ Where:

$R$ is the distance between $M_1$ and $M_2$

$\omega$ the angular velocity of $M_1$ and $M_2$ .

with the center of mass equation you get:

$$M_1\,R_1=M_2\,R_2\tag 2$$

$$\omega=\frac{2\pi}{T}\tag 3$$

$T$ is the time that take to rotate $2\,\pi$ radiant. This time is the same for $M_1$ and $M_2$

$$R=R_1+R_2\tag 4$$

.

with equation (2) you get $M_2=M_1\frac{R_1}{R_2}$ and with equation (3) and (4) in equation (1)

$$ 4\,{\frac {{\pi }^{2}}{{T}^{2}}}=G \left( M_{{1}}+{\frac {M_{{1}}R_{{1 }}}{R_{{2}}}} \right) \left( R_{{1}}+R_{{2}} \right) ^{-3} \tag 5$$

solving equation (5) for $M_1$

$$M_1=4\,{\frac {{\pi }^{2} \left( R_{{1}}+R_{{2}} \right) ^{2}R_{{2}}}{{T}^ {2}G}} $$ and

$$M_2=M_1\frac{R_1}{R_2}$$