1
$\begingroup$

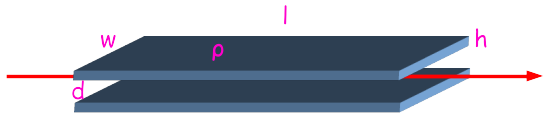
Suppose I have two massive plates of size $l\times h\times w$ mounted parallel to each other with a distance of $d$ and with a mass density of $\rho$. I send a light beam in the middle between them along the length $l$ and in parallel to them.

Light beam between massive parallel plates

How long does it take the light beam, in coordinate time, to pass through the empty space between the plates?

I assume the speed between the plates is basically determined by the gravitational field between the plates and how this then determines the speed of light.

Maybe the better question is: what is the metric tensor between the plates and how does it determine the speed of light?

Given the highly regular setup, I would hope that at least for larger $l$ and $w$ there is a nearly homogeneous (read, constant in a plane or all over) metric tensor "near the middle" of the setup.

Improve this question
$\endgroup$
2
  • $\begingroup$ You might consider the same question for a massive sphere with a small hole drilled through it. Then you could use the Schwarzchild metric to answer it. $\endgroup$
    – mmesser314
    Commented Jun 16, 2019 at 15:09
  • 2
    $\begingroup$ @mmesser314 A good thought and should be doable, but the metric inside a planet is not Schwarzschild. The time dilation at each radius is defined by two factors. One is indeed the Schwarzschild metric of the inner "smaller planet" (discounting the outer shell). The other is the outer shell. For example, the time dilation just outside a thin massive shell is Schwarzschild while the time dilation everywhere inside is the same as just outside. See: arxiv.org/abs/1203.4428 - While gravity at the center is zero, a bit counter intuitively the time dilation there is maximal. $\endgroup$
    – safesphere
    Commented Jun 16, 2019 at 18:45

2 Answers 2

Reset to default
0
$\begingroup$

In Newtonian gravitation there is no field between plates so I would expect no effect. I don't know what a GR solution would be, nor if it's feasible. I know a solution for the case of a single thin plate, but Einstein's equations aren't linear, so I'm afraid it could be of no help for present case.

Improve this answer
$\endgroup$
0
0
$\begingroup$

The speed of light is always the same in vacuum (which I assume is the case for in between the plates here). Even if the plates were enormously massive, as long as the light ray travels in the middle of the gap there would be no bending and in ANY case no change to the speed of light, that is one of the main ingredients of SR and GR.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.